A ball of mass is projected with a velocity at angle with the horizontal. It collides with a smooth box of mass at its highest position. If the co-efficient of restitution is .
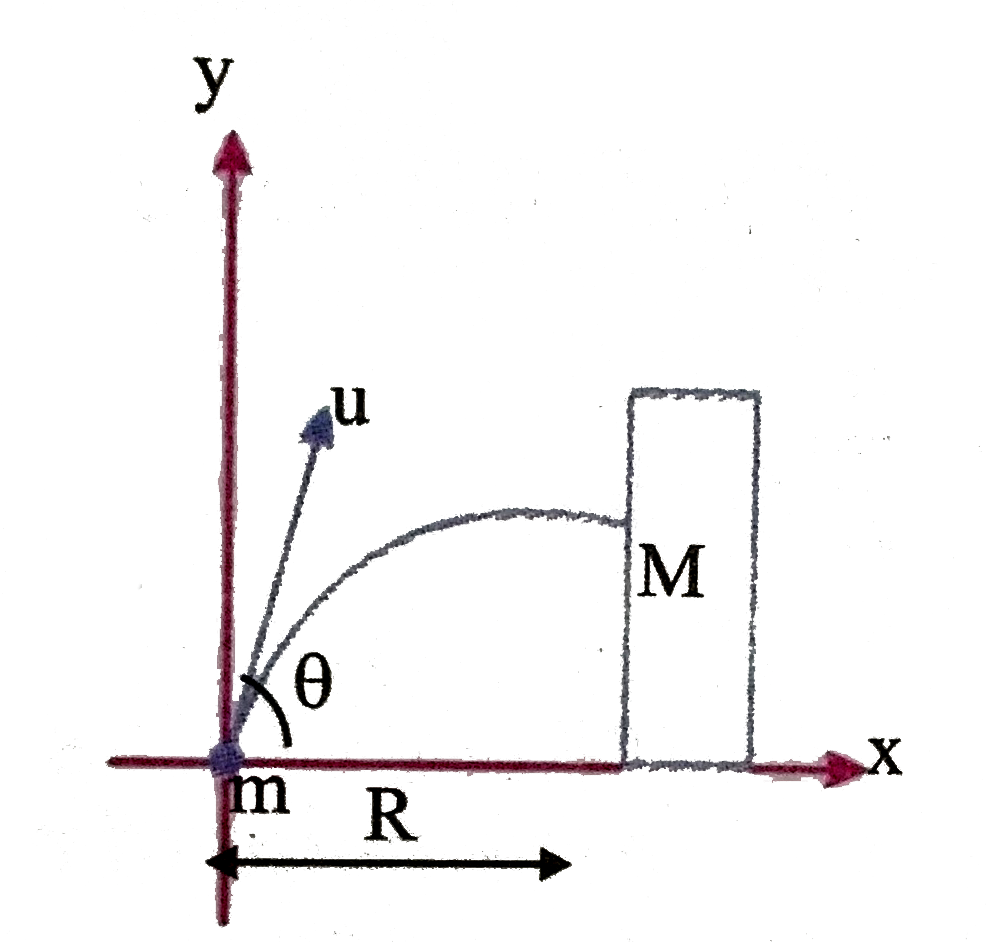
Find the horizontal distance travelled by the ball before collision.
The correct Answer is:B
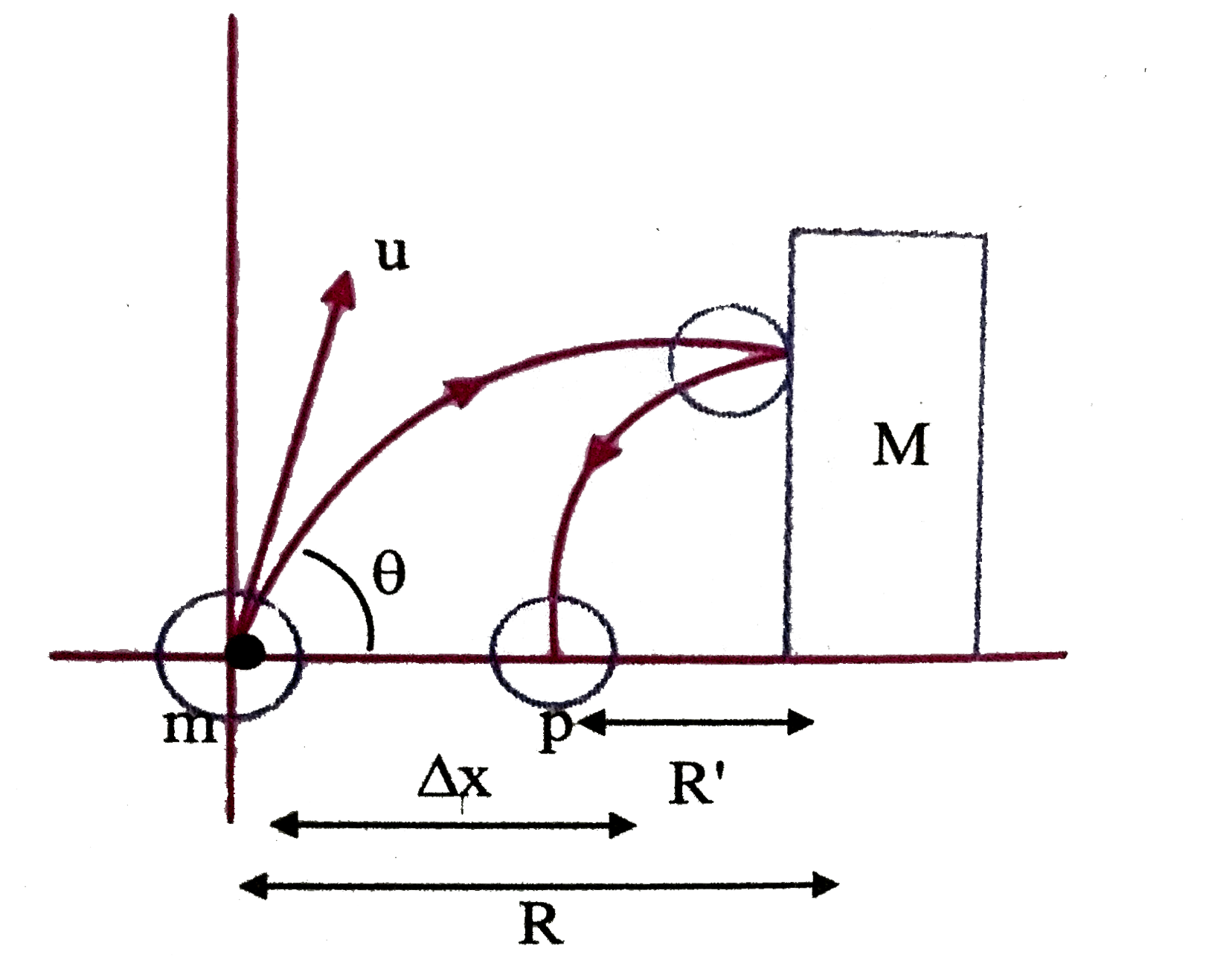
Recoil velocity of ball after collision
From
The ball hit at a point at a distance
Topper's Solved these Questions
- COLLISIONBook:NARAYNAChapter:COLLISIONExercise:Subjective type Questions
Explore 9 Videos
- COLLISIONBook:NARAYNAChapter:COLLISIONExercise:Level-I (H.W)
Explore 52 Videos
- COLLISIONBook:NARAYNAChapter:COLLISIONExercise:Multiple Answer
Explore 11 Videos
- CIRCULAR MOTIONBook:NARAYNAChapter:CIRCULAR MOTIONExercise:LEVEL II(H.W)
Explore 51 Videos
- FRICTIONBook:NARAYNAChapter:FRICTIONExercise:Passage type of questions I
Explore 6 Videos
Similar Questions
A particle of mass m is projected with velocity v at an angle θ with the horizontal. Find its angular momentum about the point of projection when it is at the highest point of its trajectory.
View SolutionA body of mass m is projected with a velocity u at an angle θ with the horizontal. The angular momentum of the body, about the point of projection, when it at highest point on its trajectory is
View Solution
Knowledge Check
A ball of mass m is projected with a velocity 'u' at angle θ with the horizontal. It collides with a smooth box of mass 'M' at its highest position. If the co-efficient of restitution is 'e'.
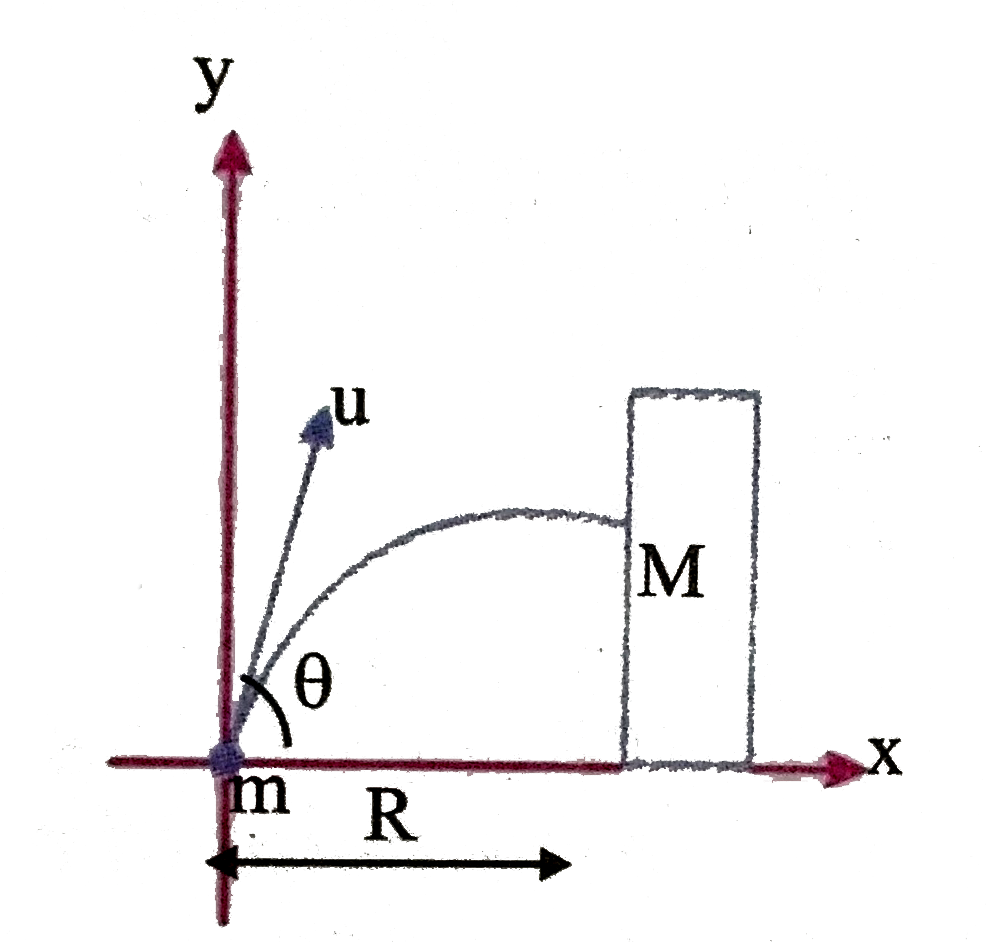
FInd the velocity of the ball after collisionA(m−eMM+m)ucosθB(M−emM+m)ucosθC(m+eMM+m)usinθD(m−eMM+m)usinθA ball of mass m is projected with a velocity 'u' at angle θ with the horizontal. It collides with a smooth box of mass 'M' at its highest position. If the co-efficient of restitution is 'e'.
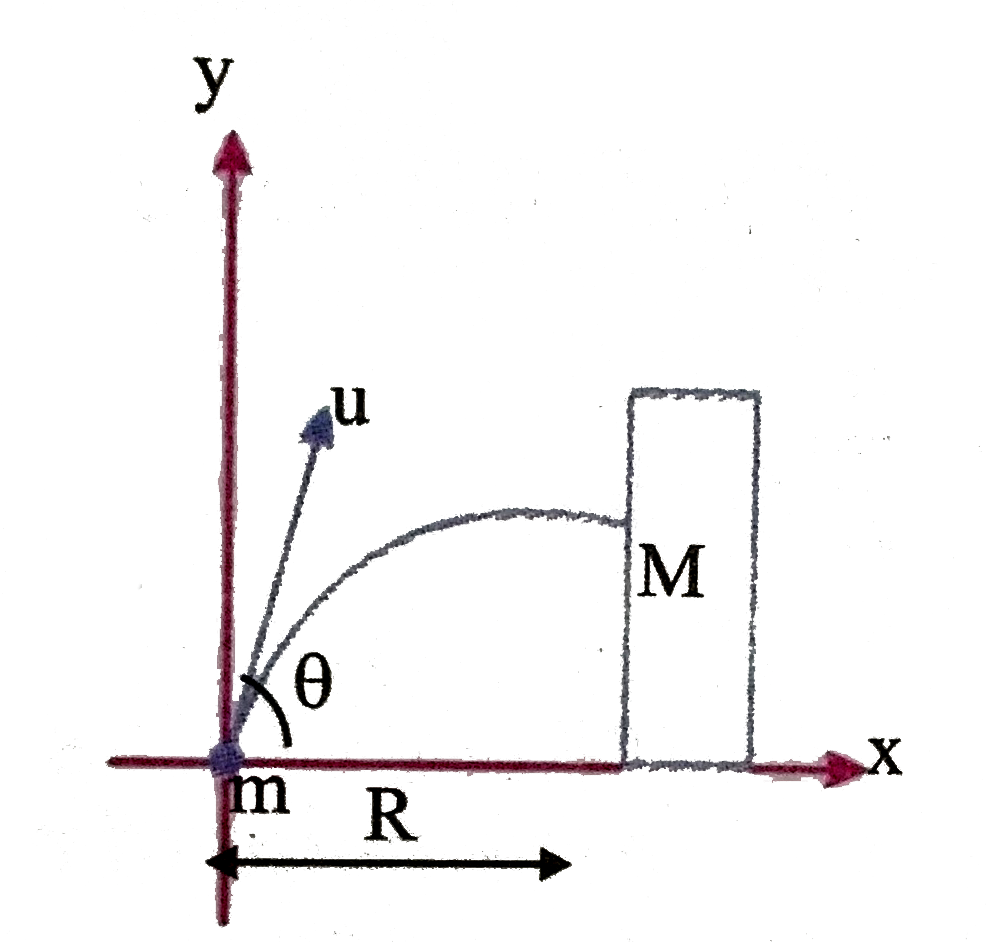
Find the position at which the ball meets the x-axis from the originA(M(1−e)+2mM+m)RB(m(1−e)+2MM+m)RC(M+m(1−e)M+m)RD(M(1−e)+2m2(M+m))RA ball is projected at t=0 with velocity v0 at angle θ with horizontal. It strikes a smooth wall at a distance from it and then falls at a distance d from the wall. Coefficient of restitution is 'e' then :
ABall will return to ground at t=2v0sinθgBd=e(R−L)(whereRisv0sin2θg)Cmaximum height during motion =v20sin2θ2gDit will return to ground at t<2v0sinθg
A ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at a distance a from the point of projection and returns to its original position. Find the coefficient of restitution between the ball and the wall.
View SolutionA ball is dropped on to a horizontal plate from a height h = 9 m above it. If the coefficient of restitution is e = 1/2, the total distance travelled before the ball comes to rest is
View SolutionA particle of mass m is projected with a velocity μ at an angle of θ with horizontal.The angular momentum of the particle about the highest point of its trajectory is equal to :
View SolutionA ball is projected with initial velocity u at an angle θ to the horizontal. Then horizontal displacement covered by ball as it collides third time to the ground would be, if coefficient of restitution is e :

View SolutionA ball is dropped on to a horizontal plate from a height h = 9 m above it. If the coefficient of restitution is e=1/2, the total distance travelled before the ball comes to rest is
View Solution