An ice cube of mass M0 is given a velocity v0 on a round horizontal surface with coefficient of friction μ. The block is at its melting point and latent heat of fusion of ice is L . The block receive heat only due to the friction forces and all work is converted into heat. Find the mass of the remaining ice block after time t.
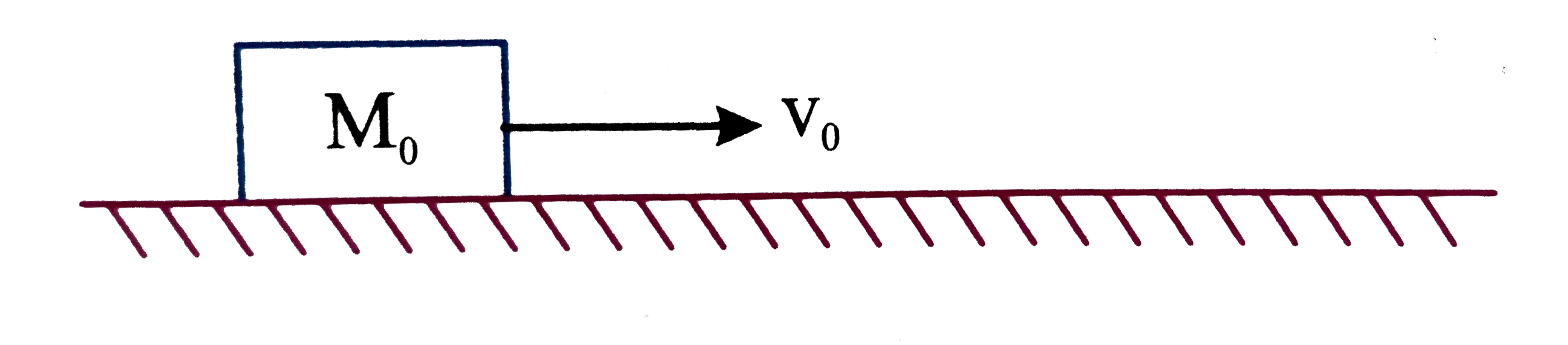
m=m0e−2μgL(V0t+12μgt2)
m=m0e−2μL(V0t−12μgt2)
m=m0e−3μL(V0t−12μgt2)
m=m0e−2μL(V0t−μgt2)
The correct Answer is:B
μmgv=−(dmdt)L
⇒m∫m0dumm=−μgLt∫0(μgt)dt
⇒m=m0e−μgL(v0t−12μgt2)
Topper's Solved these Questions
Similar Questions
A block of mass m moving with a velocity v0 on a rough horizontal surface from x=0 coefficient of friction is given by μ=μ0=ax. Find the kinetic energy of the block as function of x before it comes to rest.
View SolutionA block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View Solution
Knowledge Check
A block of mass m is given an initial downward velocity v0 and left on inclined place ( coefficient of friction =0.6). The block will
 Acontinue of move down the plane with constant velocity v0Baccelerate downwardCdecelerate and come to restDfirst accelerate downward then decelerate
Acontinue of move down the plane with constant velocity v0Baccelerate downwardCdecelerate and come to restDfirst accelerate downward then decelerateA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
A0.5m/s2B5m/s2C10m/s2D15m/s2A block of mass m is lying on horizontal surface of coefficient of friction μ. A force is applied to the block at an angle θ with the horizontal. The block will move with a minimum force F if
Aμ=tanθBμ=cosθCμ=sinθDμ=cotθ
A block of mass M is using on a rough horizontal surface. μR is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View SolutionA block of mass m is placed at rest on an inclination θ to the horizontal.If the coefficient of friction between the block and the plane is μ, then the total force the inclined plane exerts on the block is
View SolutionA marble block of mass 2 kg lying on ice when given a velocity of 6m/s is stopped by friction in 10s. Then the coefficient of friction is
View SolutionA block is kept on a rough horizontal surface as shown. Its mass is 2 kg and coefficient of friction between block and surface (μ)=0.5. A horizontal force F is acting on the block. When

View SolutionA block of mass m is placed on a rough horizontal surface. If minimum force to pull the block is mg2 then coefficient of friction is :
View Solution
