
Consider a disk of mass m, radius R lying on a liquid layer of thickness T and coefficient of viscosity η as shown in the fig.
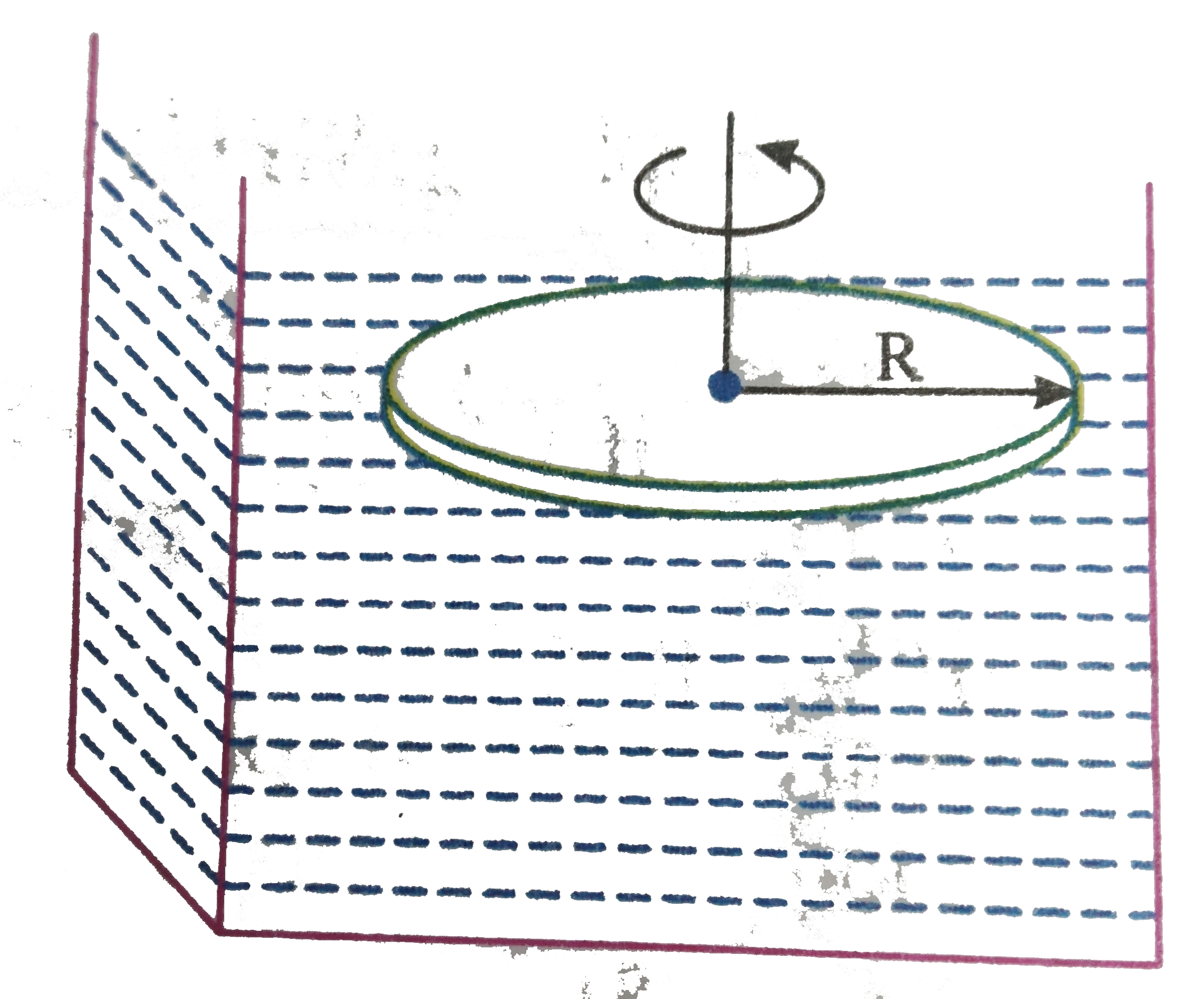
The torque required to rotate the disk at a constant angular velocity Ω given the viscosity is uniformly η.
4πωηR4T
πωηR42T
2πωηR4T
16πωηR4
The correct Answer is:B
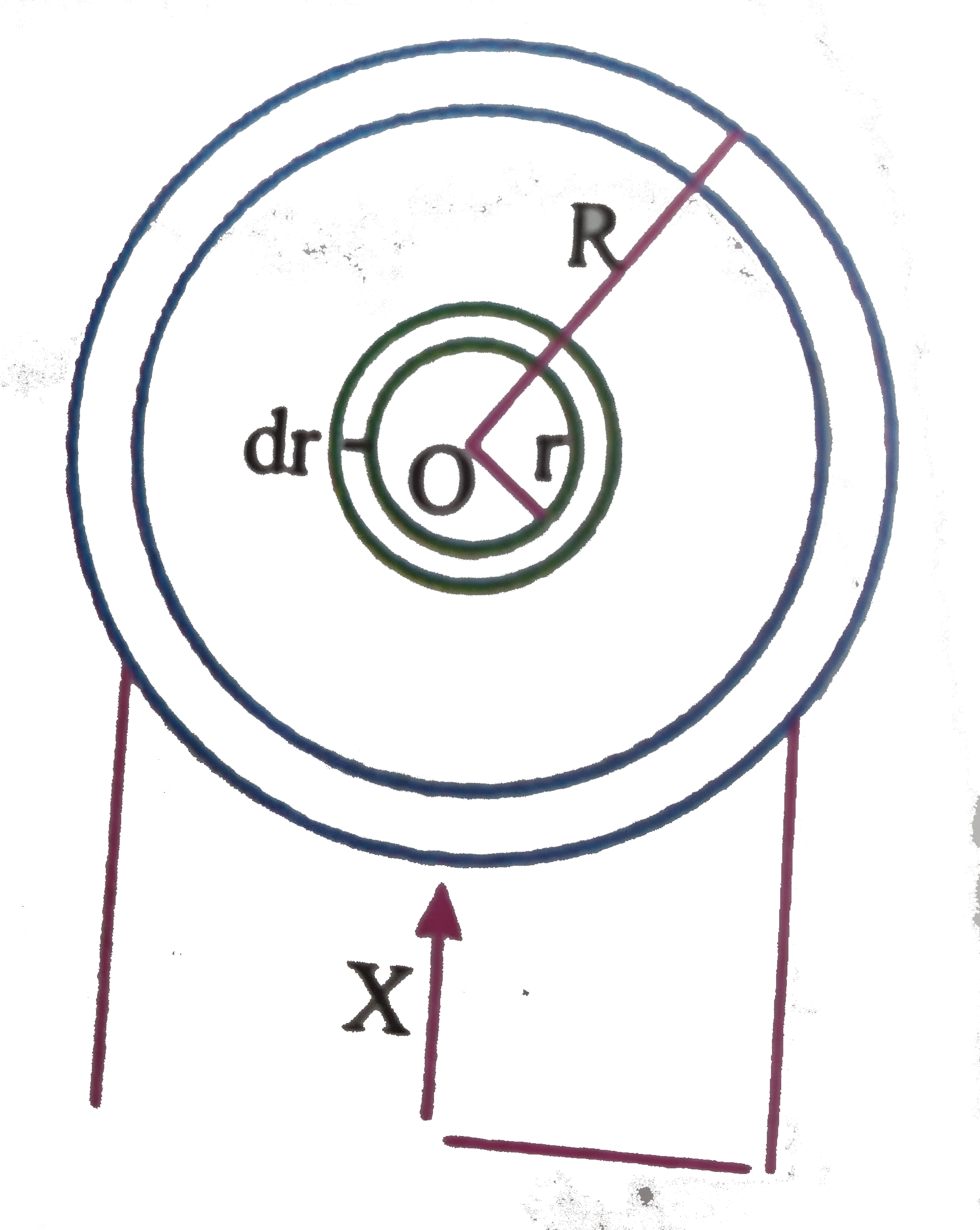
dF=η(dA)dvdx=η(2πr)dr.rωT on integrating
∫τ0dtau=∫R0.r=∫R0η2πr3ωdrT,τ=πωηR42T
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Single Answer Questions
108 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - integer Type Questions
25 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Comprehension Type Questions
40 Videos
- MATHEMATICAL REVIEW & PHYSICAL WORLDBOOK - NARAYNACHAPTER - MATHEMATICAL REVIEW & PHYSICAL WORLDEXERCISE - C.U.Q
13 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NARAYNACHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LEVEL-II (H.W)
24 Videos
Similar Questions
A metal plate of area 10−3m2 is placed on a liquid layer of thickness 0.8mm . The coefficient of viscosity of that liquid is 24poise.Then the horizontal force required to move the plate with a velocity of 1cms−1.
View SolutionSelect ALL correct answer :
A disc of radius R is placed over a horizontal table in such a way that plane of the disc is horizontal and rotated about its vertical axis passing through its center with angular velocity ω. A layer of grease of thickness l is present between the table and the disc.Coefficient of viscosity of grease is η .Then,
(A) torque needed to rotate the disc with constant angular velocity is πηωR44l
(B) torque needed to rotate the disc with constant angular velocity is πηωR42l
(C) power needed to rotate the disc with constant angular velocity is πηω2R42l
(D) power needed to rotate the disc with constant angular velocity is πnω2R44l
View Solution
Knowledge Check
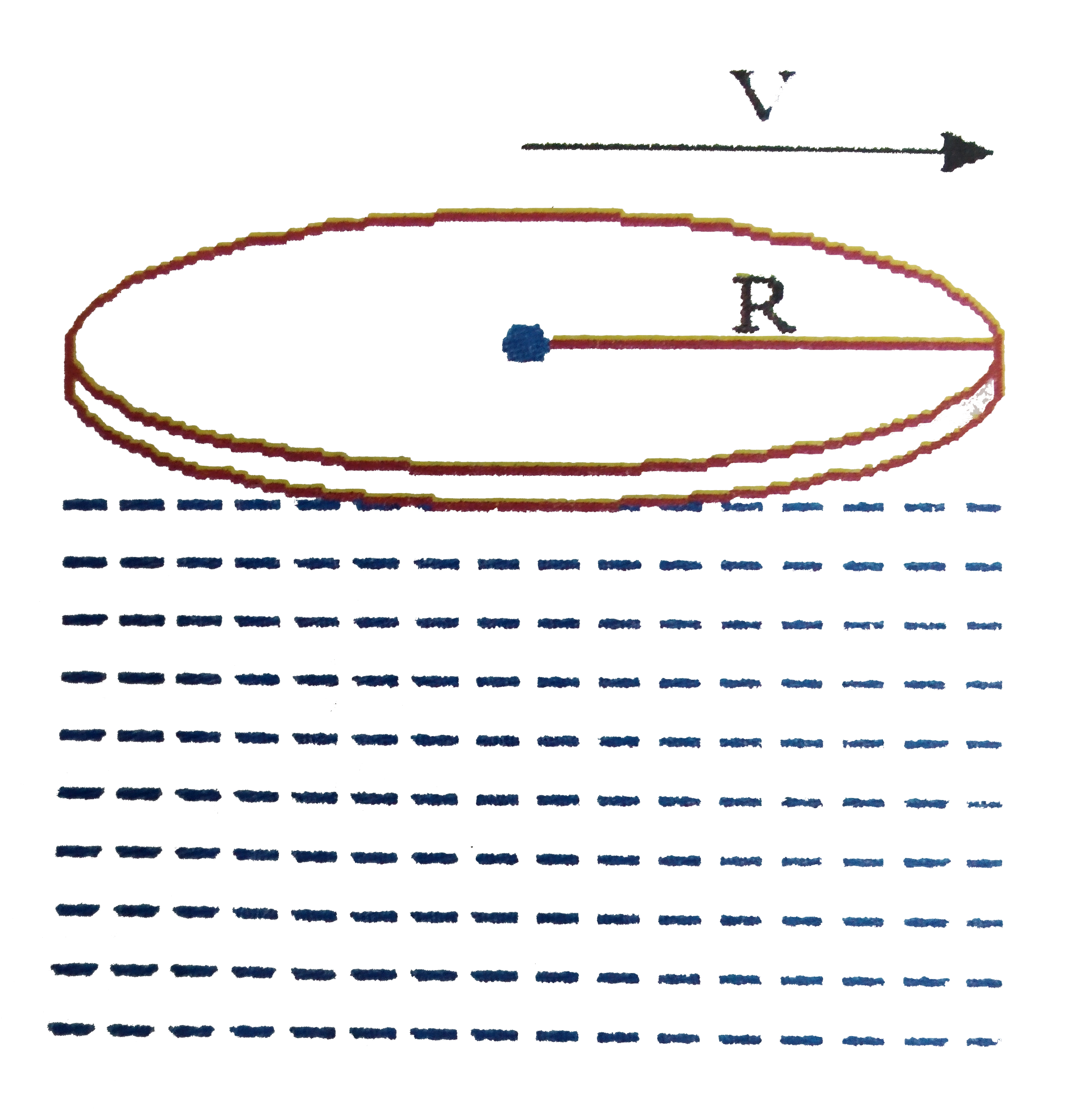
Consider a disk of mass m, radius R lying on a liquid layer of thickness T and coefficient of viscosity η as shown in the fig.
The coefficient of viscosity varies as η=η0x (x measured from centre of the disk) at the given instant the disk is floating towards right with a velocity v as shown, find the force required to move the disk slowly at the given instant.A2η0R2vTB8η0R2vTCπη0R2vTD16η0R3vT
Consider a disk of mass m, radius R lying on a liquid layer of thickness T and coefficient of viscosity η as shown in the fig.
A disc rotating with angularvelocity ω is placed on a viscous liquid of thickness T. Find the angle rotated by the disc before it comes to rest. (viscosity=η, mass of disc =M, radius of disc =R)A4ω0TMηπR2B2ω0TMηπR2Cω0TMηπR2Dω0TM2ηπR2A metal plate of area 10−2m2 is placed on a liquid layer of thickness 2×10−3m. If the liquid has coefficient of viscosity 2 S.I. units the force required to move the plate with a velocity of 3cms is
A0.3 NB0.03 NC3 ND30 N
A ring of mass m and radius R is being rotated about its axis with constant angular velocity ω in the gravity free space. Find tension in the ring.
View SolutionA man is rowing a boat with a constant velocity v0 in a river. The contact area of boat is 'A' and coefficient of viscosity is η. The depth of river is 'D' . Find the force required to row the boat.
View SolutionA drop of water of radius r is falling rhough the air of coefficient of viscosity η with a constant velocity of v the resultant force on the drop is
View SolutionA ring of radius 'r' and mass per unit length 'm' rotates with an angular velocity 'ω' in free space then :
View SolutionA small steel ball of mass m and radius r is falling under gravity through a viscous liquid of coefficient of viscosity η. If g is the value of acceleration due to gravity. Then the terminal velocity of the ball is proportional to (ignore buoyancy)
View Solution