A swing moving in a children's garden is observed to move with an angular velocity given by, ω−a(t2)ˆi+b(e−1)ˆj. What will be the angle between angular acceleration and angular velocity at t=1 s given that a=b=1 unit?
20∘
36∘
15∘
9∘
The correct Answer is:A
Step by step video, text & image solution for A swing moving in a children's garden is observed to move with an angular velocity given by, omega-a(t^2)hat(i)+b(e^-1)hat(j). What will be the angle between angular acceleration and angular velocity at t=1 s given that a=b=1 unit? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - TARGET PUBLICATIONCHAPTER - CIRCULAR MOTIONEXERCISE - COMPETITIVE THINKING
102 Videos
- ATOMS, MOLECULES AND NUCLEI BOOK - TARGET PUBLICATIONCHAPTER - ATOMS, MOLECULES AND NUCLEI EXERCISE - Evaluation test
19 Videos
- CURRENT ELECTRICITY BOOK - TARGET PUBLICATIONCHAPTER - CURRENT ELECTRICITY EXERCISE - Evaluation Test
6 Videos
Similar Questions
Knowledge Check
The angular velocity of a particle is given by the equation ω=2t2+5rads−1. The instantaneous angular acceleration at t=4 s is
A16rads−2B19rads−2C10rads−2D12rads−2A particle moving along a circule path of radius 'r' with uniform angular velocity ω. Its angular acceleration is
Arω2Br/ωCzeroDω/rIf angular velocity of a disc depends an angle rotated θ as ω=θ2+2θ, then its angular acceleration α at θ=1 rad is :
A8rad/s2B10rad/s2C12rad/s2DNone of these
A solid body rotates with angular velocity →ω=3tˆi+2t2ˆjrad/s. Find
(a) the magnitude of angular velocity and angular acceleration at time t=1s and
(b) the angle between the vectors of the angular velocity and the angular acceleration at that moment.
View SolutionThe rod of length l=1m rotates with an angular velocity ω=2rads−1 an the point P moves with velocity v=1ms−1 and acceleration a=1ms−2. Find the velocity and acceleration of Q.
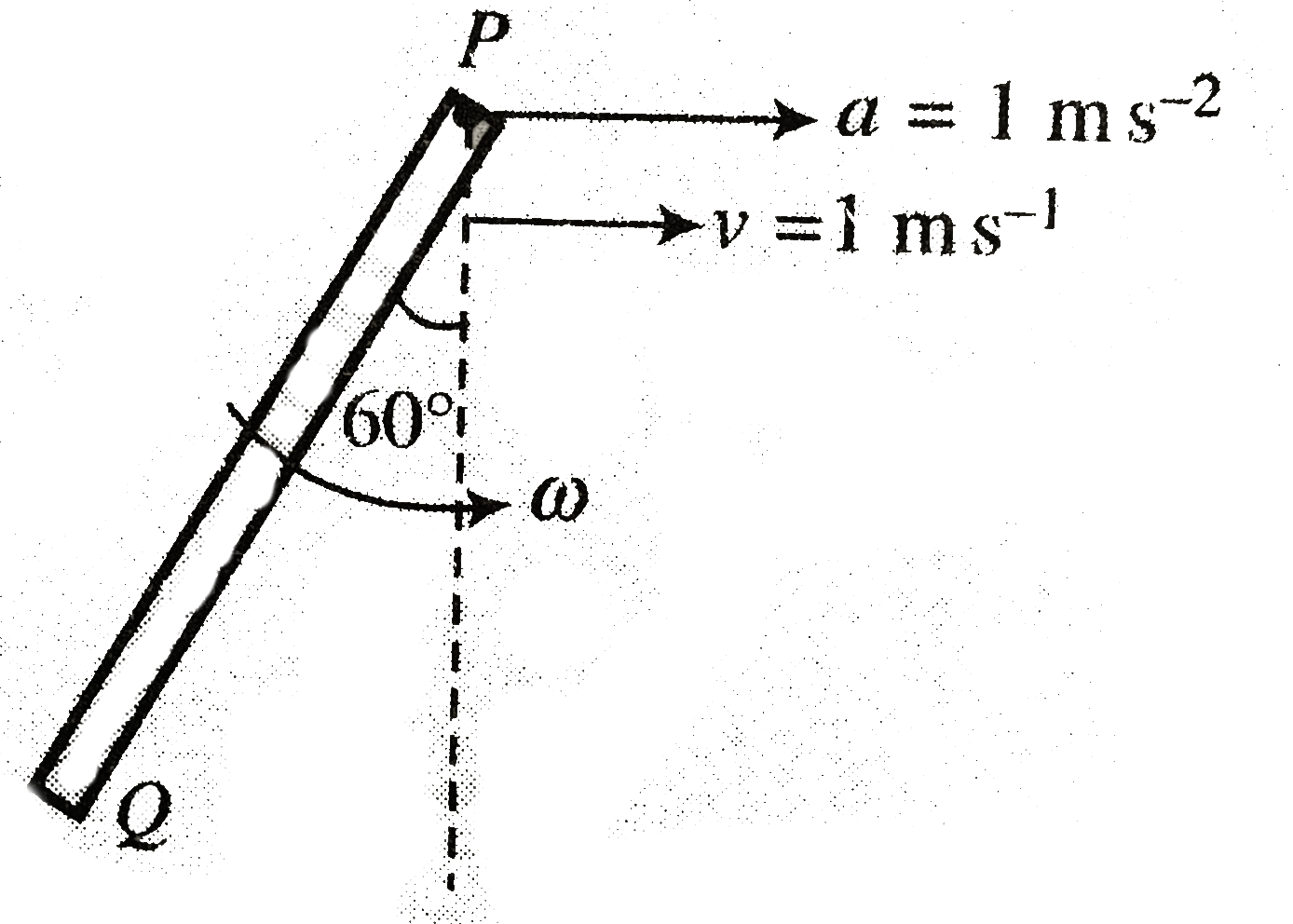
View SolutionThe angular position of a swinging door is described by θ=5+10t+20t2. Determine the angular position, angular speed and angular acceleration at t=2s.
View SolutionThe angular position of a point on the rim of a rotating wheel is given by θ=4t3−2t2+5t+3 rad. Find
(a) the angular velocity at t=1s,
(b) the angular acceleration at t=2s.
(c ) the average angular velocity in time interval t=0 to t=2s and
(d) the average angular acceleration in time interval t=1 to t=3s.
View Solutionthe angular velocity omega of a particle varies with time t as ω=5t2+25rads. the angular acceleration of the particle att=1s is
View Solution
