A particle of mass m1 experienced a perfectly elastic collision with a stationary particle of mass m2. What fraction of the kinetic energy does the striking particle lose, if
(a) it recoils at right angles to its original motion direction,
(b) the collision is a head-on one?
To solve the problem, we need to analyze the two scenarios of the elastic collision involving a particle of mass m1 colliding with a stationary particle of mass m2.
Part (a): Recoil at Right Angles
1. Initial Kinetic Energy Calculation:
The initial kinetic energy KEi of the particle m1 is given by:
KEi=12m1u21
where u1 is the initial velocity of m1.
2. Momentum Conservation:
Since the collision is elastic and m2 is stationary, we apply conservation of momentum in both the x and y directions.
- In the x-direction:
m1u1=m2v2cos(θ)+m1v1cos(90∘)⟹m1u1=m2v2cos(θ)(1)
- In the y-direction:
0=m1v1+m2v2sin(θ)⟹m1v1=−m2v2sin(θ)(2)
3. Energy Conservation:
The total kinetic energy before and after the collision must be equal:
12m1u21=12m1v21+12m2v22
Rearranging gives:
m1u21=m1v21+m2v22(3)
4. Using Equations (1) and (2):
From equation (1), we can express v2 in terms of u1, v1, and the masses:
v2=m1u1m2cos(θ)(4)
Substitute v2 from equation (4) into equation (3) to find v1.
5. Fraction of Kinetic Energy Lost:
The fraction of kinetic energy lost is given by:
Fraction lost=KEi−KEfKEi=1−KEfKEi
After substituting the expressions for KEf and simplifying, we find:
Fraction lost=2m1m1+m2
Part (b): Head-On Collision
1. Initial Kinetic Energy Calculation:
The initial kinetic energy remains the same:
KEi=12m1u21
2. Momentum Conservation:
For a head-on collision, the momentum conservation equation is:
m1u1=m1v1+m2v2(5)
3. Energy Conservation:
The energy conservation equation is:
12m1u21=12m1v21+12m2v22
4. Solving for Velocities:
From equation (5), we can express v2 in terms of u1 and v1:
v2=m1(u1−v1)m2(6)
Substitute v2 into the energy conservation equation to find v1.
5. Fraction of Kinetic Energy Lost:
The fraction of kinetic energy lost is again given by:
Fraction lost=1−KEfKEi
After substituting the expressions for KEf and simplifying, we find:
Fraction lost=4m1m2(m1+m2)2
Summary of Results
- Part (a): The fraction of kinetic energy lost when recoiling at right angles is 2m1m1+m2.
- Part (b): The fraction of kinetic energy lost in a head-on collision is 4m1m2(m1+m2)2.
The initial kinetic energy KEi of the particle m1 is given by:
KEi=12m1u21
where u1 is the initial velocity of m1.
Since the collision is elastic and m2 is stationary, we apply conservation of momentum in both the x and y directions.
- In the x-direction:
m1u1=m2v2cos(θ)+m1v1cos(90∘)⟹m1u1=m2v2cos(θ)(1)
- In the y-direction:
0=m1v1+m2v2sin(θ)⟹m1v1=−m2v2sin(θ)(2)
The total kinetic energy before and after the collision must be equal:
12m1u21=12m1v21+12m2v22
Rearranging gives:
m1u21=m1v21+m2v22(3)
From equation (1), we can express v2 in terms of u1, v1, and the masses:
v2=m1u1m2cos(θ)(4)
Substitute v2 from equation (4) into equation (3) to find v1.
The fraction of kinetic energy lost is given by:
Fraction lost=KEi−KEfKEi=1−KEfKEi
After substituting the expressions for KEf and simplifying, we find:
Fraction lost=2m1m1+m2
The initial kinetic energy remains the same:
KEi=12m1u21
For a head-on collision, the momentum conservation equation is:
m1u1=m1v1+m2v2(5)
The energy conservation equation is:
12m1u21=12m1v21+12m2v22
From equation (5), we can express v2 in terms of u1 and v1:
v2=m1(u1−v1)m2(6)
Substitute v2 into the energy conservation equation to find v1.
The fraction of kinetic energy lost is again given by:
Fraction lost=1−KEfKEi
After substituting the expressions for KEf and simplifying, we find:
Fraction lost=4m1m2(m1+m2)2
- Part (a): The fraction of kinetic energy lost when recoiling at right angles is 2m1m1+m2.
- Part (b): The fraction of kinetic energy lost in a head-on collision is 4m1m2(m1+m2)2.
Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Universal Gravitation
34 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Dynamics Of A Solid Body
56 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - The Fundamental Equation Of Dynamics
59 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions
A particle of mass m experienced an elastic collision with a stationary particle of mass 2m . What fraction of the kinetic energy does the striking particle lose if recoils at the right angles to its original motion direction.
View SolutionA particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entires in column I to all the entires in column II.
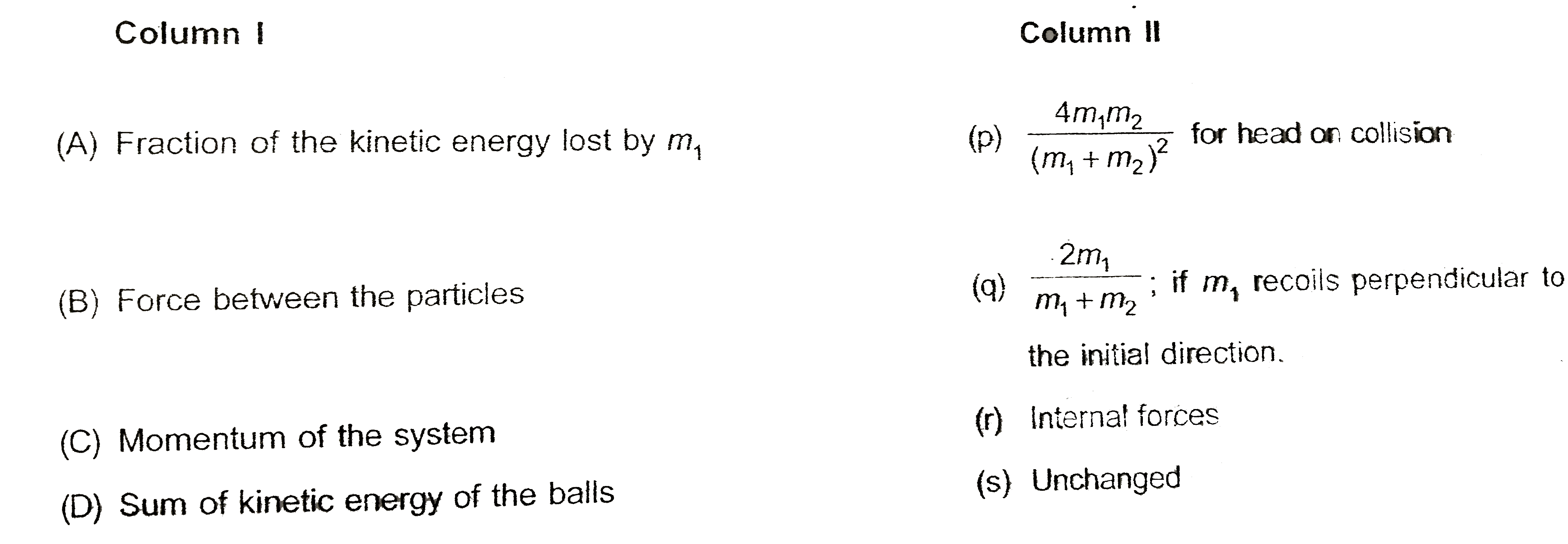
View Solution
Knowledge Check
A particle of mass m1 makes an elastic, one-dimensional collision with a stationary particle of mass m2.What fraction of the kinetic energy of m1 is carried away by m2?
Am1m2Bm2m1C2m1m2(m1+m2)2D4m1m2(m1+m2)2A particle of mass m1 makes an elastic one dimensional collision with a stationary particle of mass m2.What fraction of the kinetic energy of m1 is carried away by m2?
Am1m2Bm2m1C2m1m2(m1+m2)2D4m1m2(m1+m2)2A particle (A) of mass m1 elastically collides with another stationary particle (B) of mass m2. Then :
Am1m2=12 and the particles fly a part in the opposite direction with equal velocities.Bm1m2=13 and the particles fly apart in the opposite direction with equal velocities.Cm1m2=21 and the collision angle between the particles is 60∘ symmetrically.Dm1m2=21 and the particles fly apart symmetrically at an angle 90∘
Particle 1 experiences a perfectly collision with a staionary particle 2. Determine their mass ratio , if the particles move perpendicularly to each other.
View SolutionA particle of mass m1 collides elastically with a stationary particle of mass m2(m1>m2). Find the maximum angle through which the striking particle may deviate as a result of the collision.
View SolutionA particle of mass m moving with speed u collides perfectly inelastically with another particle of mass 2m at rest. Find loss of kinetic energy of system in the collision.
View SolutionA ball of mass m1 makes an elastic , one-dimensional collision with a stationary particle of mass m2. The fraction of the kinetic energy of m1 transferred to m2 is
View SolutionA particle of mass m moving with velocity v makes head-on elastic collision with a sationary particle of mass 2m. Kinetic energy lost by the lighter particle during period of deformation is
View Solution
