A particle of mass 1.0g moving with velocity v1=3.0i−2.0j experiences a perfectly inelastic collision with another particle of mass 2.0g and velocity v2=4.0j−6.0k. Find the velocity of the formed particle (both the vector v and its modulus), if the components of the vectors v1 and v2 are given in the SI units.
From conservation of momentum, for the closed system "both colliding particles"
m1→v1+m2→v2=(m1+m2)→v
or, →v=m1→v1+m2→v2m1+m2=1(3→i−2→j)+2(4→j−6→k)3=→i+2→j−4→k
Hence |→v|=√1+4+16m/s=4⋅6m/s
Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Universal Gravitation
34 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Dynamics Of A Solid Body
56 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - The Fundamental Equation Of Dynamics
59 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions
A particle of mass m1 moves with velocity v1 and collides with another particle at rest of equal mass. The velocity of the second particle after the elastic collision is
View SolutionA particle of mass m moving with velocity 2v collides with another particle of mass 3m moving with velocity v in the same direction.If it is perfect inelastic collision,the loss of kinetic energy of the system is
View Solution
Knowledge Check
A particle of mass 1 g moving with a velocity →v1=3ˆi−2ˆj ms−1 experiences a perfectly in elastic collision with another particle of mass 2 g and velocity →v2=4ˆj−6ˆk ms−1. The velocity of the particle is
A2.3ms−1B4.6ms−1C9.2ms−1D6ms−1A particle of mass 1.0 g moving with a velocity →v1=(3ˆi−2ˆj) m/s experiences a perfectly inelastic collision with another particle of mass 2.0 g and velocity →v2=(4ˆi−6ˆj) m/s. Find the magnitude of the velocity vector →v of the coalesced particles.
A2.3 m/sB5.93 m/sC3.2 m/sD6.4 m/sA ball of mass M moving with a velocity v collides perfectly inelastically with another ball of same mass but moving with a velocity v in the opposite direction. After collision
Aboth the balls come to restBthe velocities are exchanged between the two ballsCboth of them move at right angles to the original line of motionDone ball comes to rest and another ball travels back with velocity 2v
A particle of mass 0.1kg moving with an initial speed v collides with another particle of same mass kept at rest. If after collision total energy becomes 0.2J . Then
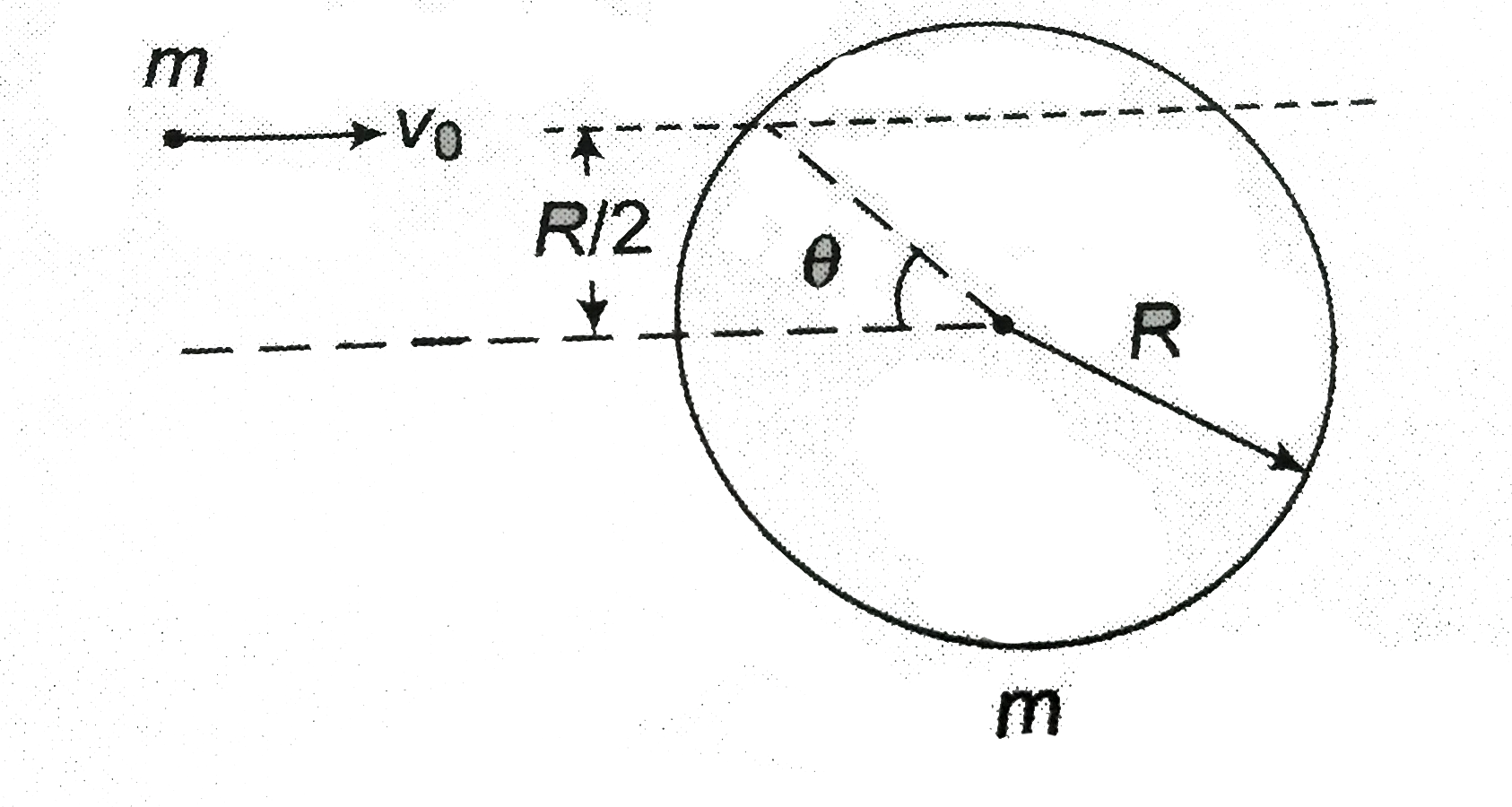
View SolutionA particle of mass m moving with velocity v0 collides with sphere of same mass at rest , as shown. If the surface of contact is smooth and the collision is elastic, find the velocities of particle and sphere after the collision.
View SolutionA charge particle of mass m and charge q is projected with velocity v along y-axis at t=0.

Find the velocity vector and position vector of the particle →v(t)and→r(t) in relation with time.
View SolutionIf the material particle is moving with velocity v and the velocity of light is c, then mass of particle is taken as (m0 is rest mass)
View SolutionA body starts moving with a velocity v0=10ms−1. It experiences a retardation equal to 0.2v2. Its velocity after 2s is given by
View Solution
