A shell acquires the initial velocity v=320m/s, having made n=2.0 turns inside the barrel whose length is equal to l=2.0m. Assuming that the shell moves inside the barrel with a uniform acceleration, find the angular velocity of its axial rotation at the moment when the shell escapes the barrel.
The shell acquires a constant angular acceleration at the same time as it accelerates linearly. The two are related by (assuming both are constant)
wl=β2πn
Where w= linear acceleration and β= angular acceleration
Then, ω=√2⋅β2πn=√2⋅wl(2πn)2
But v2=2wl, hence finally
ω=2πnvl
Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - The Fundamental Equation Of Dynamics
59 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Laws Of Conservation Of Energy, Momentum And Angular Momentum
82 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions
A train starting from rest moves with a uniform acceleration of 0.2m/s2 for 5 minutes . Calculate the speed acquired and the distance travelled in this time.
View SolutionA uniform rod of mass M & length L is hinged about its one end as shown. Initially it is held vartical and then allowed to rotate, the angular velocity of rod when it makes an angle of 37∘ with the vertical is
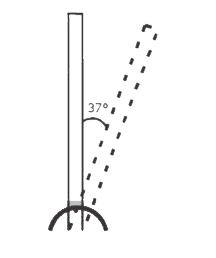
View Solution
Knowledge Check
A particle is given an initial speed u inside a smooth spherical shell of radius R=1 m such that it is just able to complete the circle. Acceleration of the particle when its velocity is vertical is
 Ag√10BgCg√2D3g
Ag√10BgCg√2D3gA rod of length l(<2R0 is kept inside a smooth sperical shell as shwon in fifure .mass of the rod is m
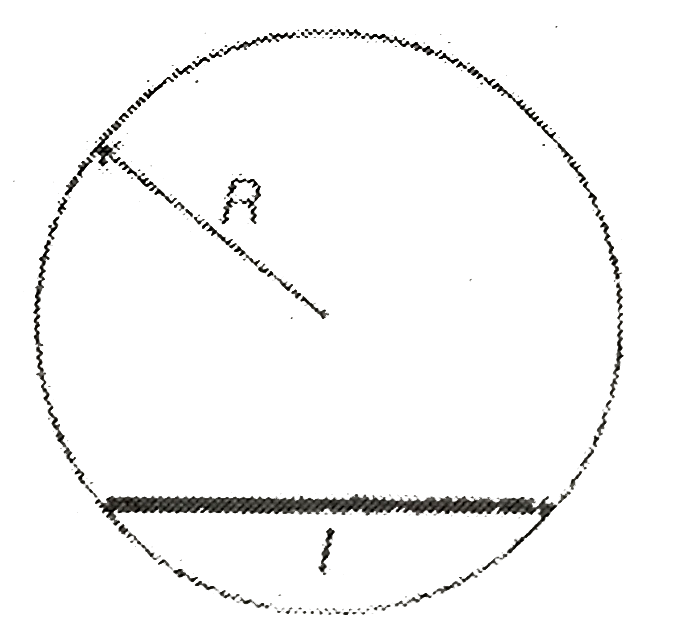
The normal reaction when l=R isAmg2Bmg4Cmg2√3Dmg√3
A solid body rotates with angular velocity ω=ati+bt2j, where a=0.50rad/s2, b=0.060rad/s3, and i and j are the unit vectors of the x and y axes. Find:
(a) the moduli of the angular velocity and the angular acceleration at the moment t=10.0s,
(b) the angle between the vectors of the angular velocity and the angular acceleration at that moment.
View SolutionA shell leaves the barrel of a gun at a speed of 800 m/s. The barrel is 2.0 m long. Find the average acceleration.
View SolutionA gun (of mass M ) whose barrel makes an angle α with the horizontal fires a shell (of mass m ) . The gun is mounted on a frictionless track , so that recoil takes place with no resistive forces . Find the recoil velocity V of the gun and the absolute velocity of the shell in magnitude and direction if the muzzle velocity of the shell relative to the barrel is v .
View SolutionThe rod of length l=1m rotates with an angular velocity ω=2rads−1 an the point P moves with velocity v=1ms−1 and acceleration a=1ms−2. Find the velocity and acceleration of Q.
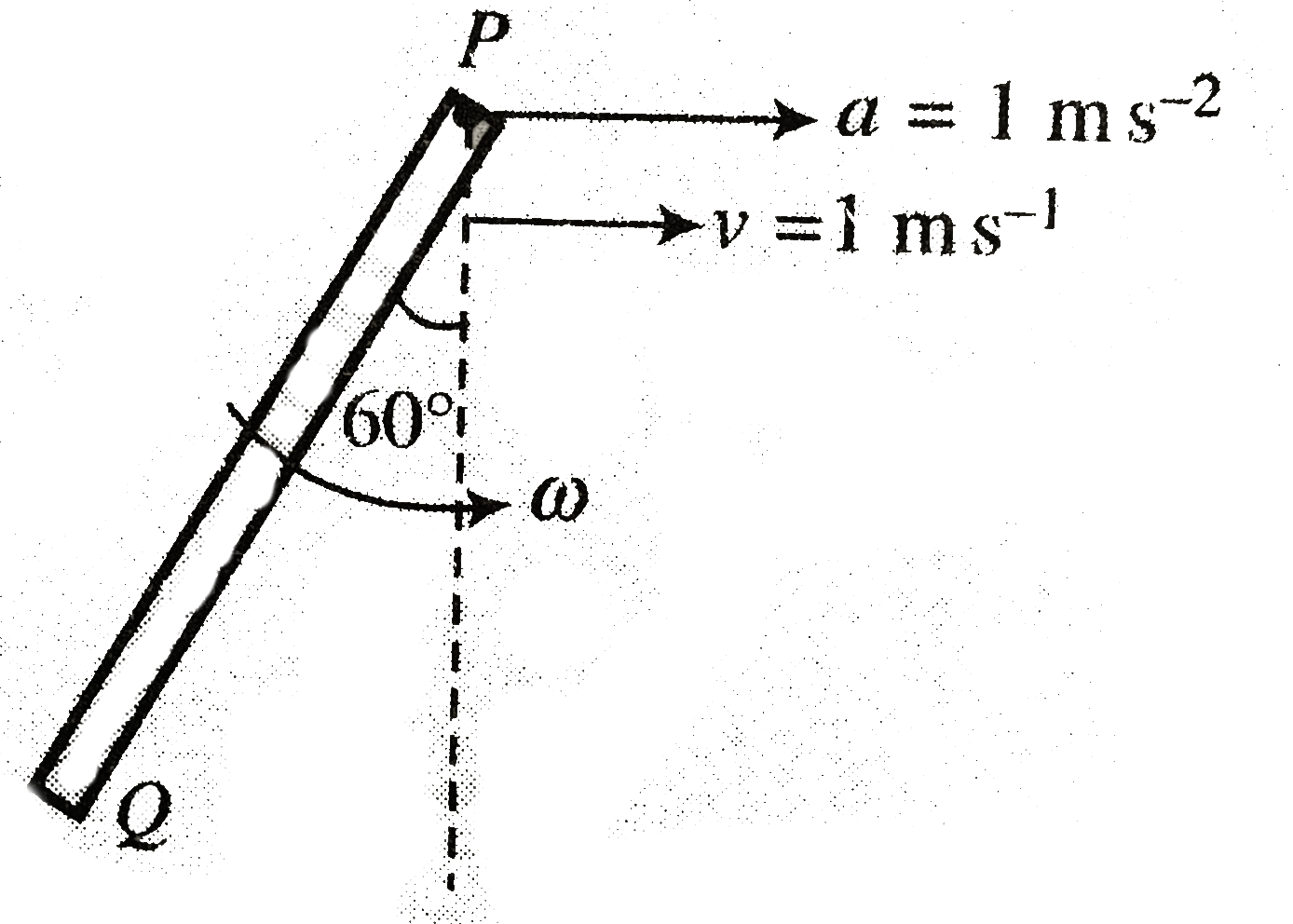
View SolutionA cannon files successively two shells with velocity v0=250m/s, the first at the angle θ1=60∘ and the second at the angle θ2=45∘ to the horizontal, the azimuth being the same. Neglecting the air drag, find the time interval between firings leading to the collision of the shells.
View SolutionThe acceleration of a particle varies with time t as
a=t2+t+2
where t is in seconds.
The particle starts with an initial velocity v-3m/s at t=0. find the velocity of the particle at the end of 5s.
View Solution