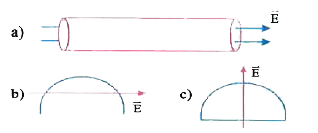
A hemispherical body of radius R is placed in a uniform electric field E. What is the flux linked with the curved surface if, the field is (a) parallel to the base, (b) perpendicular to the base.
Considering the hemispherical body as a closed body with a curved surface (CS) and a plane surface (PS), the total flux (ϕ) linked with the body will be zero, as no charge is enclosed by the body
I,e., ϕ=ϕCS+ϕPS=0 .. (i)
It implies that number of electric field lines leaving the surface.
(a) When field is parallel to the base,
ϕPS=E×πR2cos90∘=0
∴ From (i), ϕ=ϕCS=0
(b) When field is perpendicular ot the base,
ϕPS=E×πR2cos180∘=−EπR2
∴ from (i) ϕCS−EπR2=0 or ϕCS=EπR2
Similar Questions
A hemispherical body is placed in a uniform electric field E. What is the flux linked with the curved surface if the field is (a) parallel to base of the body , (b) perpendicular to base of the body and (c) perpendicular to the curved surface at every points as in figure.

View SolutionIf a hemispherical body is placed in a uniform electric field E then the flux linked with the curved surface is E B
View Solution
Knowledge Check
A hemispherical bowl of radius r is placed in uniform electric field E . The electric flux passing through the bowl is
Aπr2EB2πrE2C4πr2ED4πrE2A hemispherical body of radius R is placed in a uniform electric field E. If the field E is parallel to the base of the hemisphere the flux linked with it is
A2πREB2πR2ECπR2EDzeroIn a uniform electric field find the total flux associated with the given surfaces (R is radius)
 Aa=0,b=0,c=0,Ba=0,b=(πR2E),c=0Ca=2πRE,b=(πR2E),c=0Da=πR2E,b=0,c=0
Aa=0,b=0,c=0,Ba=0,b=(πR2E),c=0Ca=2πRE,b=(πR2E),c=0Da=πR2E,b=0,c=0
A metal slab is placed in a uniform electric field →E. What will be the net electric field intensity inside it?
View SolutionIn a uniform electric field find the total flux associated with the given surfaces (R is radius )
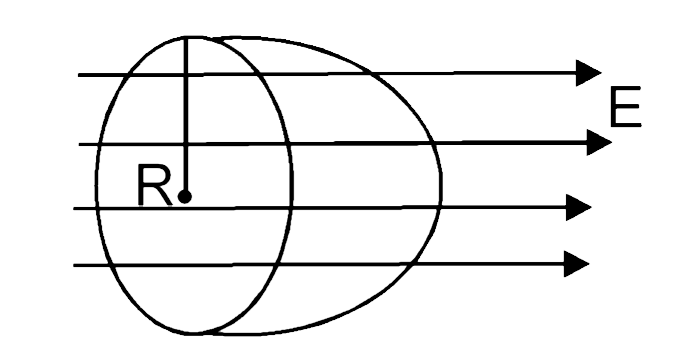
View SolutionA hemisphere (radius R) is placed in electric field as shown in fig. Total outgoing flux is -
View SolutionA cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
View SolutionThe electric flux through a hemispherical surface of radius R placed in a uniform electric field E parallel to the axis of the circular plane is
View Solution