A hemispherical body of radius R is placed in a uniform electric field E. If the field E is parallel to the base of the hemisphere the flux linked with it is
2πRE
2πR2E
πR2E
zero
The correct Answer is:D
To find the electric flux linked with a hemispherical body of radius R placed in a uniform electric field E that is parallel to the base of the hemisphere, we can follow these steps:
1. Understanding the Configuration:
- We have a hemisphere with its flat base facing downwards and the curved surface facing upwards. The electric field E is directed parallel to the flat base of the hemisphere.
2. Electric Flux Definition:
- Electric flux Φ through a surface is defined as:
Φ=∫→E⋅d→A
- For a uniform electric field, this simplifies to:
Φ=E⋅A⋅cosθ
- Where A is the area of the surface and θ is the angle between the electric field and the normal to the surface.
3. Analyzing the Flat Base:
- For the flat base of the hemisphere, the area vector d→A is directed perpendicular to the base (upwards), while the electric field →E is parallel to the base (horizontally).
- Therefore, the angle θ between →E and d→A is 90∘.
4. Calculating Flux through the Base:
- Since cos90∘=0, the flux through the flat base is:
Φbase=E⋅A⋅cos90∘=0
5. Analyzing the Curved Surface:
- For the curved surface of the hemisphere, the electric field lines enter and exit the surface.
- The flux entering the curved surface is equal to the flux exiting the surface. Thus, the net flux through the curved surface is also zero.
6. Total Electric Flux:
- Since both the flat base and the curved surface contribute zero flux, the total electric flux linked with the hemisphere is:
Φtotal=Φbase+Φcurved=0+0=0
Conclusion:
The electric flux linked with the hemispherical body in the given uniform electric field is:
Φ=0
- We have a hemisphere with its flat base facing downwards and the curved surface facing upwards. The electric field E is directed parallel to the flat base of the hemisphere.
- Electric flux Φ through a surface is defined as:
Φ=∫→E⋅d→A
- For a uniform electric field, this simplifies to:
Φ=E⋅A⋅cosθ
- Where A is the area of the surface and θ is the angle between the electric field and the normal to the surface.
- For the flat base of the hemisphere, the area vector d→A is directed perpendicular to the base (upwards), while the electric field →E is parallel to the base (horizontally).
- Therefore, the angle θ between →E and d→A is 90∘.
- Since cos90∘=0, the flux through the flat base is:
Φbase=E⋅A⋅cos90∘=0
- For the curved surface of the hemisphere, the electric field lines enter and exit the surface.
- The flux entering the curved surface is equal to the flux exiting the surface. Thus, the net flux through the curved surface is also zero.
- Since both the flat base and the curved surface contribute zero flux, the total electric flux linked with the hemisphere is:
Φtotal=Φbase+Φcurved=0+0=0
The electric flux linked with the hemispherical body in the given uniform electric field is:
Φ=0
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NARAYNACHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - C.U.Q
81 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NARAYNACHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EXERCISE -1 (C.W)
24 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NARAYNACHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EVALUATE YOURSELF-3
7 Videos
- DUAL NATUREBOOK - NARAYNACHAPTER - DUAL NATUREEXERCISE - LEVEL-II (H.W)
17 Videos
- ELECTRO MAGNETIC INDUCTIONBOOK - NARAYNACHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - Level-II (H.W)
23 Videos
Similar Questions
If a hemispherical body is placed in a uniform electric field E then the flux linked with the curved surface is E B
View SolutionA hemispherical body is placed in a uniform electric field E. What is the flux linked with the curved surface if the field is (a) parallel to base of the body , (b) perpendicular to base of the body and (c) perpendicular to the curved surface at every points as in figure.

View Solution
Knowledge Check
A hemispherical bowl of radius r is placed in uniform electric field E . The electric flux passing through the bowl is
Aπr2EB2πrE2C4πr2ED4πrE2A hemispherical body of radius R is placed in a uniform electric field E. What is the flux linked with the curved surface if, the field is (a) parallel to the base, (b) perpendicular to the base.
Aϕ=(E(πR)Bϕ=(ES=E(πR3)Cϕ=E(πR2)Dϕ=(ES=E(πR4)The electric flux through a hemispherical surface of radius R placed in a uniform electric field E parallel to the axis of the circular plane is
A(2πR)EB(πR2)EC(43πR3)ED(23πR3)E
A cylinder of radius R and length l is placed in a uniform electric field E parallel to the axis of the cylinder. The total flux over the curved surface of the cylinder is
View SolutionA charge q is placed in an uniform electric field E. If it is released, then the K.E of the charge after travelling distance y will be :
View SolutionA hemisphere (radius R) is placed in electric field as shown in fig. Total outgoing flux is -
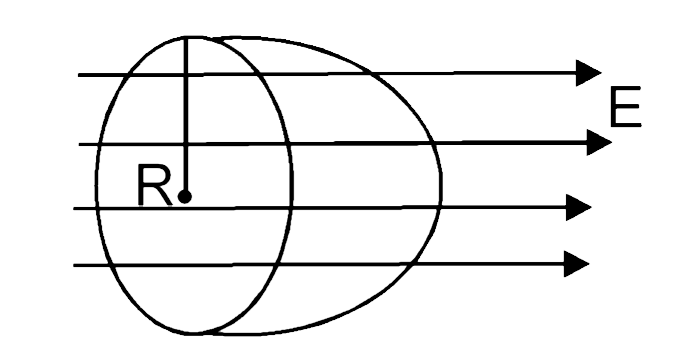
View SolutionA cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
View SolutionA charge q is placed in an uniform electric field E. If it is released, then the K.E of the charge after travelling distance y will be :
View Solution
