State Gauss's law in electrostatics. Applying Gauss's law derive the expression for electric intensity due to an infinite plane sheet of charge.Solution in Telugu
Gauss's law : The total electric flux through any closed surface is equal to 1ε0 times the net charge enclosed by the surface. i.e., ϕ=∮s→E.d→s=qε0.
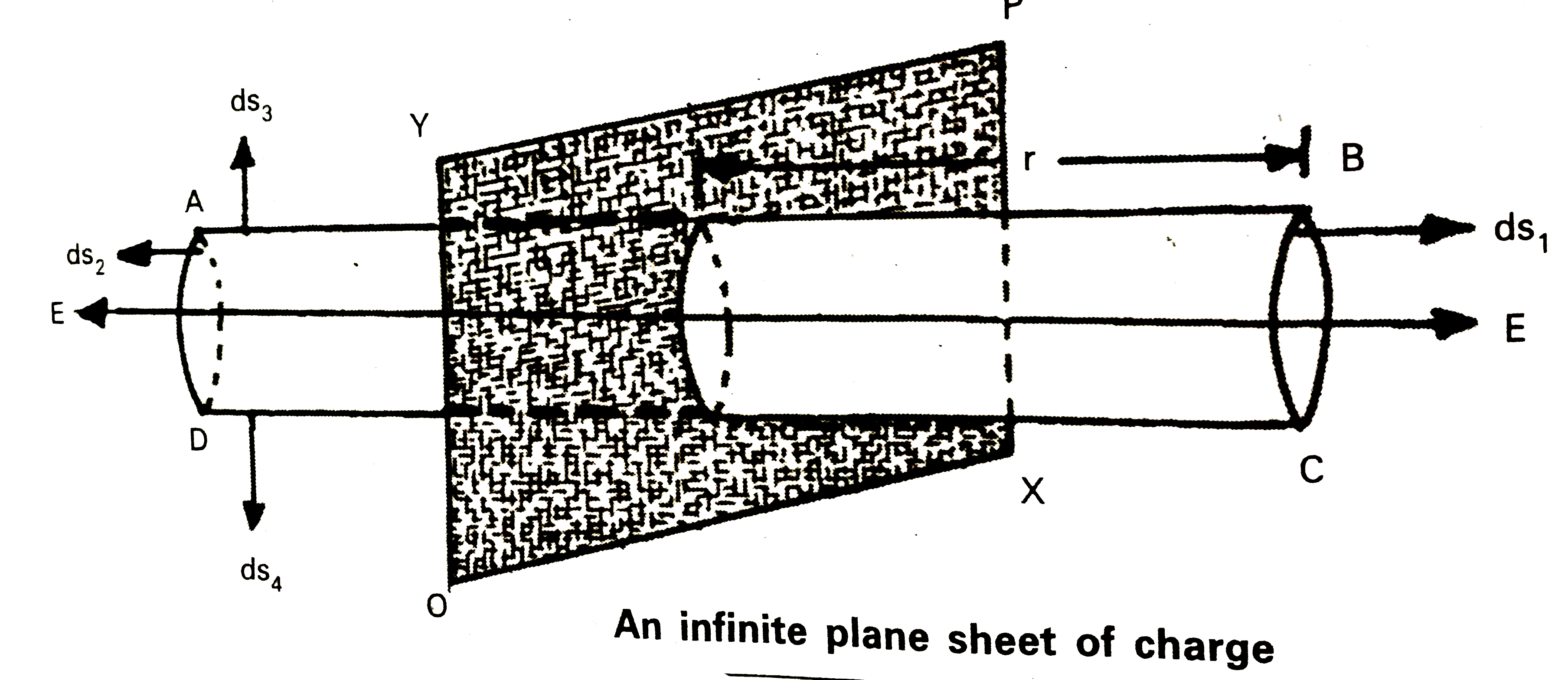
Expression for E due to an infinite plane sheet of charge :
(1) Consider an infinite plane sheet of charge. Let the charge distribution is uniform on this plane.
Uniform charge density on this surface σ=dqdS where is the charge over an infnite small area ds.
(3) Construct a horizontal cylindrical Gaussian surface ABCD perpendicular to the plane with length 2r.
(4) The flat surface BC and AD are parallel to the plane sheet and are at equal distance from the plane.
(5) Let area of these surfaces are dS1 and dS2. They are parallel to →E. So flux through these two surfaces is ∮s→E.d→S=∫EdS=E(S+S)=2ES ...(1)
Where S is area of plane surface AD or BC. Both are equal in area and intensity.
(6) consider cylindrical surface of AB and CD. Let their areas are say dS3 and dS4. These surface are ⊥lr to electric intensity →E.
(7) So angle between →E and d→S3 or dS4 is 90∘. Total flux through these surface is zero. Since ∮S→E.d→S=0.
(8) From Gauss's law total flux, ϕ=∮→E.d→S=2ES=qε0
∴2ES=σSε0[∵Q=σ×S]
(9) Therefore intensity of electric field to an infinite plane sheet of charge E=σ2ε0
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - TEXTUAL EXERCISES
11 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - ADDITIONAL EXERCISES
53 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - SHORT ANSWER QUESTIONS
6 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - DAM SURE (VSAQ)
2 Videos
- ELECTROMAGNETIC INDUCTION BOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - Additional EXERCISES
15 Videos
Similar Questions
Two small identical balls, each of mass 0.20 g, carry identical charges and are suspended by two threads of equal lengths. The balls position themselves at equilibirum such that the angle between the threads is 60∘. If the distance between the balls is 0.5 m, find the charge on each ball.
View SolutionWhat is the force between two small charged soheres having charges of 2×10−7C and 3×10−7C placed 30 cm apart in air ?
View SolutionState and explain Coulomb's incerse square law in electricity.
View SolutionThe wavelength of electromagnetic radiation is doubled. What will happen to the energy of the photons of the new radiation ?
View SolutionSuppose the loop in Textual Exercise 4 is stationary but the current feeding the electromagnet that produces the magnetic field is gradually reduced so that the field decreases from its initial value of 0.3 T at the rate of 0.02Ts−1 .If the cut is joined and the loop has a resistance of 1.6Ω how much power is dissipated by the loop as heat ? What is the source of this power ?
View SolutionState and prove Gauss's law in electrostatics.
View SolutionState Gauss's law in electrostatics. Using this law derive an expression for the electric field due to a uniformly charged infinite plane sheet.
View SolutionState Gauss's law in electrostatics. Use this law to derive an expression for the electric field due to an infinitely long straight wire of linear charge density λcm−1
View Solutionगॉस के नियम द्वारा रेखीय आवेश के कारण विद्युत् क्षेत्र की तीव्रता ज्ञात कीजिए ।
View SolutionState and explain Gauss's law in electrostatics.
View SolutionState Gauss's law. Derive an expression for electric intensity at a point outside the uniformly charged shell.
View SolutionState Gauss's theorem. Obtain an expression for elactric field at any point outside a charged spherical hollow conductor (shell).
View SolutionState Gauss's law in electrostatics. Using this law derive an expression for the electric field due to a uniformly charged infinite plane sheet.
View SolutionState Gauss's law in electrostatics. Use this law to derive an expression for the electric field due to an infinitely long straight wire of linear charge density lamda cm^(-1)
View SolutionApply Gauss's law to find the electric field due to an infinitely long plane sheet of charge.
View Solution
