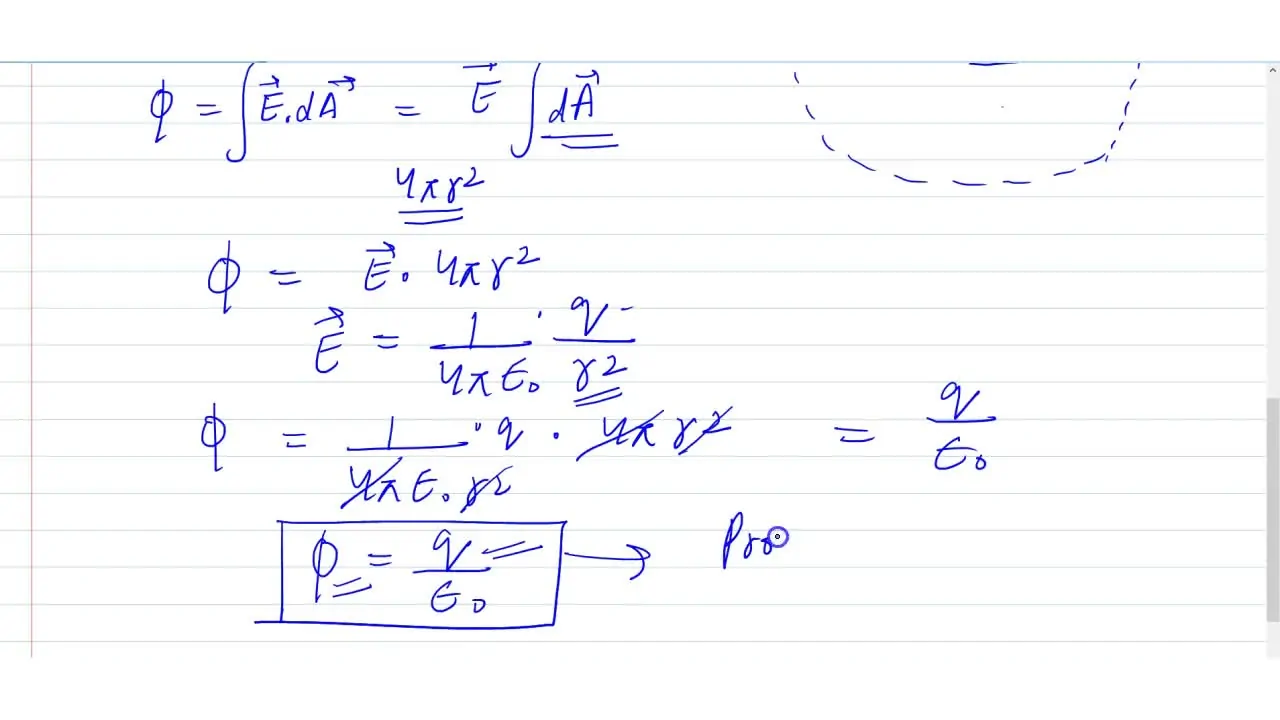
State and prove Gauss's law in electrostatics.
Step by step video & image solution for State and prove Gauss's law in electrostatics. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
State Gauss' law in electrostatic.
View SolutionState and prove Gauss's law in electrostatics.
View SolutionState and prove Hooke's law.
View SolutionState Gauss's law in electrostatics. Using this law derive an expression for the electric field due to a uniformly charged infinite plane sheet.
View SolutionState Gauss's law in electrostatics. Use this law to derive an expression for the electric field due to an infinitely long straight wire of linear charge density λcm−1
View SolutionWhat is the nature of Gaussian surface involved in Gauss's law of electrostatics?
View SolutionState Gauss' law in electrostatic. Using it derive an expression for the electric field due to an infinitely long straight wire of linear charge density λ C/m.
View SolutionState Gauss's law on electrostatics and derive an expression for the electric field due to a long straight thin uniformly charged wire (linear charge density ) at a point lying at a distance r from the wire.
View SolutionThe unity of the electriec and magnetic waves was found by Maxwell from
(i) Guss's law in electrostatics
(ii) Gauss's law in magnetism
(iii) Faraday's law of electromagnetic induction.
(iv) Ampere's law with displacement current.View SolutionState the limitation of Coulomb's law in electrostatics.
View Solution