An isosceles triangles is formed with a thin rod of length l1 and coefficient of linear expansion α1, as the base and two thin rods each of length l2 and coefficient of linear expansion α2 as the two sides. The distance between the apex and the midpoint of the base remain unchanged as the temperature is varied. the ratio of lengths l1l2 is

l1l2=2√α2α1
l1l2=2√α1α2
l1l2=√α1α2
l1l2=√α2α1
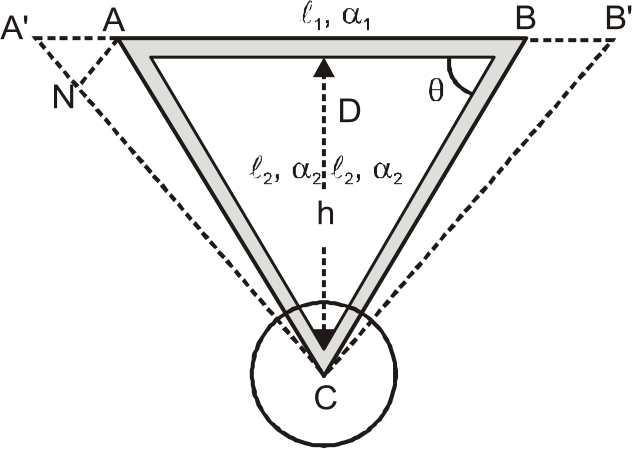
According to condition of the problem height of the isosceles triangle ABC is unchanged. The dotted lines show configuration after a temperature rise. Increase in length of AC is A'N
Considering increase in angle θ to be very small.
A'N=AA'cosθ
where cos θ=l12l2
thus we have l1α2△T=(12l1α1△T)(l12l2)
Hence l1l2=2√α2α1
Topper's Solved these Questions
Similar Questions
A radioactive element decays by β−emission. A detector records n beta particles in 2s and in next 2s it records 0.75n beta particles. Find mean life correct to nearest whole number. Given ln |2|=0.6931, ln |3|=1.0986.
05:34View SolutionWhen p−n junction diode is forward biased then
02:08View SolutionAn isosceles triangle is formed with a thin rod of length l1 and coefficient of linear expansion α1, as the base and two thin rods each of length l2 and coefficient of linear expansion α2 as the two sides. The distance between the apex and the midpoint of the base remain unchanged as the temperature is varied. the ratio of lengths l1l2 is
 03:32View Solution
03:32View SolutionA composite bar of length L = L_(1) + L_(2) is made up from a rod of material 1 and of length L_(1) attached to a rod of material 2 and of length L_(2) as shown. If alpha_(1) and alpha_(2) are their respective coefficient of linear expansion, then equivalent coefficient of linear expansion for the composite rod is
01:45View SolutionTwo rods of length l_(1) and l_(2) are made of material whose coefficient of linear expansion are alpha_(1) and alpha_(2) , respectively. The difference between their lengths will be independent of temperatiure if l_(1)//l_(2) is to
03:12View SolutionTwo rods of lengths L1 and L2 are welded together to make a composite rod of length (L1+L2). If the coefficient of linear expansion of the materials of the rod are α1 and α2 respectively. The effective coefficient of linear expansion of the composite rod is
05:57View SolutionTwo rods having length l1 and l2, made of materials with the linear coefficient of expansion α1 and α2 were welded togther. The equivalent coefficients of linear expansion for the obtained rod:-
 02:05View Solution
02:05View SolutionTwo rods each of length L2 and coefficient of linear expansion α2 each are connected freely to a third rod of length L1 and coefficient of expansion α1 to form an isoscles triangle. The arrangement is supported on a knife-edge at the midpoint of L1 which is horizontal. what relation must exist between L1 and L2 so that the apex of the isoscles triangle is to remain at a constant height from the knife edge as the temperature changes ?
02:56View SolutionTwo metal rods of lengths L_(1) and L_(2) and coefficients of linear expansion alpha_(1) and alpha_(2) respectively are welded together to make a composite rod of length (L_(1)+L_(2)) at 0^(@)C. Find the effective coefficient of linear expansion of the composite rod.
02:10View SolutionTwo rods of length L1 and L2 are made of materials of coefficients of linear expansions α1 an α2 respectively such that L1α1=L2α2. The temperature of the rods is increased by ΔT and correspondingly the change in their respective lengths be ΔL1 and ΔL2
View SolutionTwo rods of different metals of coefficient of linear expansion α1 and α2 and initial length l1 and l2 respectively are heated to the same temperature. Find relation in α1,α2,l1 and l2such that difference between their lengths remain constant.
02:39View SolutionTwo rods, each of coefficient of linear expansion alpha_(2) and length l_(2) , form the two sides of an isosceles triangle and the base is formed by another rod of length l_(1) and coefficient of linear expansion alpha_(1). The base is fixed horizontally at its mid-point. What should be the relationship between l_(1) " and " l_(2) so that the distance of the vertex from the mid-point of the base, does not change for any increase in temperature?
01:26View SolutionTwo rods having length l_(1) and l_(2) , made of materials with the linear coefficient of expansion alpha_(1) and alpha_(2) were welded together. The equivalent coefficients of linear expansion for the obtained rod:-
04:22View SolutionTwo rods of length l_(1) and l_(2) are made of material whose coefficient of linear expansion are alpha_(1) and alpha_(2) , respectively. The difference between their lengths will be independent of temperatiure if l_(1)//l_(2) is to
03:44View SolutionAn isosceles triangles is formed with a thin rod of length l1 and coefficient of linear expansion α1, as the base and two thin rods each of length l2 and coefficient of linear expansion α2 as the two sides. The distance between the apex and the midpoint of the base remain unchanged as the temperature is varied. the ratio of lengths l1l2 is
 05:43View Solution
05:43View SolutionAn isosceles triangles is formed with a thin rod of length l1 and coefficient of linear expansion α1, as the base and two thin rods each of length l2 and coefficient of linear expansion α2 as the two sides. The distance between the apex and the midpoint of the base remain unchanged as the temperature is varied. the ratio of lengths l1l2 is
 03:58View Solution
03:58View Solution

