Tweleve indentical resistor are arranged on all edfges of a cube. Then find the equivalent resistance between the corners 1 and 6 as shwon in fig 5.126(us nodal method) 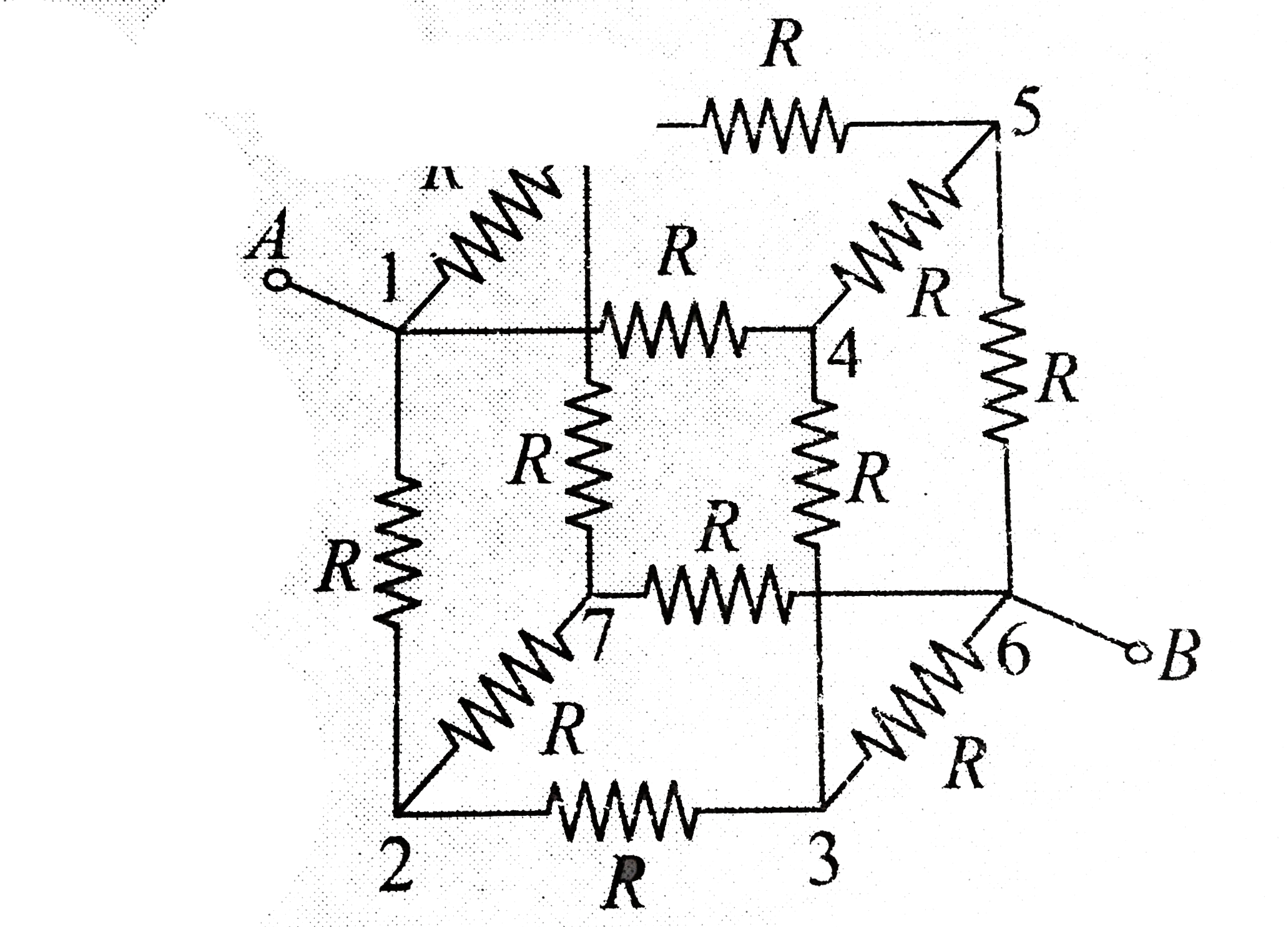
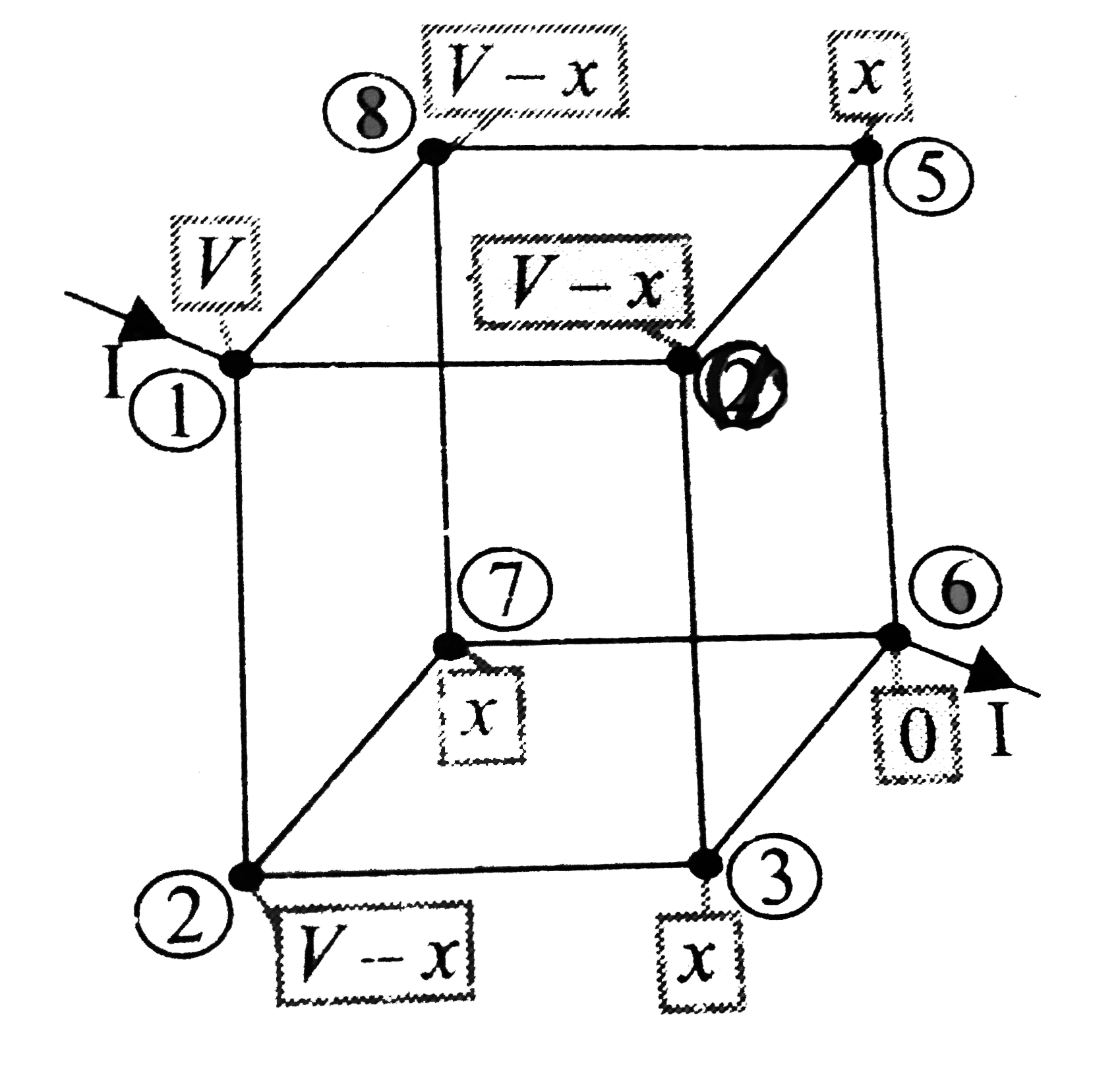
Let us assume we have
connected a battery of emf V
across points 1 and 6. if potential
of point 1 is V, then the potential
of point 6 is 0.
From symmetry, it is clear that
the current entering at point1 will equally divide the resistance. Hence, the potential differences
across resistances, conncted
across (1,8) , (1,2), and (1,4), will be equal .
Similarly the current coming out at point 6 will be equal
to current ennering at point 1. By symmetry, we can say the current in the resistances connected across points (7,6),
(3,6), and (6,5) will be equal. Hence, potential difference
across these resistance should be equal. Let us assume the
potentials at points 3,5, and 7 be x then the potentials at points 2,4, and 8 should be (V - x). At point 7,
[x−(V−x)]R+x−0R+[x−(V−x)]R=0
or x25V(i)
At point 1, I=3[V−(V−x)]R=3xR(ii)
From (i) and (ii)
VI=5R6
Hence, effective resistance will be 5R/6.
Topper's Solved these Questions
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Solved Examples
12 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Exercise 5.1
28 Videos
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits
15 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
Similar Questions
Twelve identical resistances arranged on all edges of a cube. The resistors are all the same. Then find the equivalent resistance between the edges A and B as shown in.

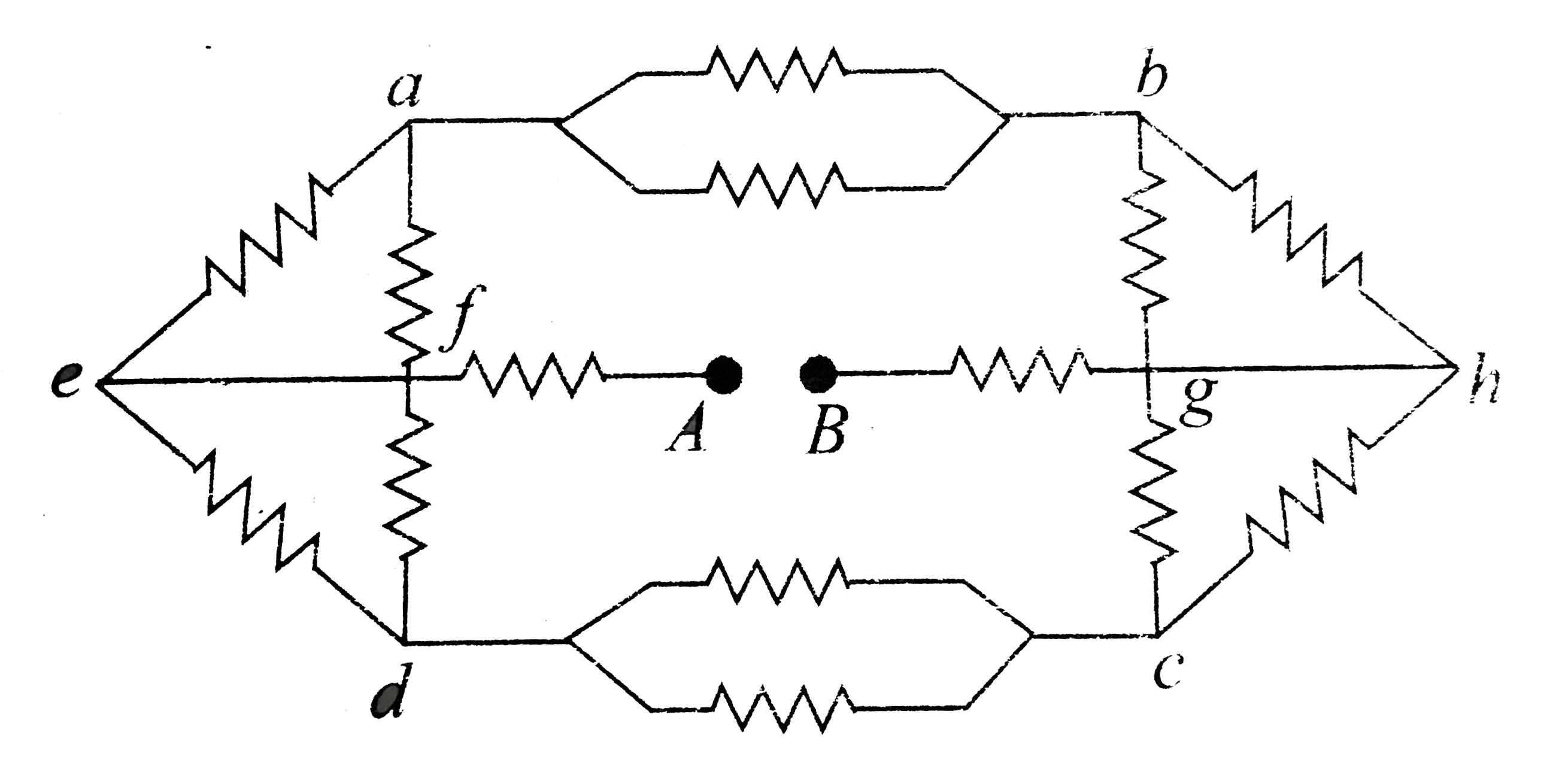
View SolutionFind the equivalent resistance between the points A and B of the network of resistors shown in Fig. 4.16.
View Solution
Knowledge Check
Each of the resistor shown in fig. has resistance R. Find the equivalent resistance between A and B
 A7R4B5R4C9R4D11R4
A7R4B5R4C9R4D11R4Five resistance, each of 5Ω are connected as shown in figure. Find the equivalent resistance between points (1) A and B , (2) A and C .
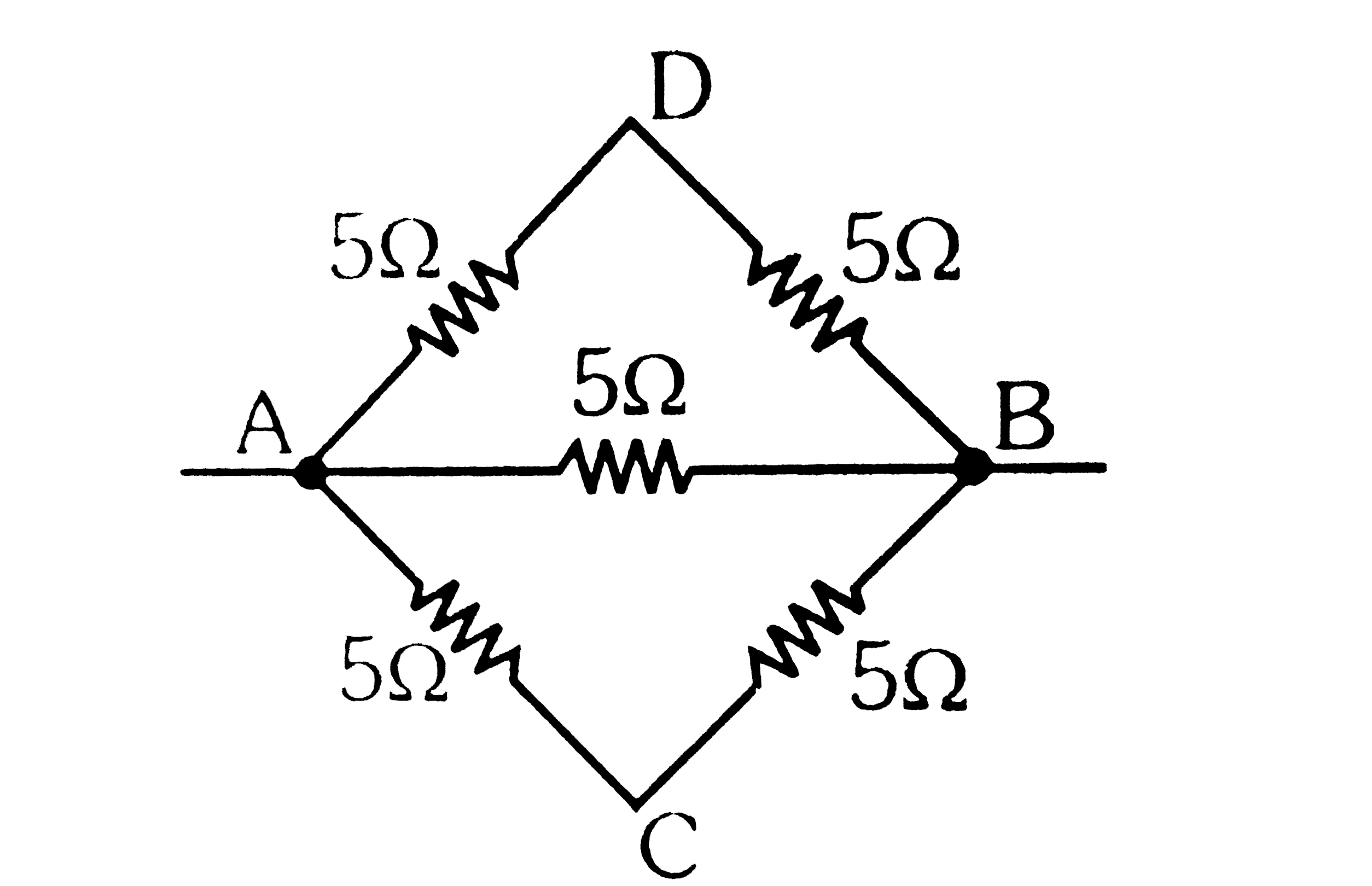 A(1) 7.5Ω (2) 2.25ΩB(1) 5Ω (2) 2.50ΩC(1) 2.5Ω (2) 3.13ΩD(1) 3Ω (2) 2.5Ω
A(1) 7.5Ω (2) 2.25ΩB(1) 5Ω (2) 2.50ΩC(1) 2.5Ω (2) 3.13ΩD(1) 3Ω (2) 2.5ΩIn the infinite gride. If the value of r=2(√5−1)Ω. Find the Equivalent resistance between the point a and c.
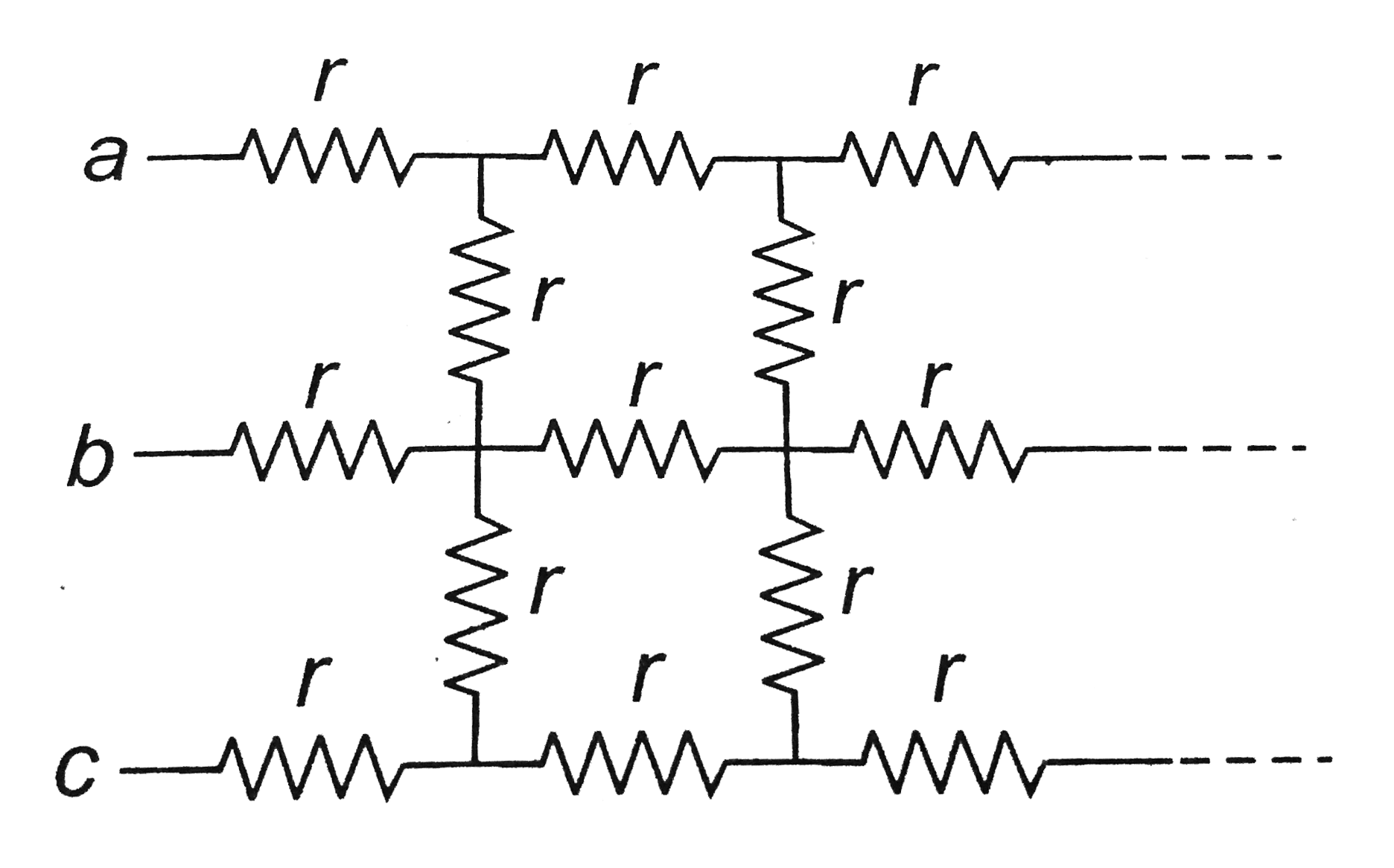 A6(√5−1)ΩB4(√5−1)ΩC4ΩDnone of these
A6(√5−1)ΩB4(√5−1)ΩC4ΩDnone of these
Twelve equal resistors each R Ω are connected to from the edges of a cube . Find the equivalent resistance of the network.
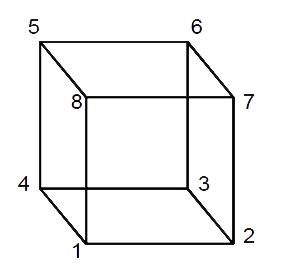
When current enters at 1 and leaves at 3
View SolutionTwelve equal resistors each R Ω are connected to from the edges of a cube . Find the equivalent resistance of the network.
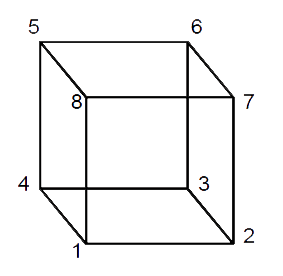
When current enters at 1 and leaves at 2
View SolutionTwelve equal resistors each R Ω are connected to from the edges of a cube . Find the equivalent resistance of the network.
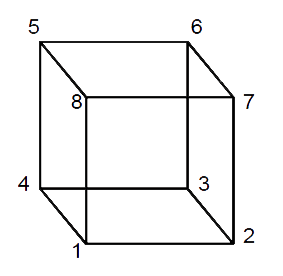
When current enters at 1 & leaves at 6 (body diagonal)
View SolutionAll resistances in fig. 5.34 are in ohm. Find the effective resistance (in Ω) between points A and B.

View SolutionFor the combination of resistors shown in (fig.)
(a) find the equivalent resistance between
(i) C and D and
(ii) A and B.
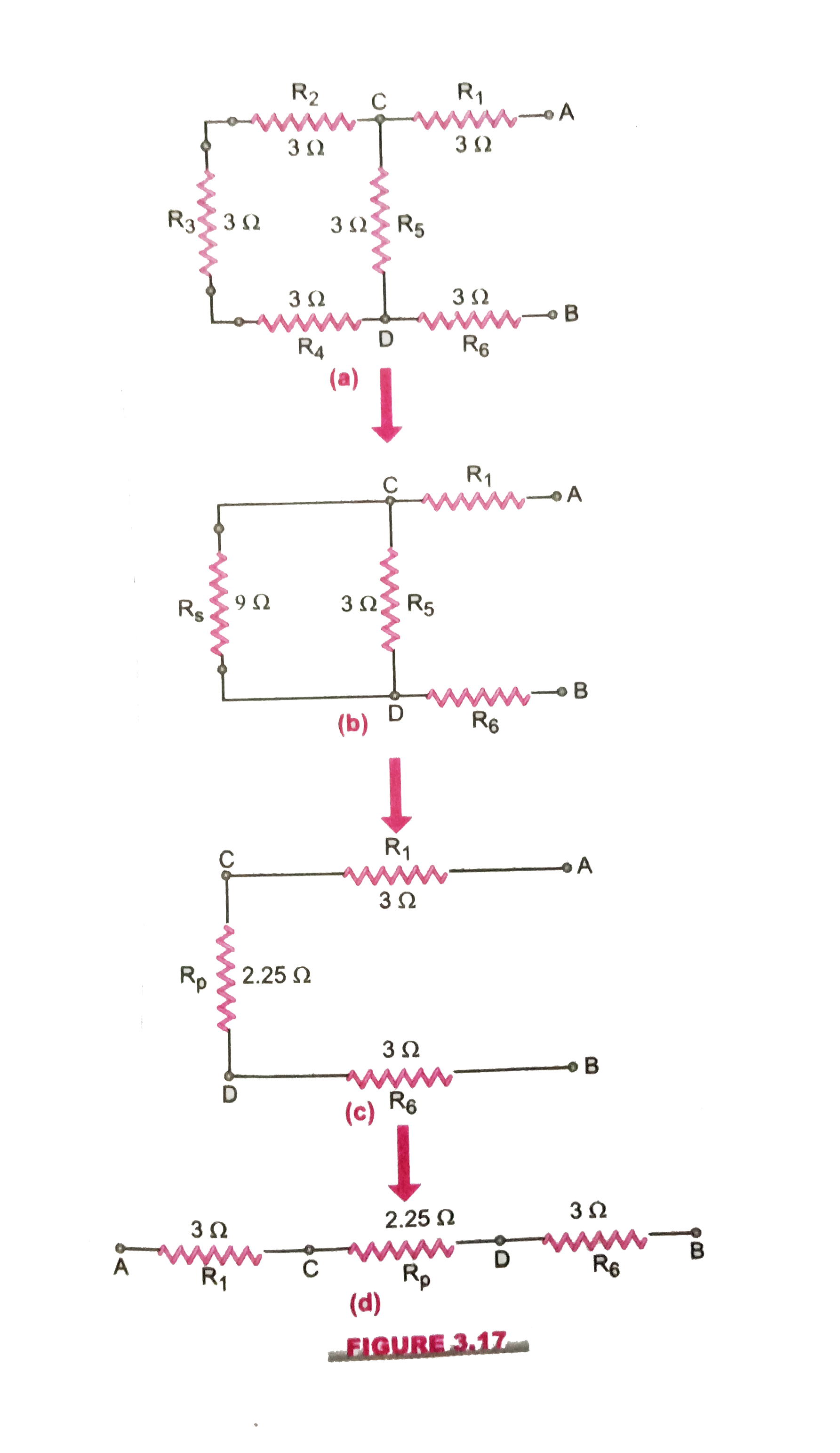 .
.
View Solution
