A particle starts from rest and moves in a circular motion with constant angular acceleration of 2rads−2. Find
(a) Angular velocity
(b) Angular displacement of the particle after 4s.
( c) The number of revolutions completed by the particle during these 4s.
(d) If the radius of the circle is 10cm, find the magnitude and direction of net acceleration of the particle at the end of 4s.
The correct Answer is:(a) 8rads−1;
(b) 16rad;
( c) 2811rev;
(d) tan−1(132).
∝=2rads−2,ω1=0,t=4s
(a) ω2=ω1+∝t⇒ω2=0+2×4=8rads−1
(b) θ=ω1t+12∝t2
⇒θ=0×4+12×2×42=16rad
( c) Irevolution=2πrad⇒1rad=12rev
⇒16rad=162πrev=8πrev=8×722=2811rev
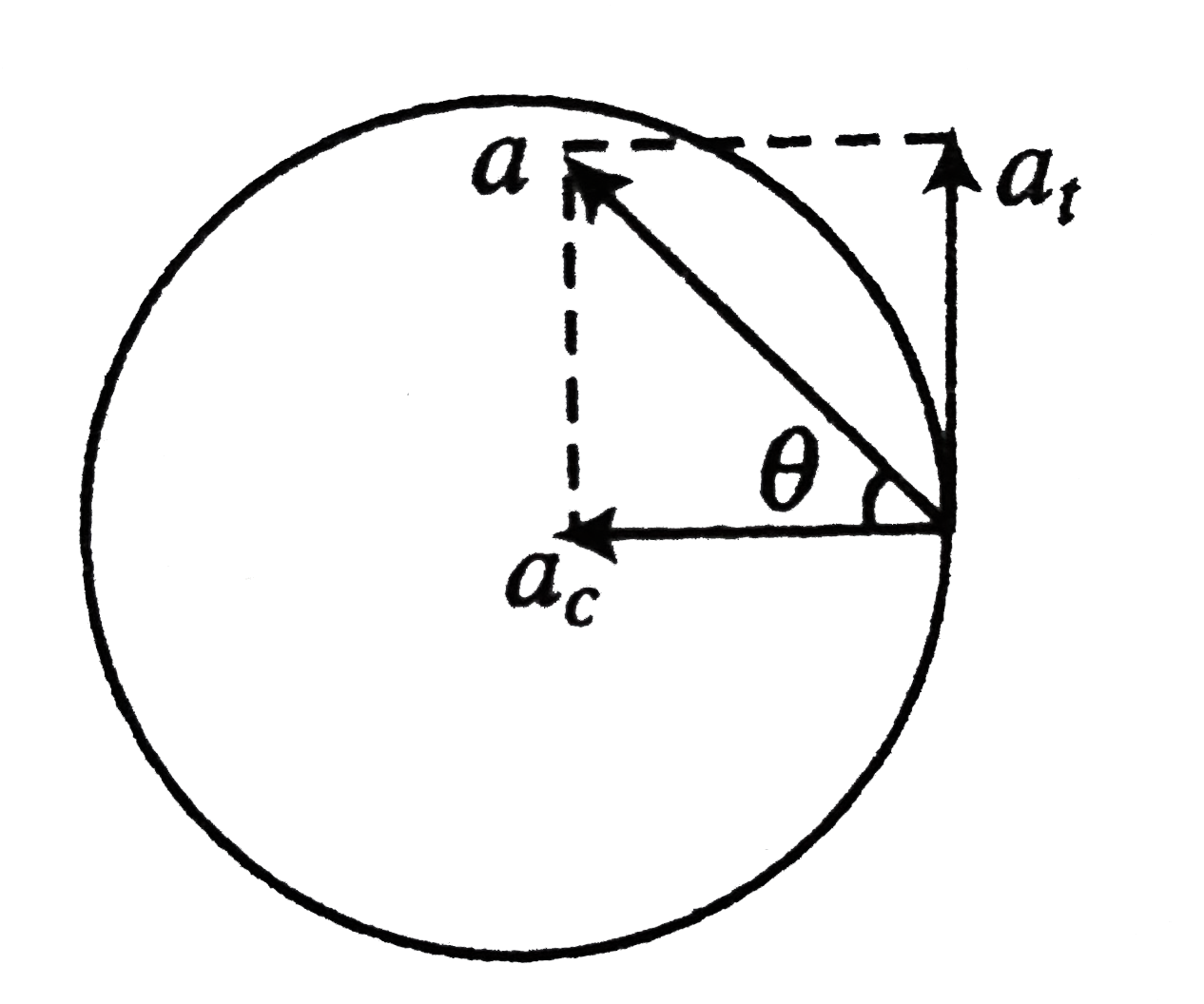
(d) Aftter 4 s : v=ω2r=8×10/100=0.8ms−1
ac=v2r=(0.8)210/100=6.4ms−2
at=∝r=2×10100=0.2ms−2
Now magnitude of net acceleration,
a=√a2c+a2t=√6.4+0.22=√41ms−2
Direction of acceleration,
tanθ=atac=0.26.4=132⇒θ=tan−1(132).
 .
.
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Single Correct
76 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Multiple Correct
11 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.4
11 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
Knowledge Check
A body starts rotating from rest and completes 10 revolutions in 4 s .Find its angular acceleration
A2.5πrad/s2B5πrad/s2C7.5πrad/s2D10πrad/s2A particle starts from rest and moves with an angular acceleration of 3 rad/s2 in circle of radius 3 m . Its linear speed after 5 seconds will be
A15 m/sB30m/sC45m/sD7.5m/sA particle of mass m starts from rest and moves in a circular path of radius R with a uniform angular acceleration α The kinetic energy of the particle after n revolutions is
AnαmR2B2πnαmR2C12mnαR2Dmnαr2
Starting from rest a wheel rotates with uniform angular acceleration 2π rads−2.After 4s ,if the angular acceleration ceases to act,its angular displacement in the next 4s is?
View SolutionA wheel starting from rest via rotating with a constant angular velocity of 3 rad s−1. What is its angular acceleration after 4 s?
View SolutionA particle moves along a circular path a radius 15 cm with a constant angular acceleration of 4 "rad/s"^(2). If the initial angular speed of the particle was 5 rad/s. Find its angular displacement in 5 seconds.
View SolutionA body starts ratating from rest and completes 100 revolutions in 20 s. Find its angular acceleration
View SolutionA particle of mass 2kg start motion form rest on a circular path of radius r=4m with constant tangential acceleration 4ms−2. Find the net work done on the particle in initial 1 second.
View Solution
