A machine gun is mounted on the top of a tower of height h. At what angle should the gun be inclined to cover a maximum range of firing on the ground below ? The muzzle speed of bullet is 150ms−1. Take g=10ms−2.
The correct Answer is:θ=sin−1√u22(u2+gh).
To solve the problem of determining the angle at which a machine gun should be inclined to cover the maximum range when fired from the top of a tower of height h, we can follow these steps:
1. Understanding the Problem:
The machine gun is mounted at a height h and fires bullets with a muzzle speed v=150m/s. We need to find the angle θ that maximizes the horizontal range of the projectile on the ground.
2. Equation of Trajectory:
The trajectory of the projectile can be described by the equation:
y=xtanθ−gx22v2cos2θ
where y is the height, x is the horizontal distance, g is the acceleration due to gravity, and v is the initial velocity.
3. Setting Up the Equation:
Since the gun is fired from a height h, we set y=−h (the bullet will hit the ground). Thus, we have:
−h=xtanθ−gx22v2cos2θ
4. Rearranging the Equation:
Rearranging gives us:
h=xtanθ−gx22v2cos2θ
This is a quadratic equation in x.
5. Finding the Range:
The range R can be found by solving for x when y=0:
0=Rtanθ−gR22v2cos2θ−h
Rearranging gives:
h=Rtanθ−gR22v2cos2θ
6. Maximizing the Range:
To maximize R, we differentiate the equation with respect to θ and set the derivative to zero. However, we can also use the known result that the angle for maximum range on level ground is 45∘.
7. Adjusting for Height:
The angle for maximum range when fired from a height can be approximated using the formula:
tanθ=v2±√v4−g(gR2+2hv2)gR
We can simplify this by substituting R with the maximum range expression derived from the previous steps.
8. Final Calculation:
After substituting the values v=150m/s and g=10m/s2, we can solve for θ using numerical methods or graphically to find the angle that maximizes the range.
Conclusion:
The angle θ that maximizes the range can be determined through the above steps, and it will depend on the height h of the tower.
The machine gun is mounted at a height h and fires bullets with a muzzle speed v=150m/s. We need to find the angle θ that maximizes the horizontal range of the projectile on the ground.
The trajectory of the projectile can be described by the equation:
y=xtanθ−gx22v2cos2θ
where y is the height, x is the horizontal distance, g is the acceleration due to gravity, and v is the initial velocity.
Since the gun is fired from a height h, we set y=−h (the bullet will hit the ground). Thus, we have:
−h=xtanθ−gx22v2cos2θ
Rearranging gives us:
h=xtanθ−gx22v2cos2θ
This is a quadratic equation in x.
The range R can be found by solving for x when y=0:
0=Rtanθ−gR22v2cos2θ−h
Rearranging gives:
h=Rtanθ−gR22v2cos2θ
To maximize R, we differentiate the equation with respect to θ and set the derivative to zero. However, we can also use the known result that the angle for maximum range on level ground is 45∘.
The angle for maximum range when fired from a height can be approximated using the formula:
tanθ=v2±√v4−g(gR2+2hv2)gR
We can simplify this by substituting R with the maximum range expression derived from the previous steps.
After substituting the values v=150m/s and g=10m/s2, we can solve for θ using numerical methods or graphically to find the angle that maximizes the range.
The angle θ that maximizes the range can be determined through the above steps, and it will depend on the height h of the tower.
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Single Correct
76 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Multiple Correct
11 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.4
11 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
A machine gun is mounted on the top of a tower of height h=100 m. At what angle should the gun be inclined to cover a maximum range of firing on the ground below ?The muzzle speed of the bullet is u=150ms−1 and g=10ms−2 .
View SolutionA machine gun is mounted on the top of a tower 60m At what angle showld the gun be inclined to cver a vaximu range of firing on the groun below? The muzzle speed of the bullet is 90ms−1 take g=10ms1.
View Solution
Knowledge Check
A machine gun is mounted on an armored car moving with a speed of 20ms−1. The gun point against the direction of motion of car. The muzzle speed of bullet is equal to speed of sound in air i.e., 340ms−1. The time difference between bullet actually reaching and sound of firing reaching at a target 544m away from car at the instant of firing is reaching at a target 544m away from car at the instant of firing is
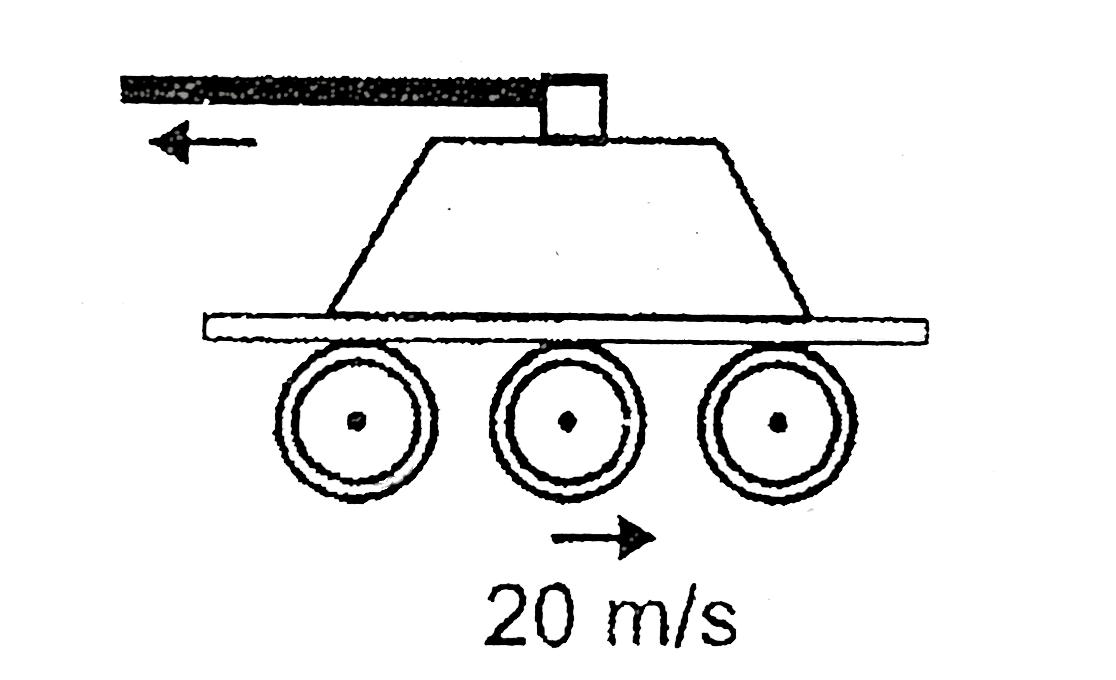 A1.2sB0.1sC1sD10s
A1.2sB0.1sC1sD10sA machine gun fires a bullet of mass 40 g with a velocity 1200ms−1. The man holding it can exert a maximum force of 144 N on the gun. How many bullets can be fire per second at the most?
ATwoBFourCOneDThreeA gun movunted on the top of a voing truck is aimed in the backward direction at an angle of 30∘ to the vertical. If the muzzle velocity of the bullet firee from the gun is 4ms−1, then the speed of the truck that will make the bullet come out vertically is.
A0.5ms−1B1.0ms−1C1.5ms−1D2.0ms−1
A stone is thrown from a cliff 4.2 m high . At what angle he must incline his rifle in order to cover a maximum range of firing on the ground below . Taken muzzle velocity of bullet =20m/s and g=10ms−2.
View SolutionA body is thrown horizontal from the top of a tower and strikes the ground after two seconds at angle of 45∘ with the horizontal. Find the height of the tower and the speed with which the body was thrown. Take g=9.8ms−2.
View SolutionA bullet fired from a gun with a velocity of 140ms−1 strikes the ground at the same level as the gun at a distance of 1 km. Find the angle of inclination with the horizontal at which the bullet is fixed. Take g=9.8ms−2.
View SolutionA machine gun fires 50-g bullets at a speed of 1000 m/s . The gunner , holding the machine in his hands , can exert an average force of 150 N against the gun . Calculate the maximum number of bullets he can fire one minute.
View SolutionA bullet is fired from a gun eith a speed of 1000m/s on order to hit target 100 m away At what height above target should the gun be aimed ? (The resistance of air is negligible and g = 10 m/s2)
View Solution
