In the figure in ΔABC, point D on side BC is such that ∠BAC=∠ADC.
Prove that CA2=CB×CD.
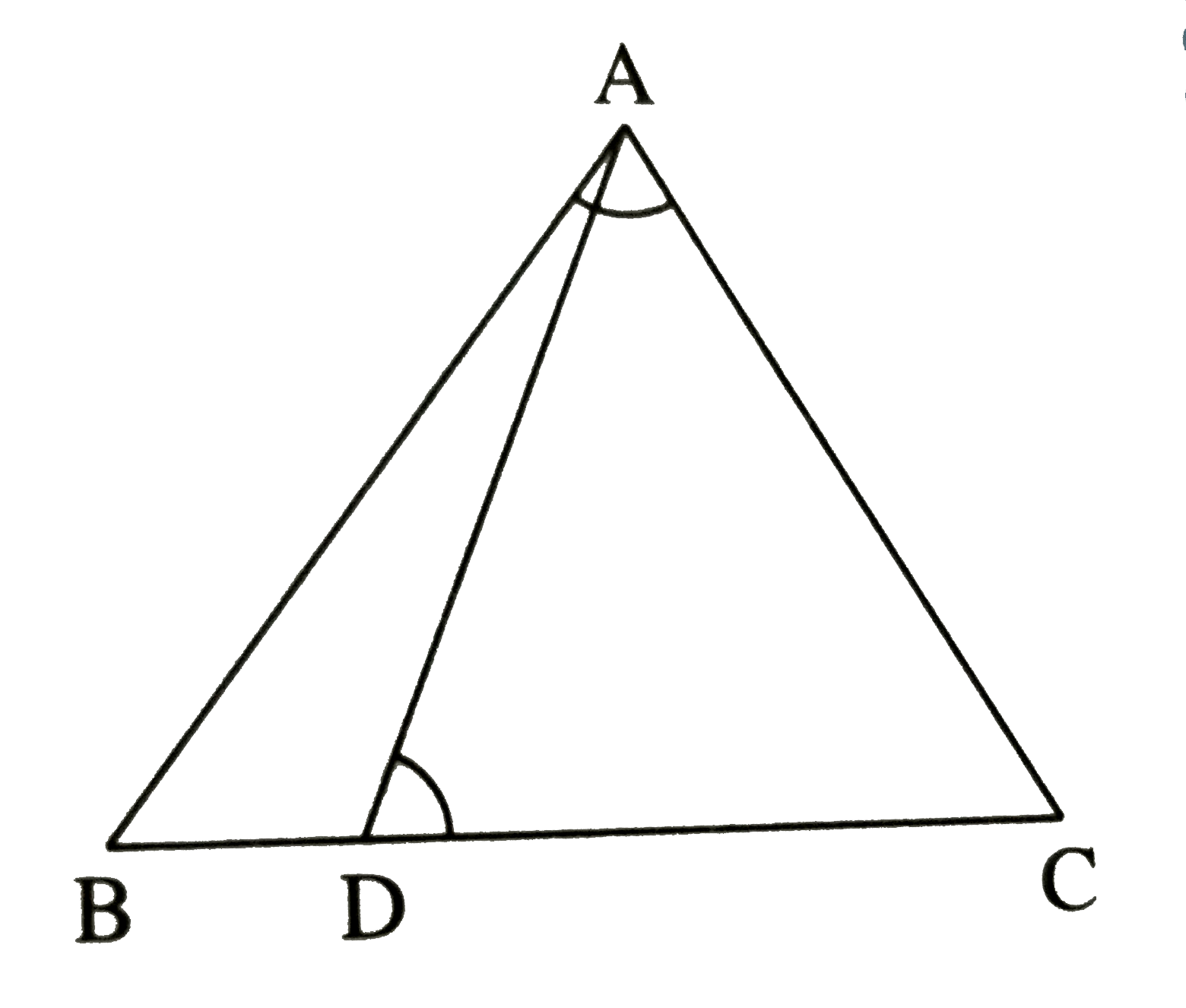
In DBAC and ΔADC
∠BAC≅∠ADC..(Given)
∠ACB≅∠DCA………(Common angle)
∴ΔBAC~ΔADC ………(AA test of similarity)
∴CACD=CBCA ………..(Corresponding sides of similar triangles are in proportion)
∴CA2=CB×CD.
Topper's Solved these Questions
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - CHALLENGING QUESTIONS
8 Videos
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - MCQ TYPE
15 Videos
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - 2.5 (4 mark each)
3 Videos
- QUADRATIC EQUATIONS BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUADRATIC EQUATIONS EXERCISE - ASSIGNEMENT 2.4
8 Videos
- STATISTICSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - STATISTICSEXERCISE - EXAMPLES FOR PRACTICE (MCQs)
35 Videos
Similar Questions
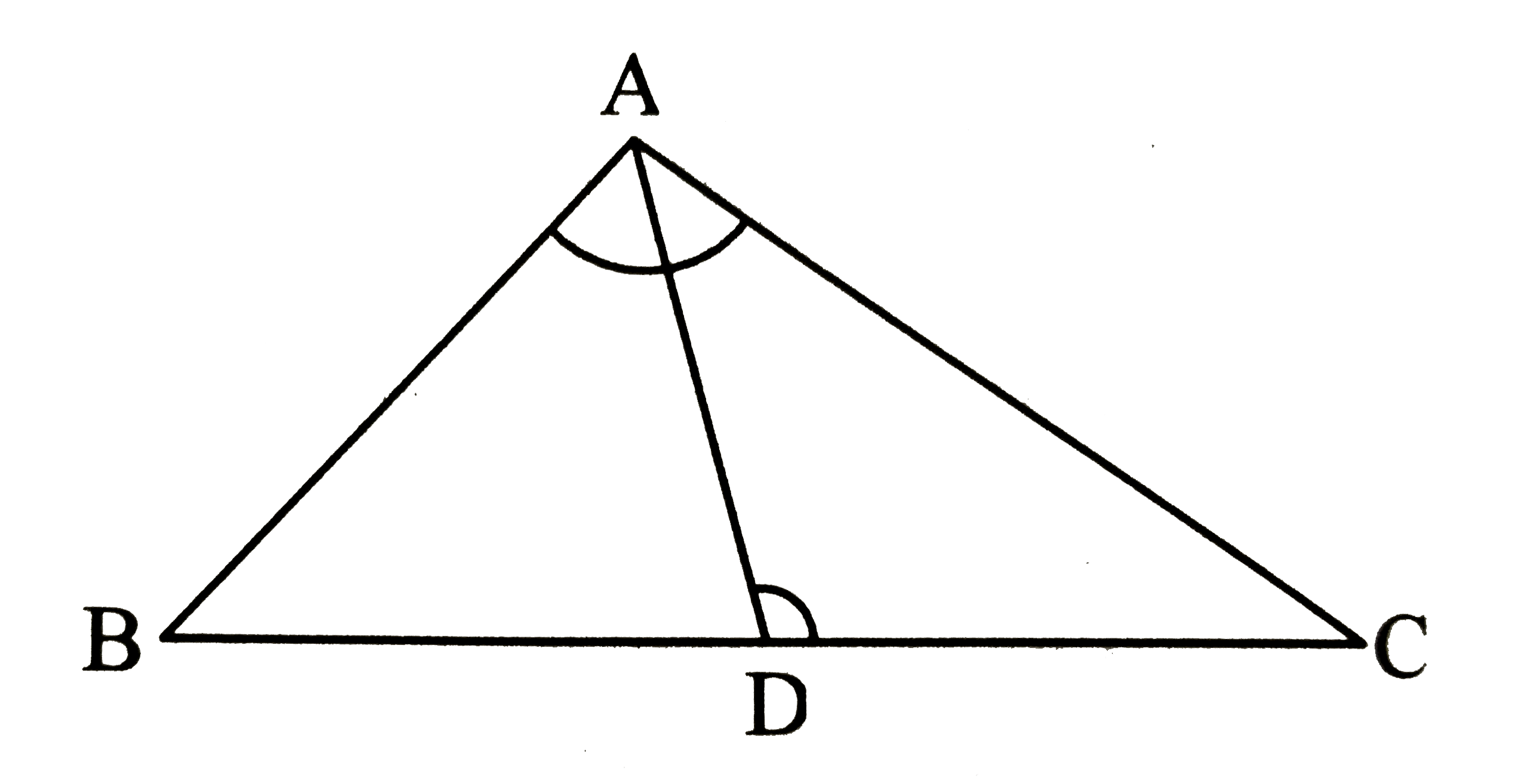
In the adjoining figure, in ΔABC, point D is on side BC such that, ∠BAC=∠ADC. Prove that, CA2=CB×CD.
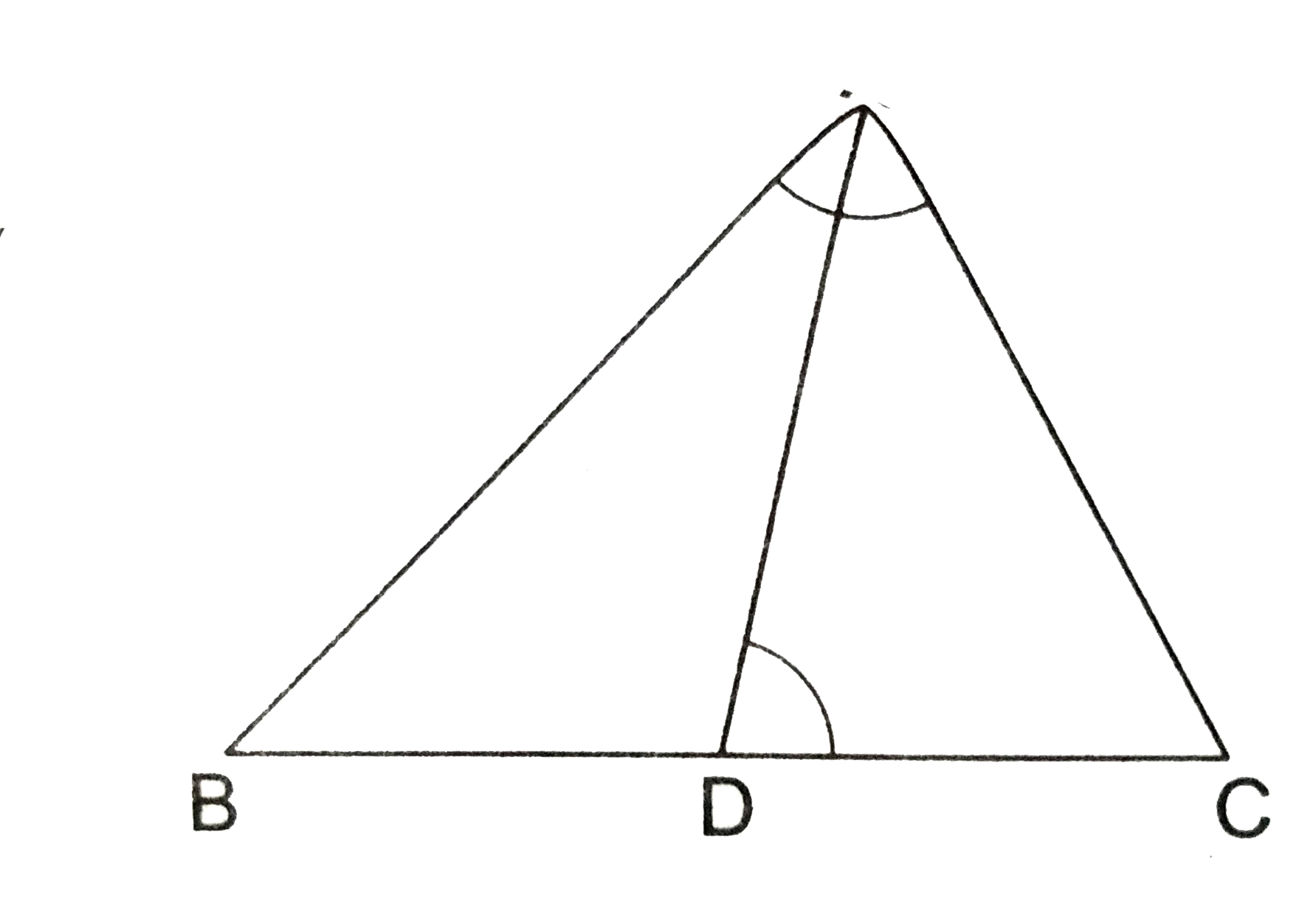
View SolutionIn the given figure , D is a point on the side BC of ΔABC such that ∠ADC=∠BAC. Prove that CA2=CB×CD.
View SolutionD is a point on the side BC of a triangle ABC such that ∠ADC=∠BAC. Show that CA2=CB.CD.
View SolutionD is a point on the side BC of ABC such that ∠ADC=∠BAC . Prove that CACD=CBCA or, CA2=CB×CD .
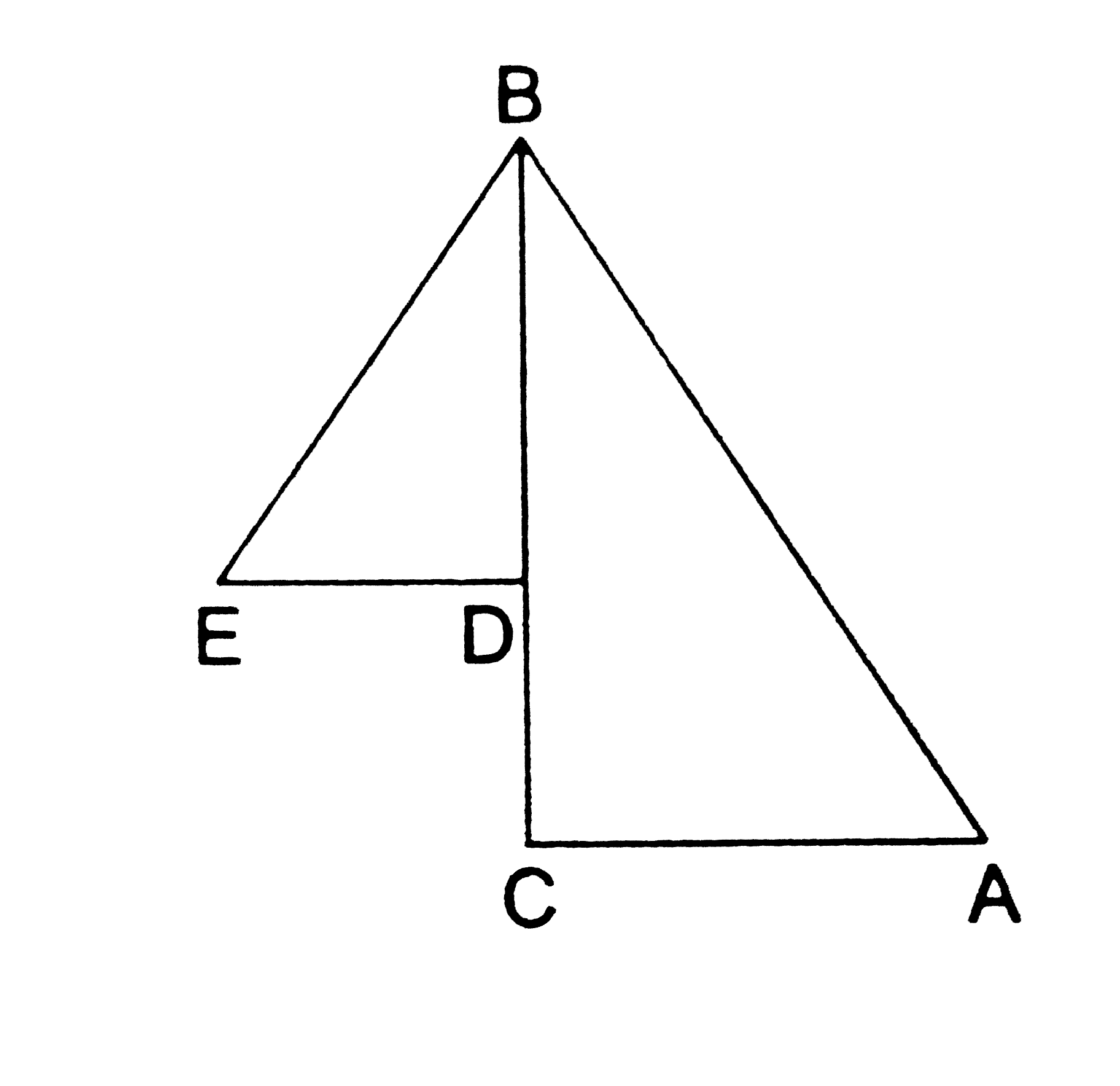
View SolutionIn the given figure, D is a point on side BC of a ΔABC and E is a point such that CD = DE.
Prove that AB+AC>BE.
View SolutionIn ΔABC,D is any point on side BC. Prove that
AB+BC+CA>2AD
View SolutionIn ΔABC, if D is any point on the side BC, show that (AB+BC+AC)>2AD.

View SolutionIn the given figure ,P is a point on the side BC of △ABC.Prove tat (AB+BC+AC)GT2AP
View SolutionIn triangle ABC , angle B is equal to twice of angle C , D is a point on BC such that AD bisects ∠BAC and AB=AD . Prove that ∠BAC=72∘
View SolutionIn Figure, it is given that AB=CD and AD=BC. Prove that ADC ≅CBA
View Solution
