A ray incident on the droplet of water at an angle of incidence i undergoes two reflections (not total) and emerges. If the deviation suffered by the ray within the drop is minimum and the refractive index of the droplet be μ, then show that cosi=√(u2−1)8.
The correct Answer is:A
δTotal=δRefraction+2δReflection+δRefraction
or δ=(i−r)+2(180∘−2r)+(i−r)
=360∘+2i−6r
=360∘+2i−6sin−1(siniμ)
For deviation to be minimum, dδdi=0
By putting first derivative of δ(w.r.t.i) equal to
zero, we get the desired result.
Topper's Solved these Questions
Similar Questions
A ray incident on a droplet of water from air at an angle of incidence 30∘ undergoes two reflections and emerges. If the deviation suffered by the ray is minimum then the requried refractive index of the drop μ=√x. Find the value of x.
View SolutionA ray of light is incident on the surface of water. For what angle, the ray will undergo total internal reflection ?
View Solution
Knowledge Check
A light ray is incident on a smooth reflecting surface making an angle of incidence 2i. If the angle of incidence is doubled, then the deviation of the light ray is equal to ______ .
A90-40iB90-2iC2iD180-8iA ray of light incident on a transparent block at an angle of incident 60∘. If the refractive index of the block is 1.732, the angle of deviation of the refracted ray is
A15∘B25∘C30∘D45∘A light ray is incident on a smooth reflecting surface making an angle of incidence 2i. If the angle of incidence is doubled, then the deviation of the light ray is equal to ______ .
A90−4iB90−2iC2iD180−8i
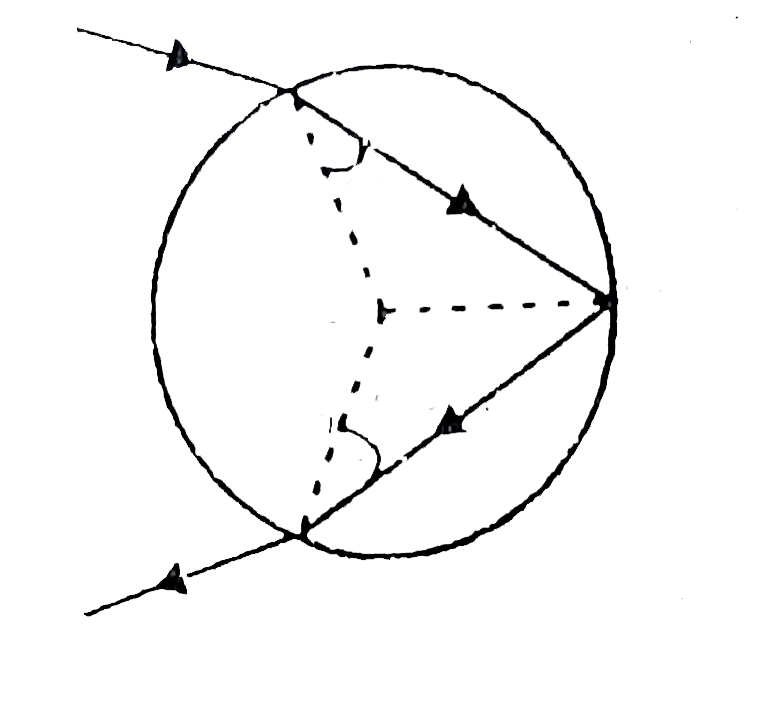
A ray is incident on a sphere of refractive index √2 as shown in figure. Angle of refraction of the ray inside sphere is 30∘ if total deviation suffered by the ray is 30x0, then find x.
View Solution(a) If the refracting angle of a prism is 60∘ and the minimum deviation is 30∘, then find the angle of incidence.
(b) A ray of light passes through an equilateral prism such that the angle of emergence is equal to the angle of incidence and each is equal to 3/4th of the angle of prism. Find the angle of deviation.
(c) The refracting of a prism is A and the refractive index of the material of the prism is cotA/2. Find the angle of deviation.
(d) The angle of a prism is 60∘. What is the angle of incidence for minimum deviation? The refractive index of the material of the prism is √2.
View SolutionA ray of light incident on a transparent sphere at an angle π/4 and refracted at an angle r, emerges from the sphere after suffering one internal reflection. The total angle of deviation of the ray is
View SolutionA ray of light is incident on a medium at an angle i. It is found that the reflected ray is at right angles to the refracted ray . The refractive index of the medium is given by
View SolutionThe angle of a prism is 60∘ and its refractive index is √2 . The angle of minimum deviation suffered by a ray of light in passing through it is
View Solution