
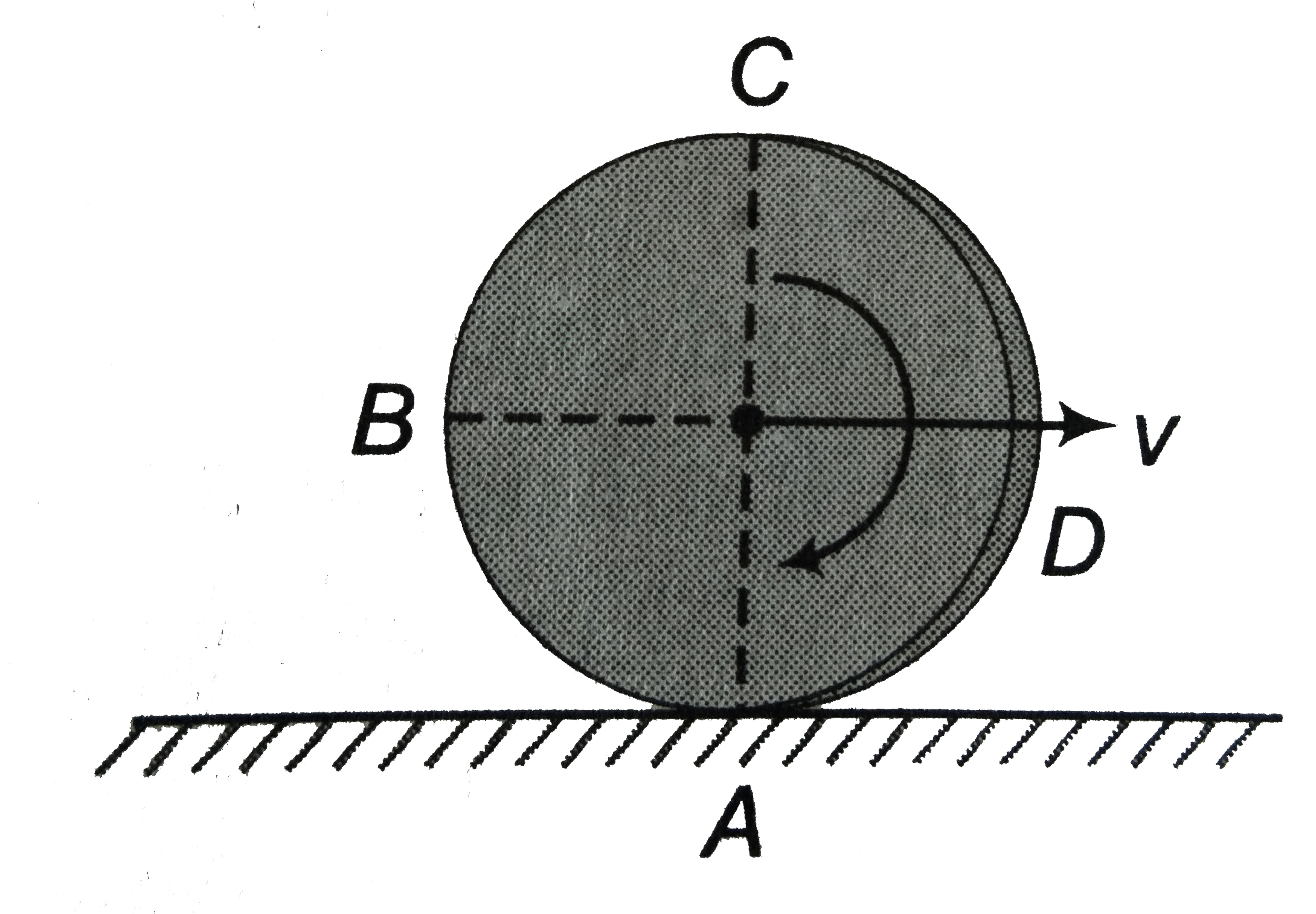
A ring of mass m is rolling without slipping with linear speed v as shown in figure. Four particles each of mass m are also attached at points A, B , C and D find total kinetic energy of the system.
Earlier we have have learned that in case of pure rolling,
ω=vR,vA=0,vB=vD=√2v and vC=2v
Now, total kinetic energy =[ tanslational kinetic energy of four particles]+[ translational kinetic energy of ring + rotational kinetic energy of ring]
∴(KE)total=[12m(0)2+12m(√2v)2+12m(√2v)2+12m(2v)2]+[12mv2+12Iω2]
Substituting I=mR2 and ω=vR
we get (KE)total=5mv2
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Miscellaneous Examples2videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Solved Example1videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions2videos
ROTATIONAL MECHANICS
- BOOK - DC PANDEYCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises39videos
ROTATION
- BOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions17videos
ROTATIONAL MOTION
Similar Questions
A disc is rolling without slipping with linear velocity v as shown in figure. With the concept of instantaneous axis of rotation, find velocities of point A, B, C and D.View SolutionA ring of mass 3kg is rolling Without Slipping with linear velocity 1ms−1 on a smooth horizontal surface. A rod of same mass is fitted along its one diameter. Find total kinetic energy of the system (in J).
View Solution
Knowledge Check
- Question 1 - Select One
A ring of mass m is rolling without slipping with linear velocity v as shown is figure. A rod of identical mass is fixed alone one of its diameter. The total kinetic energy of the system is
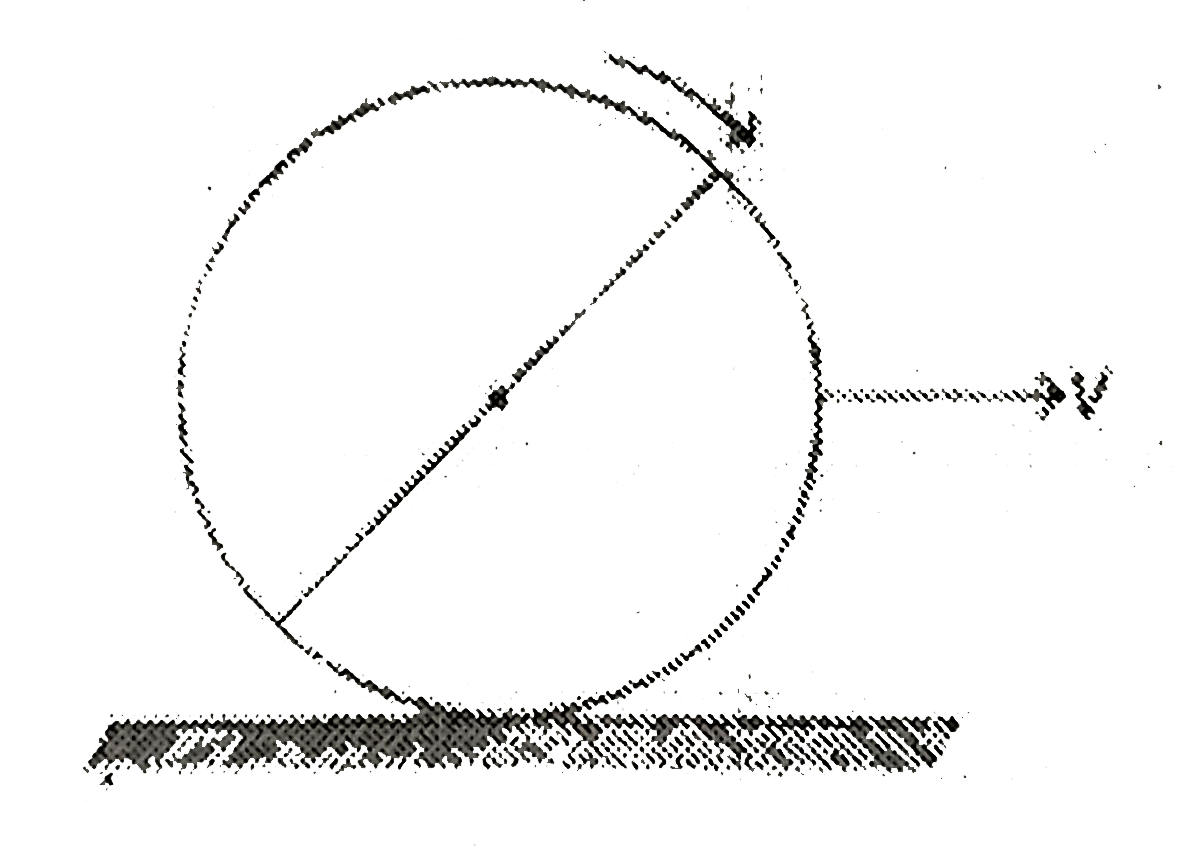 A75mv2B23mv2C43mv2D53mv2
A75mv2B23mv2C43mv2D53mv2 - Question 2 - Select One
A small sphere of mass 1 kg is rolling without slipping with linear speed
v=√2007m/s

It leaves the inclined plane at point C.
Find ratio of rotational and translational kinetic energy of the sphere when it strikes the ground after leaving from point C.A25B23C16D12 - Question 3 - Select One
A small sphere of mass 1 kg is rolling without slipping with linear speed
v=√2007m/s

It leaves the inclined plane at point C.
Find the linear speed at point C.A√1007 m/sB√507 m/sC√10035 m/sD√20035 m/s
Four particles each of mass m are kept at the four vertices of a square of side 'a' . Find graitational potential energy of this system.
View SolutionA solid sphere of mass 1 kg rolls on a table with linear speed 2 m/s, find its total kinetic energy.
View Solution
A solid sphere of mass m and radius R is rolling without slipping as shown in figure. Find angular momentum of the sphere about z-axis.View SolutionA ring and a disc roll on the horizontal surface without slipping with same linear velocity. If both have same mass and total kinetic energy of the ring is 4 J then total kinetic energy of the disc is
View SolutionA ring and a disc roll on the horizontal surface without slipping, with same linear velocity. If bolh have same mass and total kinetic energy of the ring is 4 J, then total kinetic energy of the disc is
View Solution

