A wheel of radius 10 cm can rotate freely about its centre as shown in

A wheel of radius $0.4 m$ can rotate freely about its axis as shown in the figure. A string is wrapped over its rim and a mass of $4 k g$ is hung. An angular acceleration of $8 r a d / s^{2}$ is produced in it due to the torque. Then, the moment of inertia of the wheel is ( $g = 10 m / s^{2}$ )

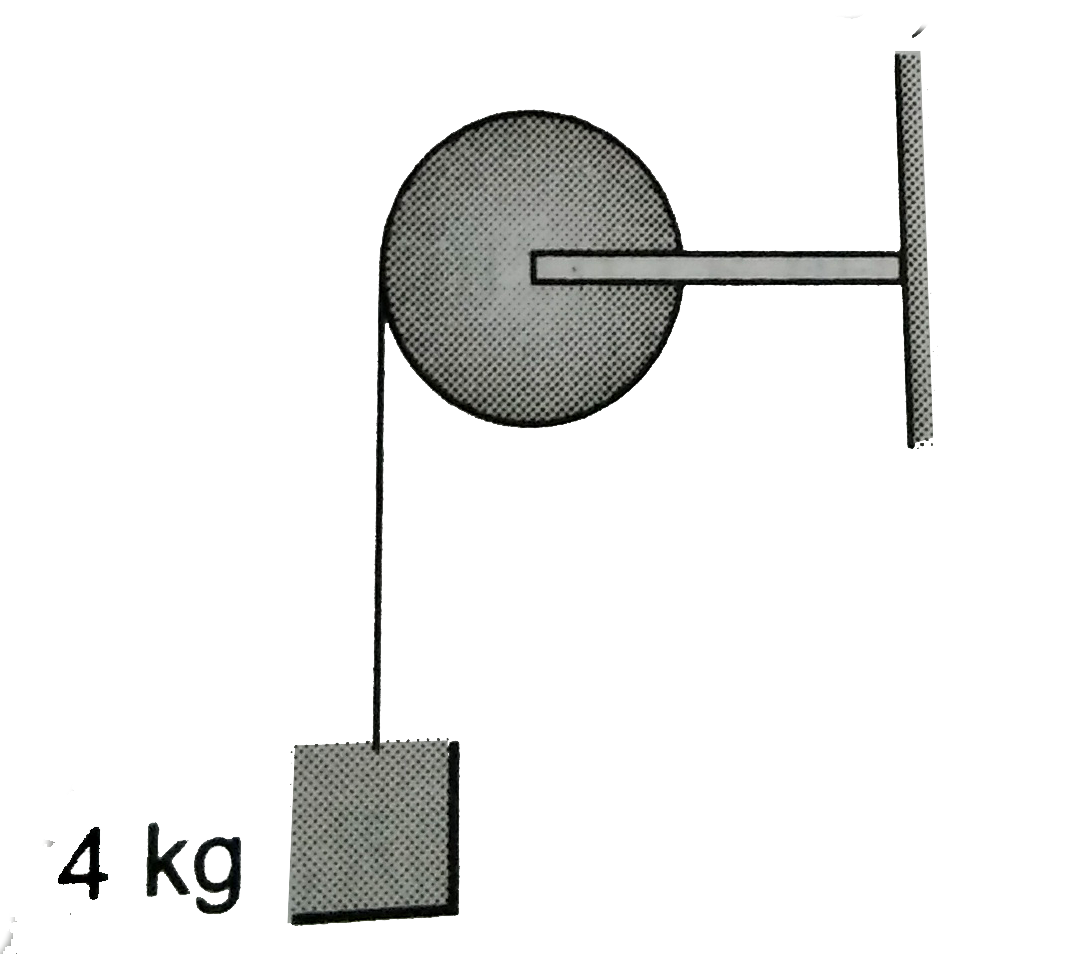A $2 k g - m^{2}$
B $1.36 k g - m^{2}$
C $4 k g - m^{2}$
D $8 k g - m^{2}$
A constant torque of 3.14 N mis exerted on a pivoted wheel. If the angular acceleration of the wheel is $4 π rad/ s^{2}$ then the moment of inertia of the wheel is
A $0.25 k g m^{2}$
B $2.5 k g m^{2}$
C $4.5 k g m^{2}$
D $25 k g m^{2}$
A wheel of radius $20 c m$ has four forces applied to it as shown in fig. Then, the torque produced by these forces about $O$ is.
.
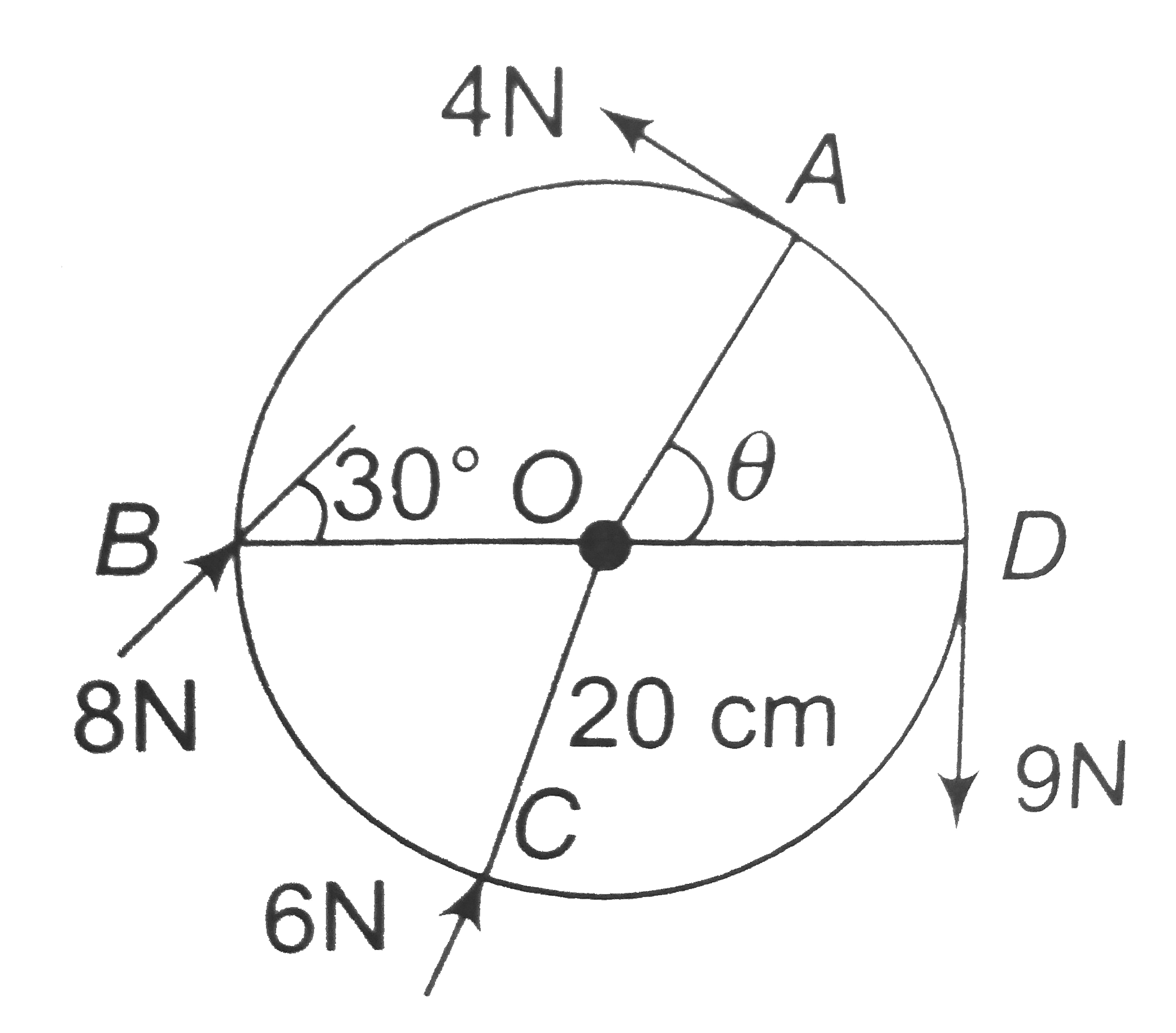A $5.4 N m$ anticlockwise
B $1.8 N m$ clockwise
C $1.8 N m$ anticlockwise
D $5.4 N m$ clockwise

A wheel of radius 10 cm can rotate freely about its centre as shown in figure. A string is wrapped over its rim and is pulled by a force of 5.0 N. It is found that the torque produces an angular acceleration 2.0 $r a \frac{d}{s^{2}}$ in the wheel. Calculate the moment of inertia of the wheel.