A particle moves along a horizontal path, such that its velocity is given by v=(3t2−6t)m/s, where t is the time in seconds. If it is initially located at the origin O, determine the distance travelled by the particle in time interval from t=0 to t=3.5s and the particle's average velocity and average speed during the same time interval.
The correct Answer is:A, B, C, D
To solve the problem step by step, we will follow these instructions:
Step 1: Determine the velocity function
The velocity of the particle is given by:
v(t)=3t2−6tm/s
Step 2: Find the time intervals when the velocity is zero
To find when the particle changes direction, we need to set the velocity function to zero:
3t2−6t=0
Factoring out 3t:
3t(t−2)=0
This gives us:
t=0ort=2
Step 3: Analyze the velocity function
- For t<0: Not applicable since time cannot be negative.
- For 0<t<2: The velocity is negative (the particle moves in the negative direction).
- For t>2: The velocity is positive (the particle moves in the positive direction).
Step 4: Calculate the displacement from t=0 to t=3.5
We will calculate the displacement in two parts:
1. From t=0 to t=2 (where the velocity is negative).
2. From t=2 to t=3.5 (where the velocity is positive).
Part 1: Displacement from t=0 to t=2
The displacement x can be calculated by integrating the velocity function:
x=∫20(3t2−6t)dt
Calculating the integral:
x=(t3−3t2)20=(23−3⋅22)−(0−0)=(8−12)=−4m
This means the particle moves 4 meters in the negative direction.
Part 2: Displacement from t=2 to t=3.5
Now, we calculate the displacement from t=2 to t=3.5:
x=∫3.52(3t2−6t)dt
Calculating the integral:
x=(t3−3t2)3.52=((3.5)3−3(3.5)2)−(23−3(22))
Calculating each part:
=(42.875−36.75)−(8−12)=6.125+4=10.125m
Step 5: Total displacement
The total displacement from t=0 to t=3.5:
Total Displacement=−4+10.125=6.125m
Step 6: Calculate the total distance traveled
The total distance traveled is the sum of the absolute values of the displacements:
Total Distance=|−4|+|10.125|=4+10.125=14.125m
Step 7: Calculate the average velocity
The average velocity is given by:
Average Velocity=Total DisplacementTotal Time=6.125m3.5s=1.75m/s
Step 8: Calculate the average speed
The average speed is given by:
Average Speed=Total DistanceTotal Time=14.125m3.5s≈4.03m/s
Final Results
- Total Distance Traveled: 14.125m
- Average Velocity: 1.75m/s
- Average Speed: 4.03m/s
The velocity of the particle is given by:
v(t)=3t2−6tm/s
To find when the particle changes direction, we need to set the velocity function to zero:
3t2−6t=0
Factoring out 3t:
3t(t−2)=0
This gives us:
t=0ort=2
- For t<0: Not applicable since time cannot be negative.
- For 0<t<2: The velocity is negative (the particle moves in the negative direction).
- For t>2: The velocity is positive (the particle moves in the positive direction).
We will calculate the displacement in two parts:
1. From t=0 to t=2 (where the velocity is negative).
2. From t=2 to t=3.5 (where the velocity is positive).
The displacement x can be calculated by integrating the velocity function:
x=∫20(3t2−6t)dt
Calculating the integral:
x=(t3−3t2)20=(23−3⋅22)−(0−0)=(8−12)=−4m
This means the particle moves 4 meters in the negative direction.
Now, we calculate the displacement from t=2 to t=3.5:
x=∫3.52(3t2−6t)dt
Calculating the integral:
x=(t3−3t2)3.52=((3.5)3−3(3.5)2)−(23−3(22))
Calculating each part:
=(42.875−36.75)−(8−12)=6.125+4=10.125m
The total displacement from t=0 to t=3.5:
Total Displacement=−4+10.125=6.125m
The total distance traveled is the sum of the absolute values of the displacements:
Total Distance=|−4|+|10.125|=4+10.125=14.125m
The average velocity is given by:
Average Velocity=Total DisplacementTotal Time=6.125m3.5s=1.75m/s
The average speed is given by:
Average Speed=Total DistanceTotal Time=14.125m3.5s≈4.03m/s
- Total Distance Traveled: 14.125m
- Average Velocity: 1.75m/s
- Average Speed: 4.03m/s
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - More Than One Correct
6 Videos
- KINEMATICSBOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - Comprehension
7 Videos
- KINEMATICSBOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - Objective
45 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
A particle in moving in a straight line such that its velocity is given by v=12t−3t2, where v is in m//s and t is in seconds. If at =0, the particle is at the origin, find the velocity at t=3s.
View SolutionVelocity-time graph of a particle moving in a straight line is shown in figure. In the time interval from t=0 to t=14s, find
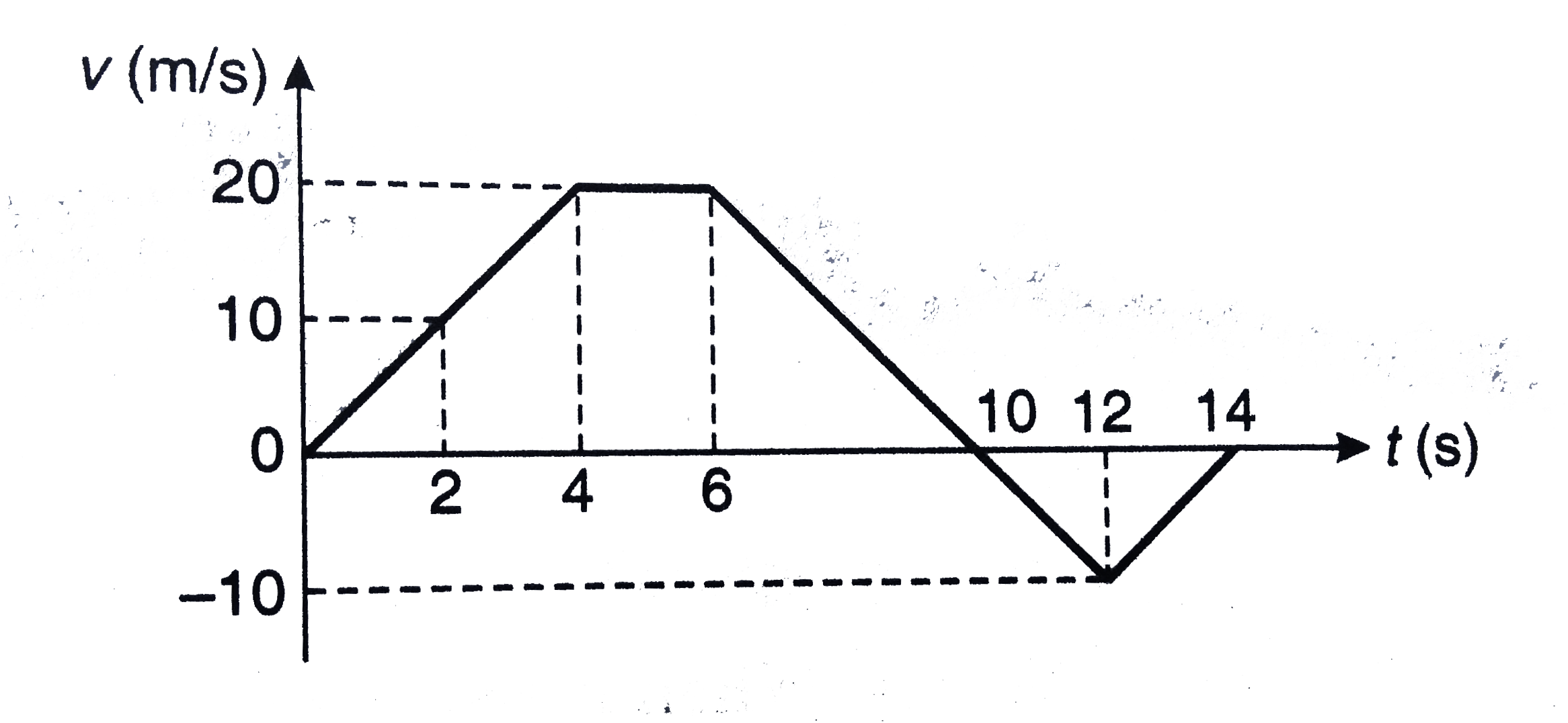
(a) average velocity and
(b) average speed of the particle.
View Solution
Knowledge Check
A particle moves along x-axis and its acceleration at any time t is a = 2 sin (πt), where t is in seconds and a is in m/s2 . The initial velocity of particle (at time t = 0) is u = 0.
Q. Then the distance travelled (in meters) by the particle from time t = 0 to t = 1 s will be :A2πB1πC4π4Dnone of theseA particle moves along x-axis and its acceleration at any time t is a = 2 sin (πt), where t is in seconds and a is in m/s2 . The initial velocity of particle (at time t = 0) is u = 0.
Q. Then the distance travelled (in meters) by the particle from time t = 0 to t = t will be :A2π2sinπt−2tπB−2π2sinπt+2tπC2tπDnone of theseThe position of a body moving along x-axis at time t is given by x=(t2−4t+6)m. The distance travelled by body in time interval t = 0 to t = 3 s is
A5 mB7 mC4 mD3 m
The speed verses time graph for a particle is shown in the figure. The distance travelled (in m) by the particle during the time interval t = 0 to t = 5 s will be __________

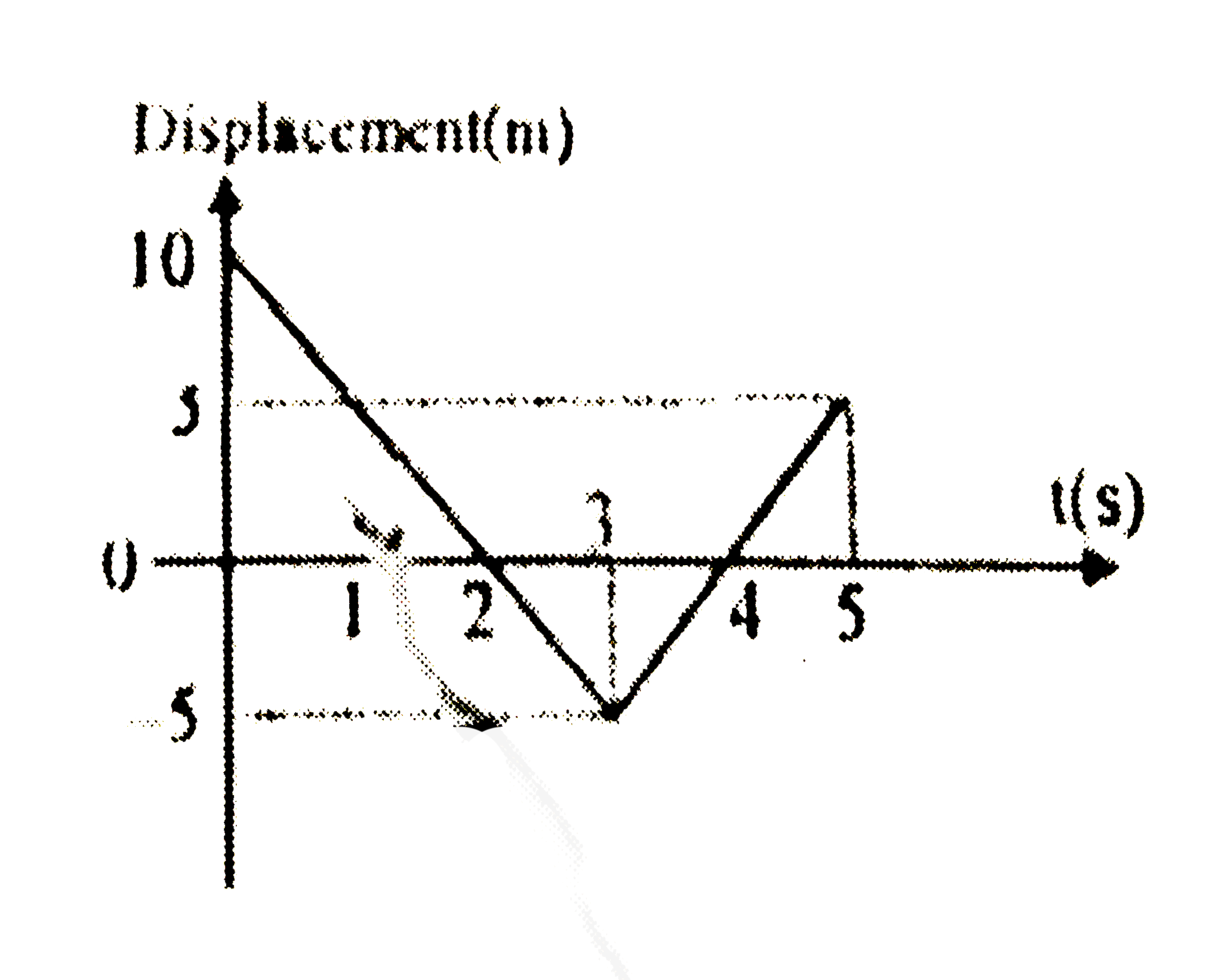
View SolutionThe diagram shows the displacement - time graph for a particle moving in a straight line. The distance (in m) coverted by the particle in the time interval t=0 to t=5s is
View SolutionA particle is moving along the x-axis such that s=6t−t2, where s in meters and t is in second. Find the displacement and distance traveled by the particle during time interval t=0 to t=5s.
View SolutionA particle moves rectilinearly with initial velocity u and constant acceleration a. Find the average velocity of the particle in a time interval from t=0 to t=t second of its motion.
View SolutionVelocity time equation of a particle moving in a straight line is v=2t−4 for t≤2s and v=4−2t for t>2.The distance travelled by the particle in the time interval from t=0 to t=4s is (Here t is in second and v in m/s)
View Solution
