Using Ampere's circuital law, obtain an expression for the magnetic induction at a point near an infinitely long straight conductor carrying an electric current.
An ideal solenoid is tightly wound and infinitely long. Let n be the number of turns of wire per unit length and I be the steady current in the solenoid.
For an ideal solenoid, the magnetic induction →B inside is reasonably uniform over the cross section and parallel to the axis throughout the volume enclosed by the solenoid, →B outside is negligible.
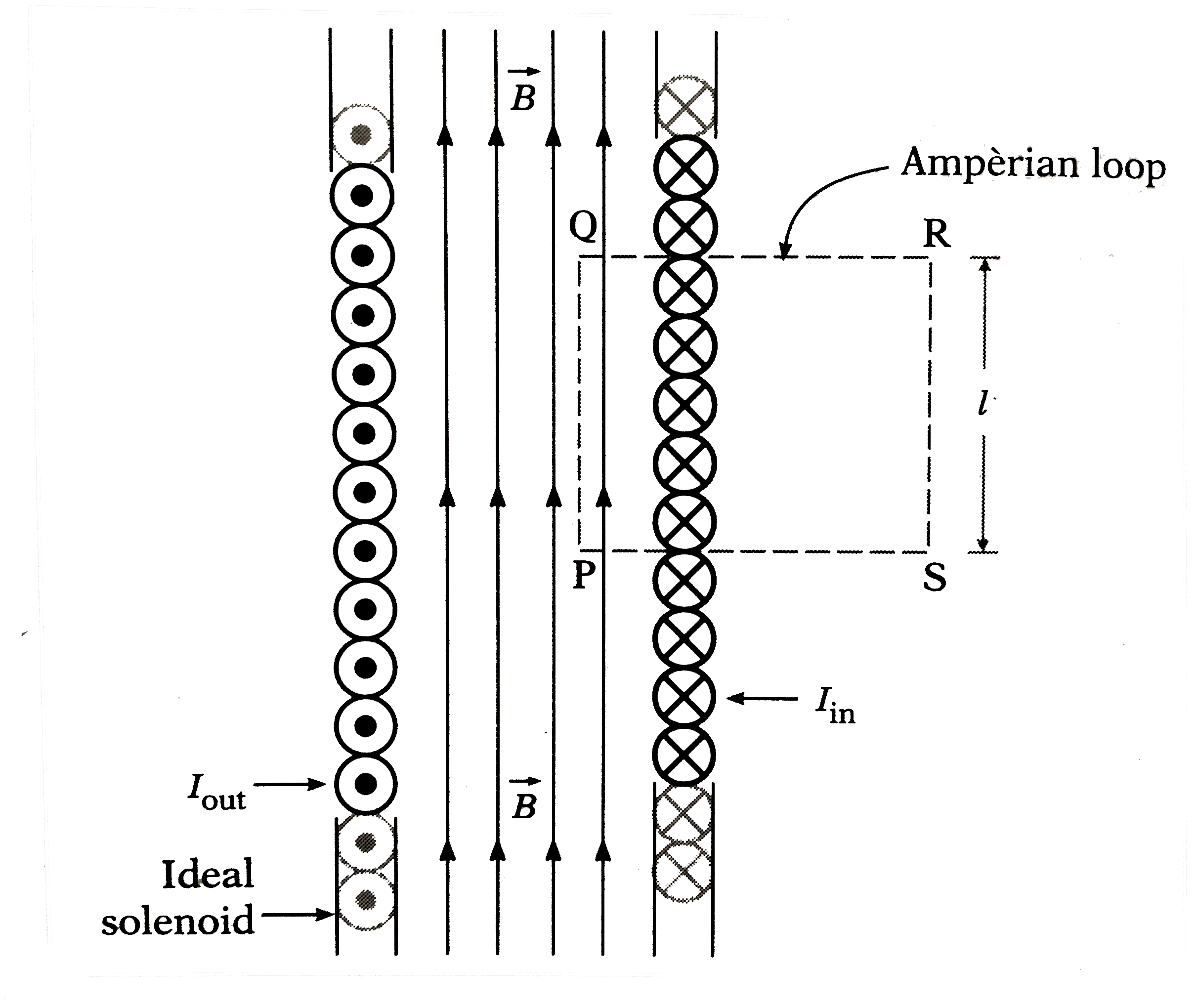
An an Amperian loop, we choose a rectangular path PQRS of length l parallel to the solenoid axis. The width of the rectangle is taken to be sufficiently large so that the side RS is far from the solenoid where →B≅0.
The line integral of the magnetic induction around the Amperian loop in sense PQRSP is
∮→B⋅→dl=Q∫P→B⋅→dl+R∫Q→B⋅→dl+S∫R→B⋅→dl+P∫S→B⋅→dl ...(1)
→B has the same magnitude inside and is parallel to side PQ. Hence, as we go in the same direction as →B from P to Q, →Band→dl are parallel so that
Q∫P→B⋅→dl=Q∫PBdl=BQ∫Pdl=Bl ...(2)
Along the paths Q→RandS→P,→B is perpendicular to →dl inside the solenoid while →B=0 outside.
∴R∫Q→B⋅→dl=P∫S→B⋅→dl=0 ...(3)
Also →B=0 along side RS, so that
S→BR⋅→dl=0 ...(4)
Thus, from Eqs. (1),(2), (3) and (4),
∮→B⋅→dl=Bl ...(5)
The net current enclosed by the Amperian loop is
Iencl= current through each turn × number of turns enclosed by the loop
=I×nl=nlI ...(6)
By Ampere's law, in free space,
∮→B⋅→dl=μ0Iencl, where μ0 is the permeability of free space.
Therefore, from Eqs. (5) and (6), Bl=μ0nlI
∴B=μ0nI ...(7)
This is the required expression.
Topper's Solved these Questions
- DERIVATIONS-IIBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:DERIVATIONS-IIExercise:Magnetism
Explore 1 Video
- DERIVATIONS-IIBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:DERIVATIONS-IIExercise:Electromagnetic Induction
Explore 6 Videos
- DERIVATIONS-IIBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:DERIVATIONS-IIExercise:Electrostatics
Explore 12 Videos
- DERIVATIONS-IBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:DERIVATIONS-IExercise:Assignments
Explore 9 Videos
- DISTINGUISH BETWEENBook:NAVNEET PUBLICATION - MAHARASHTRA BOARDChapter:DISTINGUISH BETWEENExercise:Assingment
Explore 3 Videos
Similar Questions
Knowledge Check
Magnetic field at a distance r from an infinitely long straight conductor carrying steady varies as
A1/r2B1/rC1/r2D1/√rThe magnetic induction at any point due to a long straight wire carrying a current is
AProportional to the distance from the wireBInversely proportional to the distance from wireCInversely proportional to the square of the distance from the wireDDoes not depend on distanceThe magnitude of magnetic induction at a distance 4 cm due to straight conductor carrying a current of 10 A is
A5×10−6Wb/m2B5×10−5 N/AmC5×10−5 gaussD5×10−6 tesla
Using Ampere's circuital law, obtain an expression for the magnetic induction at a point near an infinitely long straight conductor carrying an electric current.
View SolutionState Ampere's circuital law. By using it derive an expression for magnetic field intensity at a point due to a straight current carrying conductor.
View SolutionUsing Ampere's circuital law, obtain an expression for the magnetic field along the aixs of a current carrying solenoid of length l and having N number of turns.
View SolutionCalculate the magnetic field at a distance of 5 cm from an infinite straight conductor carrying a current of 10 A.
View SolutionCalculate the magnetic field at a point P. 0.5m away from an infinitely long straight wire carrying current of 90 A.
View Solution
