Find the area bounded by the curve y=2x−x2 and the straight line y=−x
A
132 sq unit
B
92 sq unit
C
72 sq unit
D
212 sq unit
Video Solution
Text Solution
The correct Answer is:B
Intersection points of the given curves are (0, 0) and (3, 3).
∴ Required area =∫30(y1−y2)dx
=∫30{2x−x2−(−x)}dx
⎡⎢⎣∵P(x,y1)andQ(x,y2) lie on y=2x−x2and y = - x respectively.∴y1=2x−x2andy2=−x⎤⎥⎦
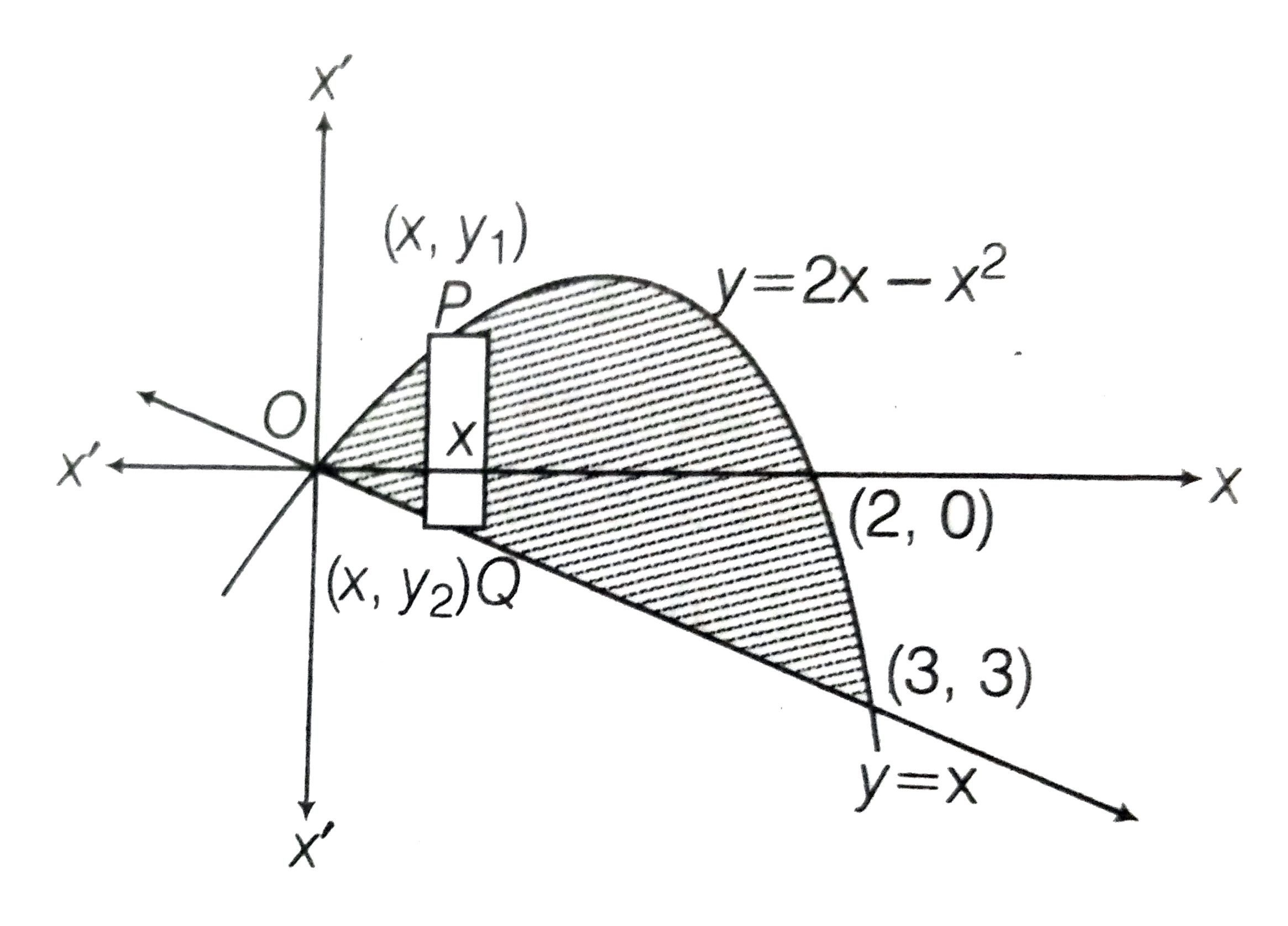
=∫30(3x−x2)dx
=[32x2−x33]30=272−273=92 sq units
|
Topper's Solved these Questions
- APPLICATIONS OF DEFINITE INTEGRALS Book:MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSChapter:APPLICATIONS OF DEFINITE INTEGRALS Exercise:MHT CET Corner
Explore 6 Videos
- APPLICATIONS OF DEFINITE INTEGRALS Book:MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSChapter:APPLICATIONS OF DEFINITE INTEGRALS Exercise:MHT CET Corner
Explore 6 Videos
- APPLICATIONS OF DERIVATIVESBook:MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSChapter:APPLICATIONS OF DERIVATIVESExercise:MHT CET CORNER
Explore 21 Videos
Similar Questions
Knowledge Check
What is the area bounded by the curves y=e^x, y=e^(-x) and the straight line x=1?
A(e+1/e )B(e−1e)C(e+1e−2)sq unitD(e−1e−2)sq unitWhat is the area bounded by the curves y=ex,y=e−x and the straight line x=1?
A(e+1e) sq. unitsB(e−1e) sq. unitC(e+1e−2) sq. unitsD(e−1e−2)sq units
For what value of 'a' is the area bounded by the curve y=a2x2+ax+1 and the straight line y=0. x=0andx=1 the least?
View SolutionFind the area enclosed by the curve y=−x2 and the straight line x+y+2=0.
View SolutionFind the area enclosed by the curve y=−x2 and the straight line x+y+2=0.
View SolutionFind the area bounded by the curve y=−3|x|+2 and x-axis
View SolutionWhat is the area bounded by the curves y=ex,y=e−x and the straight line x=1 ?
View SolutionFind the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View Solution