The area bounded by y=sin−1x,x=1√2 and X-axis is
A
(1√2+1) sq units
B
(1−1√2) sq units
C
π4√2 sq units
D
(π4√2+1√2−1) sq units
Video Solution
Text Solution
The correct Answer is:D
Required area = area of rectangle OABC - area of curve OBCO
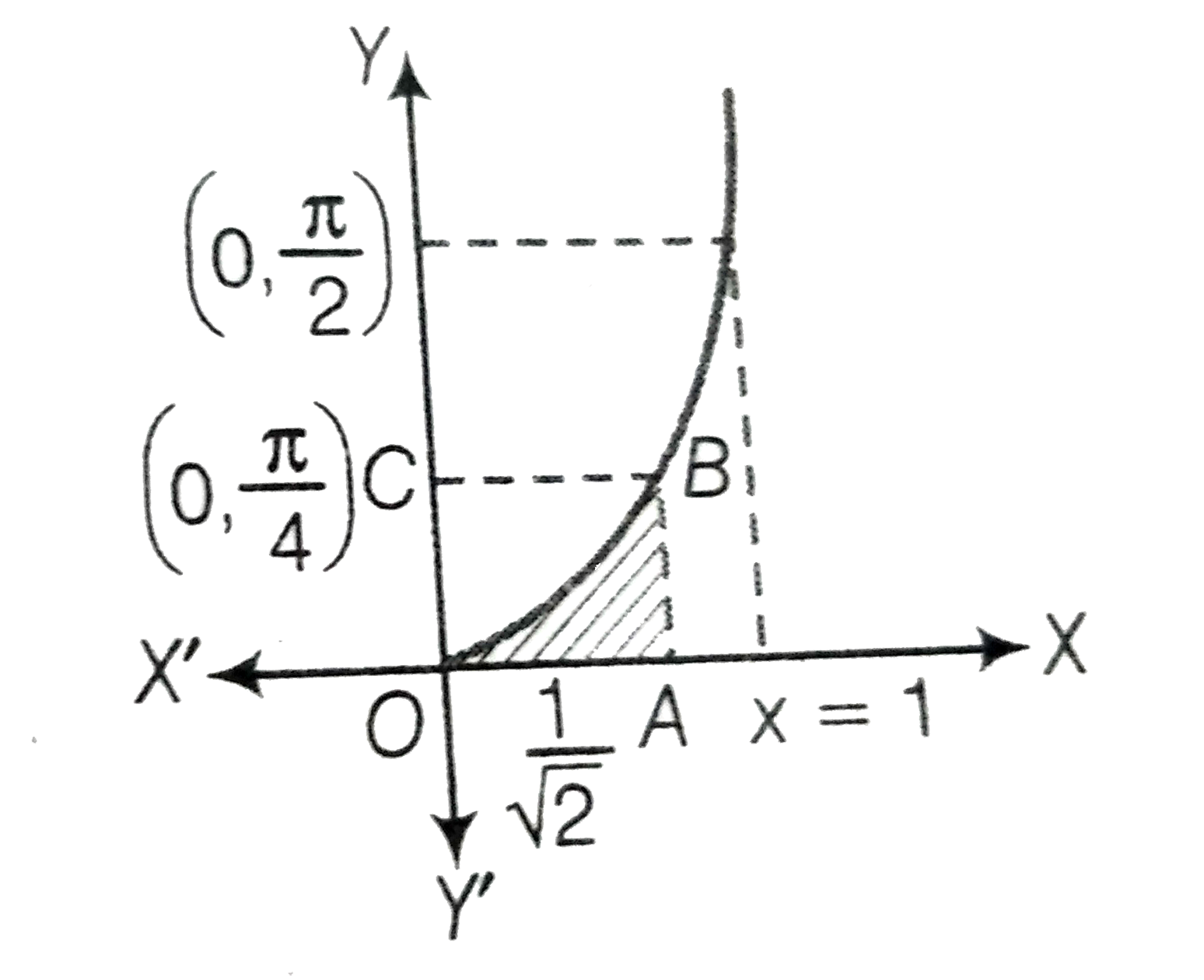
=π4√2−∫π/40sinydy=π4√2+[cosy]π/40
=[π4√2+(1√2−1)] sq unit
|
Topper's Solved these Questions
- APPLICATIONS OF DEFINITE INTEGRALS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - MHT CET Corner
6 Videos
- APPLICATIONS OF DEFINITE INTEGRALS BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - MHT CET Corner
6 Videos
- APPLICATIONS OF DERIVATIVESBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - MHT CET CORNER
21 Videos
Similar Questions
Knowledge Check
The area bounded by y=sin−1x, y-axis and |y|=π2 is
A2BπC2πD1The area bounded by the curves y=sin x, y= cos x and y-axis in 1 quadrant is -
A√2B√2+1C√2−1D√2+2The area bounded by the curve y=12x2, the X-axis and the lines x = 2 is
A13 sq unitB23 sq unitC1 sq unitD43 sq units
Find the area bounded by ysin−1(sinx) and x-axis for x∈[0,100π]
View SolutionFind the area bounded by y=sin−1(sinx) and x=aξorx∈[0,100π]
View SolutionThe area bounded by the curve y=|cosx−sinx|,0≤x≤π2 and above x-axis is
View SolutionThe area bounded by the curve y=sin2x, axis and y=1, is
View SolutionThe area bounded by y = |sin x|, X-axis and the line |x|=π is
View Solution
