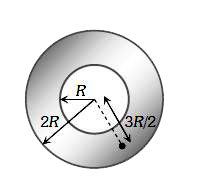
Figure shows the cross-sectional view of the hollow cylindrical conductor with inner radius 'R' and outer radius '2R'. Cylinder is carrying uniformly distributed current along it's axis. The magnetic induction at point 'P' at a distance 3R2 from the axis of the cylinder will be
Zero
5μ0i72πR
7μ0i18πR
5μ0i36πR
The correct Answer is:A
By using B=μ0i2πr(r2−a2b2−a2) , here r=3R2 a = R , b = 2 R
We get B=μ02π(3R2)×(3R2)2−R2(2R)2−R2⎫⎪
⎪
⎪⎬⎪
⎪
⎪⎭=5μ0i36πR
Aliter : For R<x<2R
Current Inet=(iπ(2R)2−R2)[π(3R2)2−(R)2π]
= i3R2[9R24−R2]
=i3R2×5R24=5i12
Apply ampere circuit law - ∮B.dl=μ0Inet⇒B×2π3R2=μ05i12=5μ0i36πR
Similar Questions
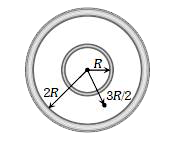
Figure shows the cross-sectional view of the hollow cylindrical conductor with inner radius 'R' and outer radius '2R', cylinder carrying uniformly distributed current along it's axis. The magnetic induction at point 'P' at a distance 3R2 from the axis of the cylinder will be
View SolutionA hollow cylindrical conductor of inner radius a and outer radius b carries a current I uniformly spread over its cross-section. Find the magnetic field induction at a point inside the body of the conductor at a distance r [where a<r<b] from the axis of the cylinder.
View Solution
Knowledge Check
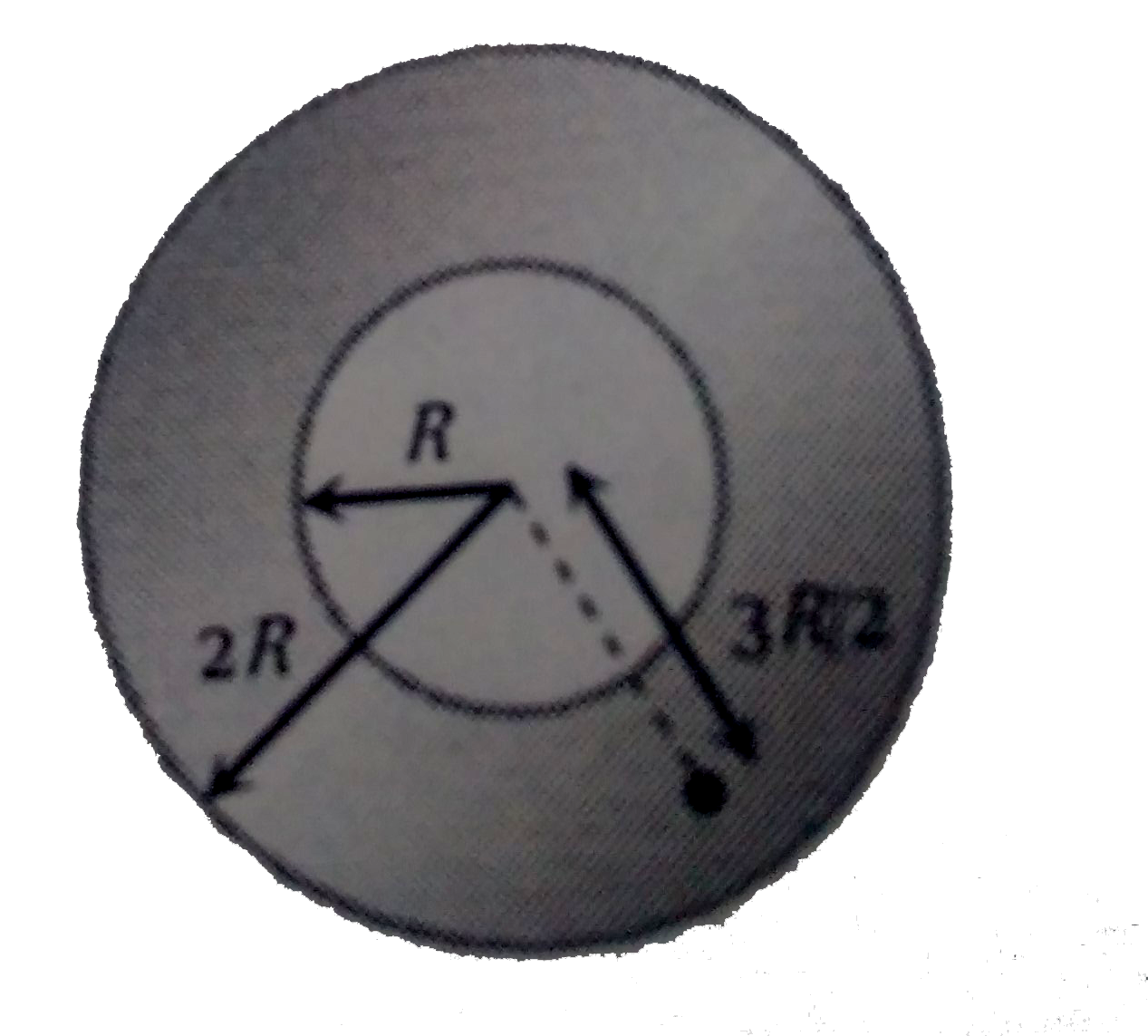
Figure shows the cross-sectional view of the hollow cylindrical conductor with inner radius 'R' and outer radius '2R', cylinder carrying uniformly distributed current along it's axis. The magnetic induction at point 'P' at a distance 3R2 from the axis of the cylinder will be
 AZeroB5μ0i72πRC7μ0i18πRD5μ0i36πR
AZeroB5μ0i72πRC7μ0i18πRD5μ0i36πRAn infinitely long hollow conducting cylinder with inner radius r2 and outer radius R carries a uniform current ra density along its length . The magnitude of the magnetic field , ∣∣→B∣∣ as a function of the radial distance r from the axis is best represented by
A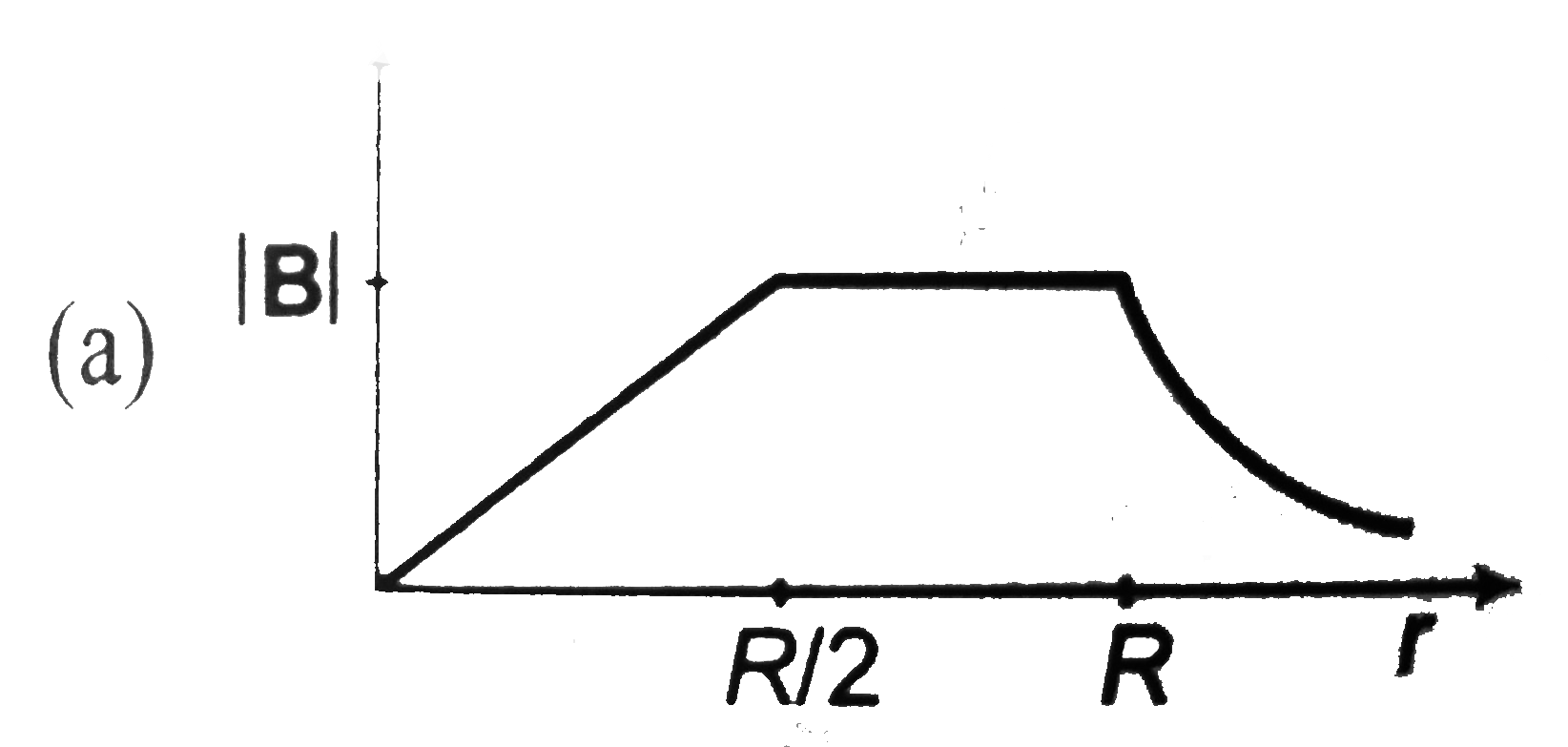 B
B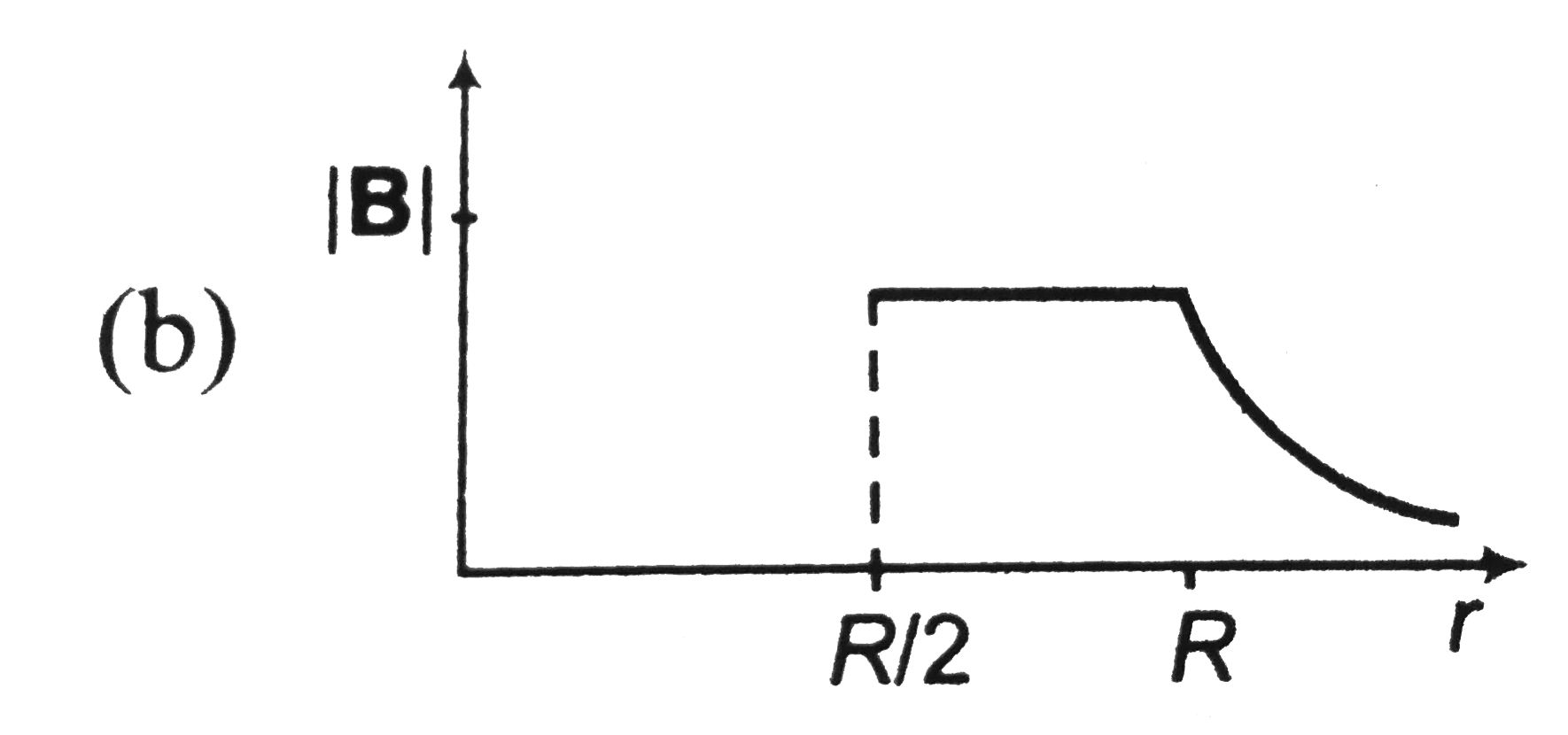 C
C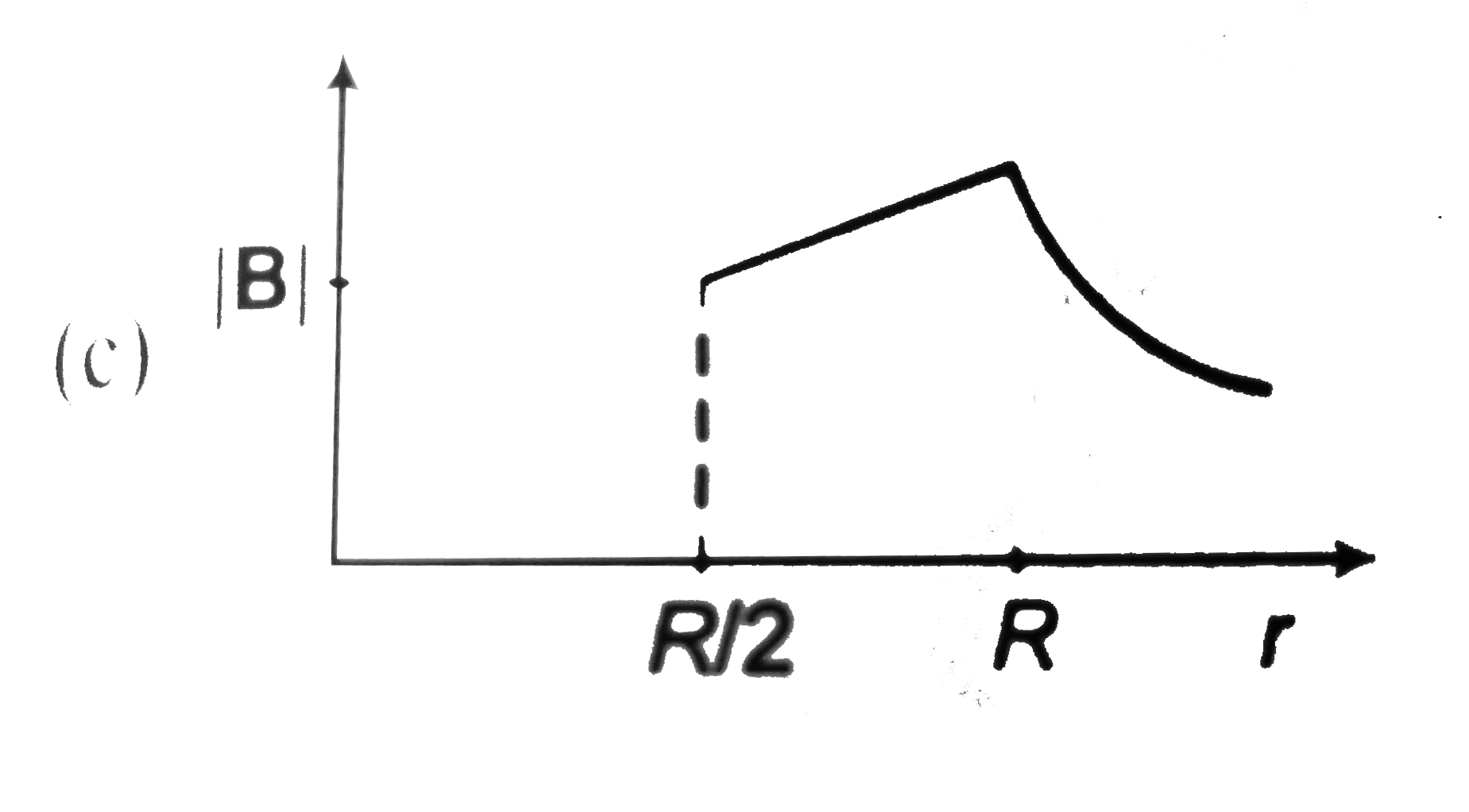 D
D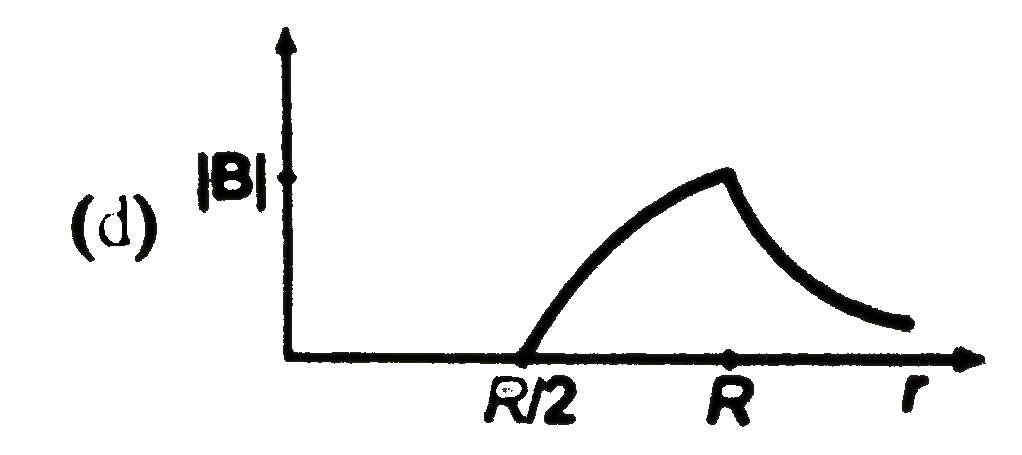
Axis of a solid cylinder of infinite length and radius R lies along y-axis. It carries a uniformly distributed current I along +y direction. Magnetic field at a point (R/2,y,R/2) is
Aμ0I4πR(ˆi−ˆk)Bμ0I2πR(ˆj−ˆk)Cμ0I4πRˆjDμ0I4πR(ˆi+ˆk)
A solid cylinder carries current of 1 A uniformly distributed over its cross sectional area. Calculate the magnetic field induction at point P, at a distance of 3 cm from the axis of cylinder, if its radius is 7 cm .
View SolutionThe figure shows the cross-section of a long cylindrical conductor of radius a carrying a uniformly distributed current i. The magnetic field due to current at P is :
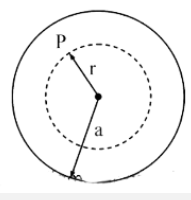
View SolutionThe figure shows the cross-section of a long cylindrical conductor of radius a carrying a uniformly distributed current i. The magnetic field due to current at P is :

View SolutionA long straight solid metal rod of radius 4cm carries a current 2A, uniformly distributed over its circular cross-section. Find the magnetic field induction at a distance 3cm from the axis of the wire.
View SolutionAxis of a solid cylinder of infinite length and radius R lies along y-axis. It carries a uniformly distributed current I along +y direction. Magnetic field at a point (R/2,y,R/2) is
View Solution
