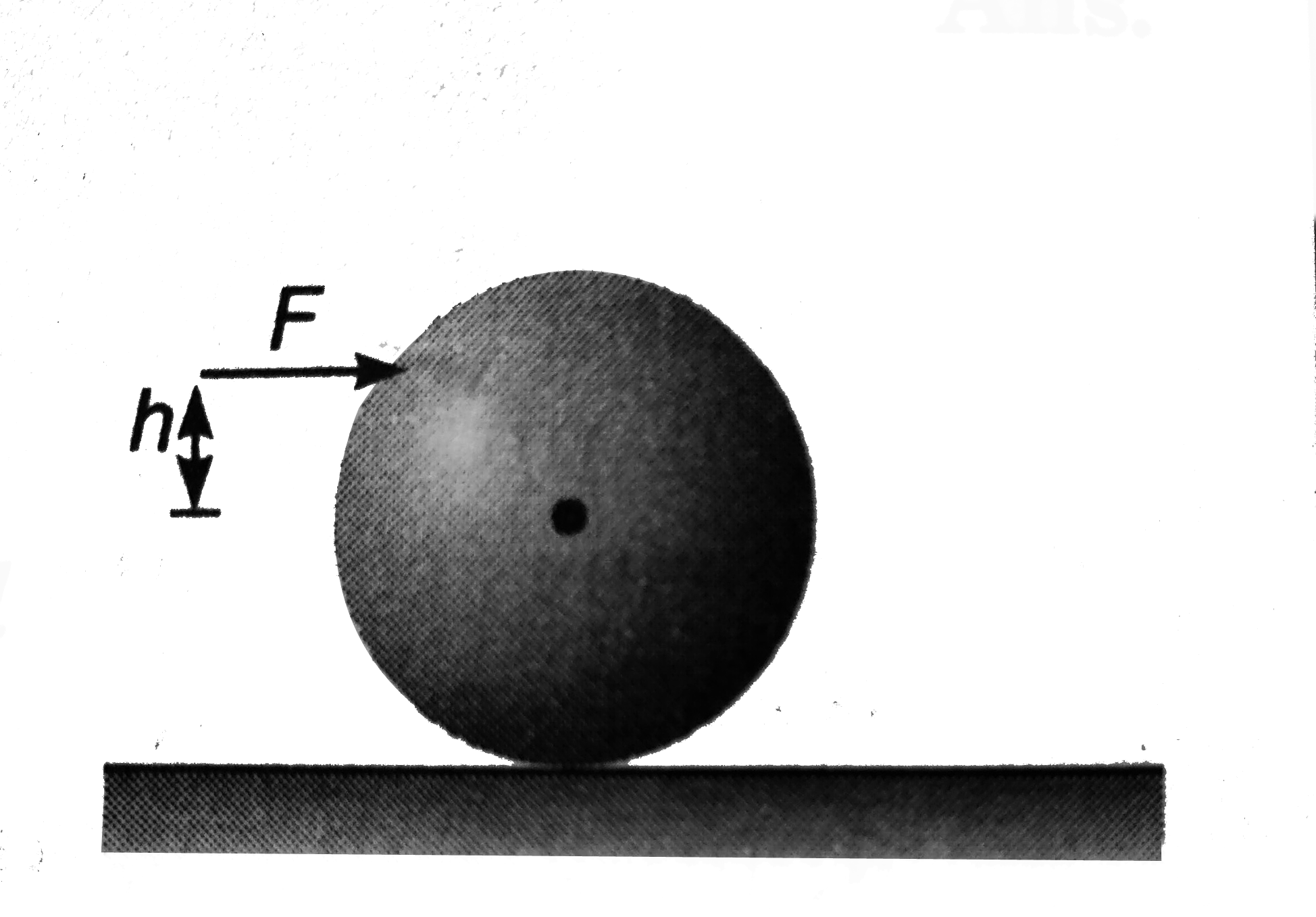
A billiard ball, initially at rest, is given a sharp impulse by a cue. The cue is held horizontally a distance h above the centre line as shown in figure. The ball leaves the cue with a speed v0 and because of its backward slipping eventually acquires a final
speed 97v0 show that h=45R
Where R is the radius of the ball.
The correct Answer is:0.8
Static frictional force acts in the forward direction because the point of contact has a tendency to slip backward. Due to frictional force, the linear velocity gradually decreases and the angular velocity increases till the ball starts rolling without sliding.
μmg=maC.M.⇒aC.M.=μg
∴vC.M.=v∘−aC.M.t=v∘−μgt
Considering the rotational motion about c.m.
τ=μmgR=(12mR2)α⇒α=5μg2R
Using ω=ω~+αt⇒ω=0+5μg2Rt=5μgt2R
When the ball rolls without sliding
vC.M.=ωR or vC.M.=52μgt
⇒vC.M.=v!−μg.25vC.M.μg or vC.M.=57v∘
At this stage (when there is rolling without slipping) , the point of contact is the instantaneous point of rotation. The velocity of a point at a distance h above the central line is vC.M.+ωh. This is (9/7)v∘.
∴vC.M.+ωh=97v∘ or vC.M.+vC.M.Rh=97v∘ (∴ω=vC.M.R)
⇒vC.M.(1+hR)=97v∘⇒57v∘(1+hR)=97v0
∴5+5hR=9 or h=45R=0.8R.
Similar Questions
In order to get a billiard ball to roll without sliding from the start, where should the cue hit ? Calculate the height from centre of the sphere. Take R as the radius of sphere.
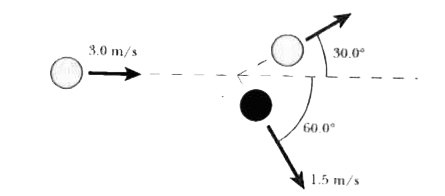
View SolutionIn the game of billiards, all the balls have approximately the same mass, about 0.17 kg. In the figure, the cue ball strikes another ball such that it follows the path shown. The other ball has a speed of 1.5 m/s immediately after the collision. What is the speed of the cue ball after the collision?
View Solutionद्रव्यमान की एक विलियर्ड गेंद जिसकी त्रिज्या r है। एक क्षैतिज दिशा में केन्द्र से h ऊँचाई पर एक क्यू (लकड़ी) द्वारा हिट की जाती है तब उसका रेखीय वेग v0 प्राप्त होता है। गेंद द्वारा प्राप्त किया गया कोणीय वेग , होगा
View SolutionA billiard ball of mass m and radius 7, when hit in a horizontal direction by a cue at a height h above its centre, acquired a linear velocity v0. The angular velocity Wo acquired by the ball is
View SolutionA solid sphere of mass M and radius R is hit by a cue at a height h above the centre C. for what value of h the sphere will rool without slipping ?
View Solution
A billiard ball, initially at rest, is given a sharp impulse by a cue. The cue is held horizontally a distance h above the centre line as shown in figure. The ball leaves the cue with a speed v0 and because of its forward english (backward slipping) eventually acquires a final
speed 97v0 show that h=45R
Where R is the radius of the ball.
View SolutionA billiard ball of mass m and radius r, when hit in a horizontal direction by a cue at a height h above its centre, acquired a linear velocity v_(0). The angular velocity omega_(0) acquired by the ball is
View SolutionThe cue stick hits a cue ball horizontally a distance x above the centre of the ball. Find the value of x for which the cue ball will instantaneously roll without slipping. Calculate the answer in terms of the radius R of the ball.
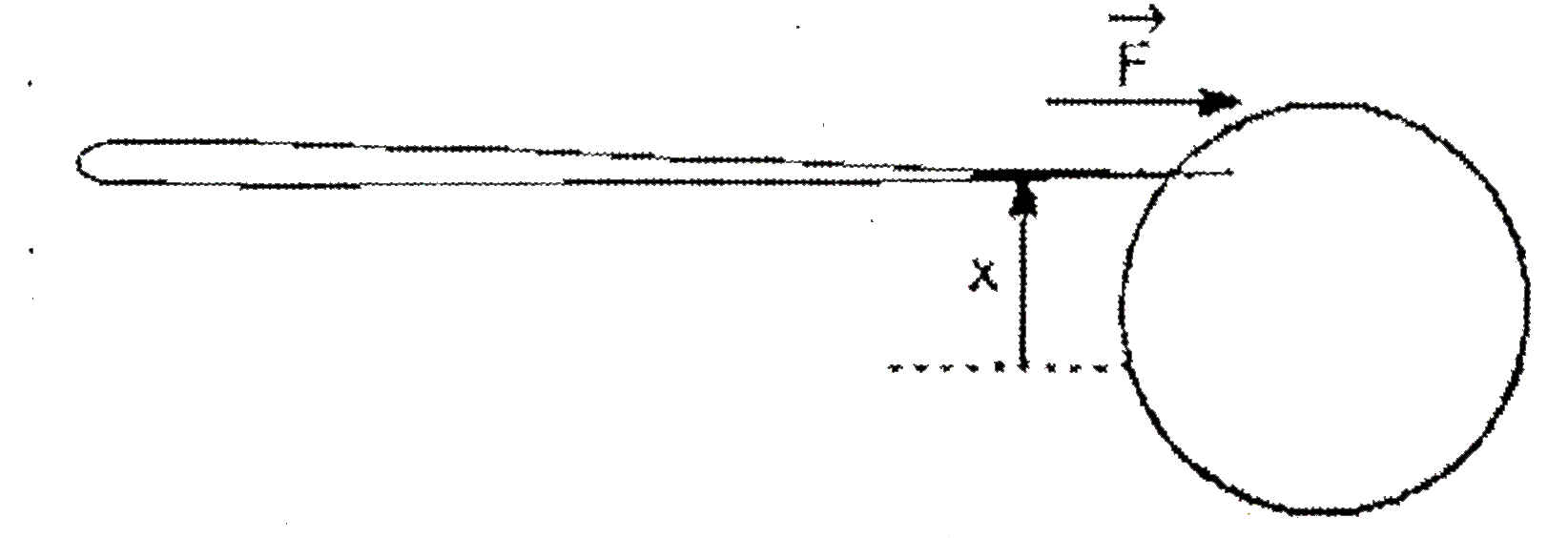
View Solution

