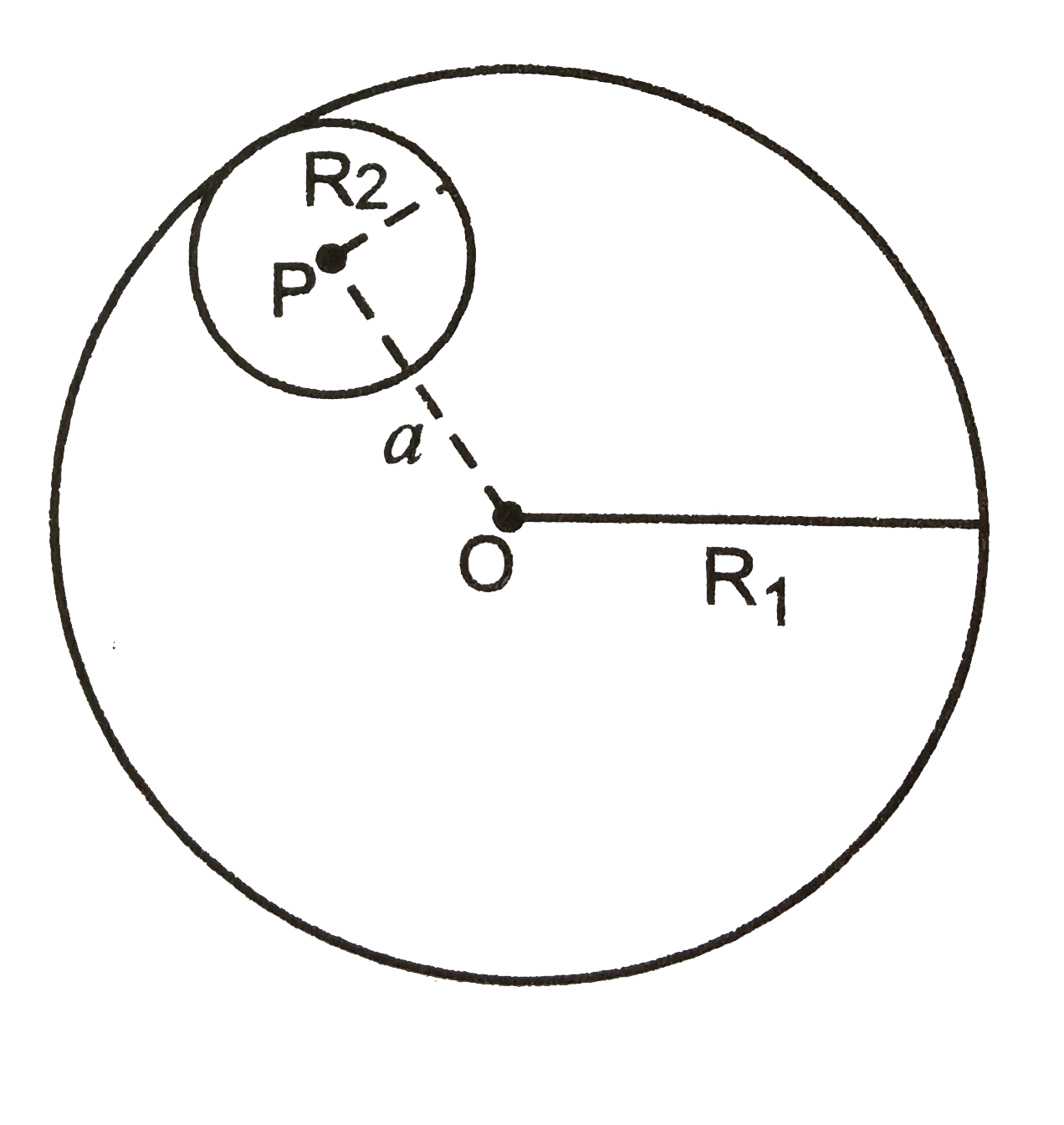
Consider a uniform spherical charge distribution of radius R1 centred at the origin O. In this distribution a spherical cavity of radius R2, centred at P with distance OP=a=R1−R2 (fig) is made.If the electric field inside the cavity at position →r, then the correct statement is
→E is uniform, its magnitude is independent of R2 but its direction depends on →r
→E is uniform, its magnitude independs of R2 and its direction depends on →r
→E is uniform, its magnitude is independent of a but its direction depends on →a
→E is uniform, and both its magnitude and direction depends on →a
The correct Answer is:D
→E=ρ(−−→OP)3ε0=ρ→a3ε0
E is uniform and both its magnitude and direction depends on →a.
Similar Questions
A charge is uniformly distributed inside a spherical body of radius r1=2r0 having a concetric cavity of radius r2=r0 (ρ is charge density inside the sphere). The potential of a point P at a distance 3r02 from the centre is
View SolutionA conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
Electric potential at the centre of the cavity will be :
View SolutionA conducting sphere of radius R_(2) has a spherical cavity of radius R_(1) which is non concentric with the sphere. A point charge q_(1) is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions. Electric field (magnitude only) at the center of the cavity will be :
View SolutionA conducting sphere of radius R_(2) has a spherical cavity of radius R_(1) which is non concentric with the sphere. A point charge q_(1) is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions. If q_(1) is shifted to the centre of the cavity, then: (choose the correct alternative)
View SolutionA metallic spherical shell has an inner radius R_(1) and outer radius R_(2) . A charge is placed at the centre of the spherical cavity. The surface charge density on the inner surface is
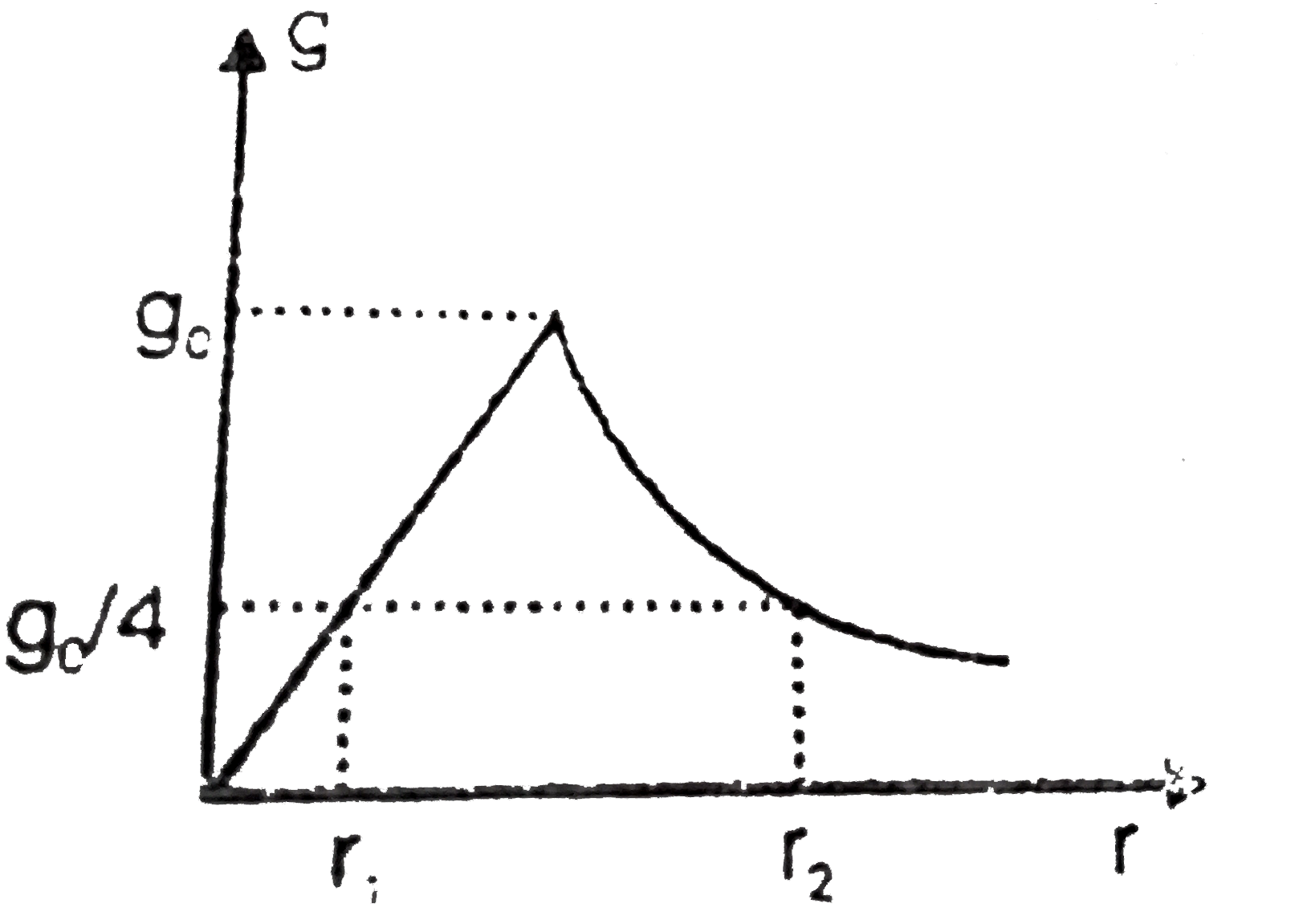
View SolutionFigure shows variation of acceleration due to gravity with distance from centre of a uniform spherical planet, Radius of planet is R. What is r1−r1
View SolutionTwo concentric spherical shells of radius R1 and R2(R2>R1) are having uniformly distributed charges Q1 and Q2 respectively. Find out total energy of the system.

View SolutionA conducting sphere of radius R_(2) has a spherical cavity of radius R_(1) which is non concentric with the sphere. A point charge q_(1) is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions. Electric potential at the centre of the cavity will be :
View Solution
