A conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
If q1 is shifted to the centre of the cavity, then: (choose the correct alternative)
electric field in the region outside sphere may change
electric potential in the region outside sphere may change
electrostatic energy stored in the system may change
electric potential of sphere may change
The correct Answer is:C
To solve the problem, we need to analyze the situation step by step, focusing on the effects of moving the point charge q1 to the center of the cavity within a conducting sphere.
1. Understanding the Setup:
- We have a conducting sphere of radius R2 with a spherical cavity of radius R1.
- A point charge q1 is initially placed at a distance r from the center of the cavity.
2. Initial Charge Distribution:
- When the charge q1 is placed at distance r from the center of the cavity, it induces a charge on the inner surface of the cavity. The induced charge will be negative and equal to −q1.
- Consequently, a positive charge +q1 will appear on the outer surface of the conducting sphere to maintain overall neutrality.
3. Moving q1 to the Center:
- When q1 is moved to the center of the cavity, the charge distribution on the inner surface of the cavity will still be −q1 because the charge q1 remains the same.
- The outer surface will still have a charge of +q1.
4. Electric Field Inside the Cavity:
- The electric field inside a conductor is zero. Therefore, the electric field in the conducting material remains zero.
- The electric field inside the cavity (where q1 is now at the center) can be calculated using Gauss's law. The electric field will be uniform and directed outward from the charge q1.
5. Electric Field Outside the Sphere:
- The electric field outside the conducting sphere is determined only by the total charge on the outer surface, which remains +q1.
- Therefore, the electric field in the region outside the sphere does not change when q1 is moved to the center.
6. Electric Potential:
- The electric potential inside the cavity will change because the distance from the point charge q1 to any point in the cavity changes.
- The potential at any point in the cavity when q1 is at the center will be different from when it was at distance r.
7. Electrostatic Energy:
- The electrostatic energy stored in the system may change due to the change in potential distribution when q1 is moved to the center.
Conclusion:
Based on the analysis, the correct alternatives regarding the effects of moving q1 to the center of the cavity are:
- The electric field in the region outside the sphere does not change.
- The electric potential in the cavity changes.
- The electrostatic energy stored in the system may change.
- We have a conducting sphere of radius R2 with a spherical cavity of radius R1.
- A point charge q1 is initially placed at a distance r from the center of the cavity.
- When the charge q1 is placed at distance r from the center of the cavity, it induces a charge on the inner surface of the cavity. The induced charge will be negative and equal to −q1.
- Consequently, a positive charge +q1 will appear on the outer surface of the conducting sphere to maintain overall neutrality.
- When q1 is moved to the center of the cavity, the charge distribution on the inner surface of the cavity will still be −q1 because the charge q1 remains the same.
- The outer surface will still have a charge of +q1.
- The electric field inside a conductor is zero. Therefore, the electric field in the conducting material remains zero.
- The electric field inside the cavity (where q1 is now at the center) can be calculated using Gauss's law. The electric field will be uniform and directed outward from the charge q1.
- The electric field outside the conducting sphere is determined only by the total charge on the outer surface, which remains +q1.
- Therefore, the electric field in the region outside the sphere does not change when q1 is moved to the center.
- The electric potential inside the cavity will change because the distance from the point charge q1 to any point in the cavity changes.
- The potential at any point in the cavity when q1 is at the center will be different from when it was at distance r.
- The electrostatic energy stored in the system may change due to the change in potential distribution when q1 is moved to the center.
Based on the analysis, the correct alternatives regarding the effects of moving q1 to the center of the cavity are:
- The electric field in the region outside the sphere does not change.
- The electric potential in the cavity changes.
- The electrostatic energy stored in the system may change.
Similar Questions
A sphere of radius 2R and mas M has a spherical cavity of radius R as shown in the figure. Find the value of gravitational field at a point P at a distance of 6R from centre of the sphere.

View SolutionA non-conducting sphere of radius R has a spherical cavity of radius R/2 as shown. The solid part of the sphere has a uniform volume charge density ρ. Find the magnitude and direction of electric field at point (a) O and (b) A.

View Solution
Knowledge Check
A conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
Electric potential at the centre of the cavity will be :AzeroBkq1r−kq1R1Ckq1r−kq1R1+Kq1R2DNone of theseA conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
Electric field (magnitude only) at the center of the cavity will be :AzeroBKq1r2CKq1r2−Kq1R21DNone of theseThere is a conductor with cavity of radius R as shown in figure. A point charge Q is placed at R/2 distance from centre of cavity.
A point P is at r distance from centre of conductor. Then,
 Acharge density at iner surface of cavity will be non uniformBcharge density at surface of conductor will be uniformCelectric field at point P is Q4πε0r2Dforce on charge Q is non zero
Acharge density at iner surface of cavity will be non uniformBcharge density at surface of conductor will be uniformCelectric field at point P is Q4πε0r2Dforce on charge Q is non zero
A solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distance R/4 from the centre of sphere. The gravitational force on mass m is
View SolutionA conducting sphere of radius b has a spherical cavity with its centre displaced by 'a' from centre of sphere O1. A point charged q is placed at the centre of cavity O2. Q charge is given to conducting sphere and charge q0 is placed at P, a distance c from centre O1. Further O1,O2 and P are collinear

View SolutionThe potential at a distance R/2 from the centre of a conducting sphere of radius R will be
View SolutionA spherical conductor A contains two spherical cavities as shown in Fig.2.127. The total charge on conductor itself is zero . However , there is a point charge q1 at the center of one cavity and q2 at the center of the other cavity . Another charge q3 is placed at a large distance r from the center of the spherical conductor.
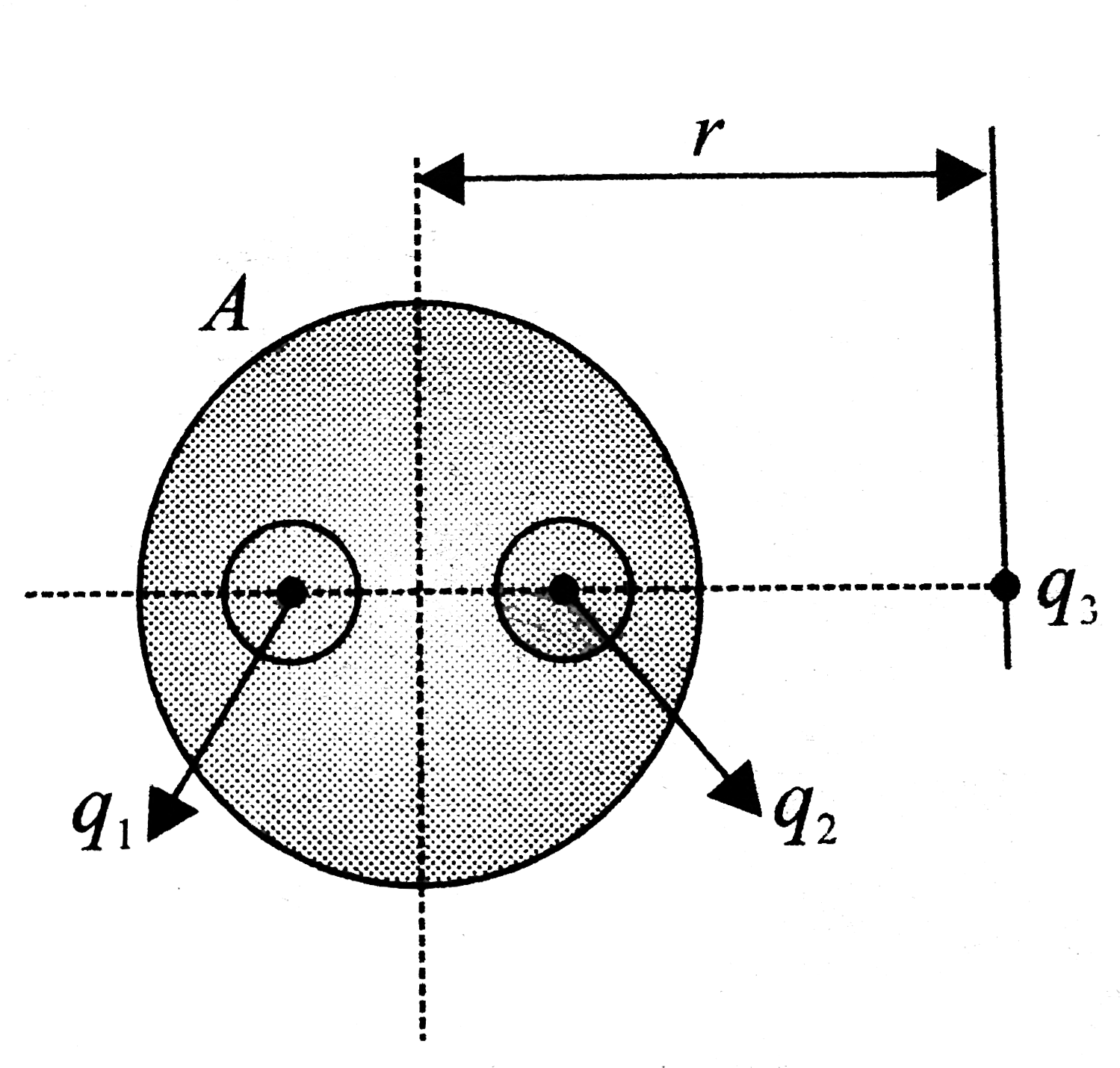
If q1 is displaced from its center slightly (being always inside the same cavity ), then the correct representation of field lines inside the same cavity is
View SolutionA solid spherical conductor of radius R has a spherical cavity of radius at its centre. A charge is kept at the centre of the cavity. The charge at the inner surface, outer surface are respectively.
View Solution
