A spherical conductor $A$ contains two spherical cavities as shown in Fig.2.127. The total charge on conductor itself is zero . However , there is a point charge $q_{1}$ at the center of one cavity and $q_{2}$ at the center of the other cavity . Another charge $q_{3}$ is placed at a large distance $r$ from the center of the spherical conductor.

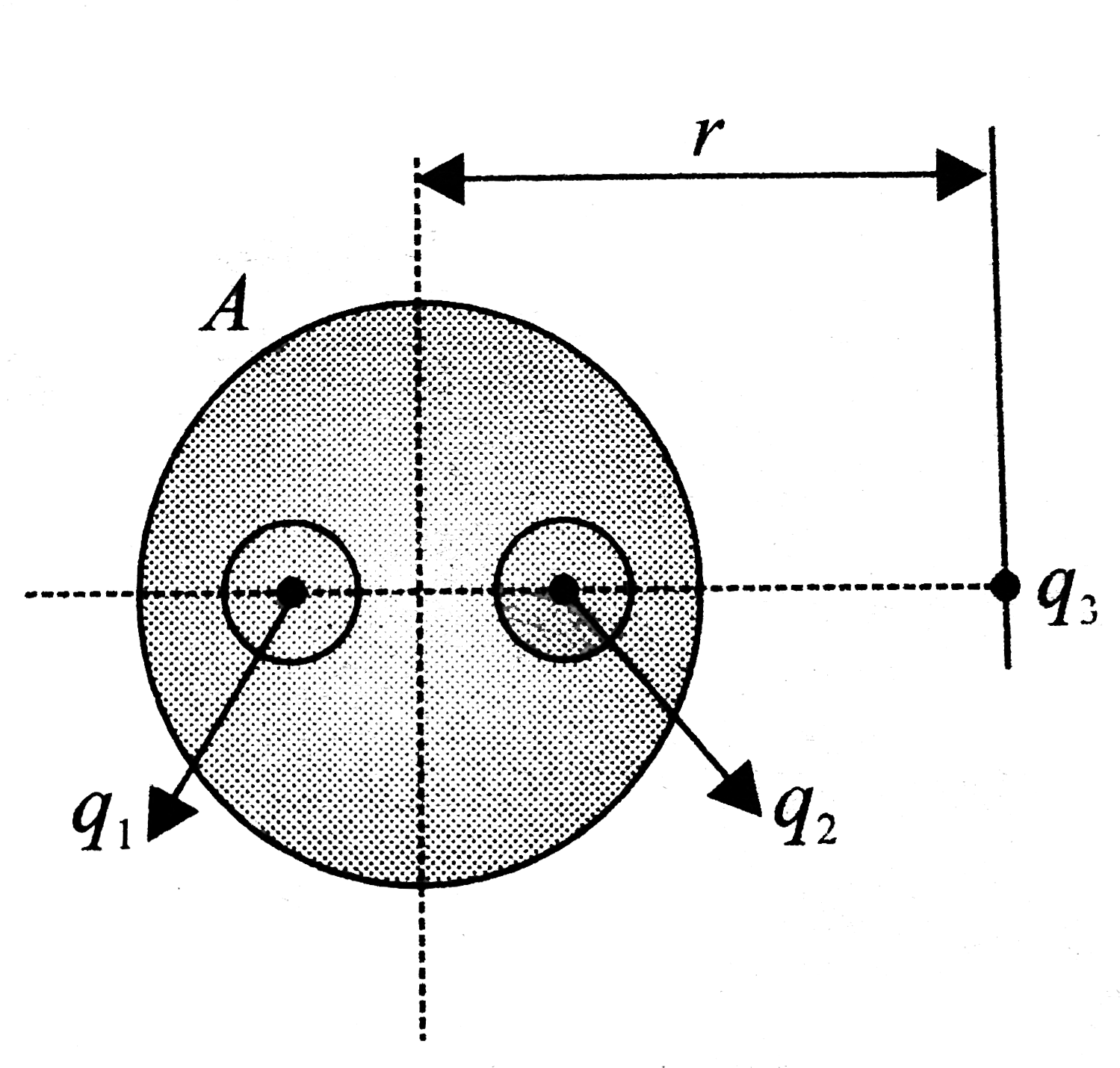
If $q_{1}$ is displaced from its center slightly (being always inside the same cavity ), then the correct representation of field lines inside the same cavity is

ocr_image

There will be no field lines inside the cavity.

ocr_image

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Thereis charge inside cavity , so field lines will be present , but the potential at surface of conductor is same everywhere . So field lines should be perpendicular .

Updated on:21/07/2023

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
A spherical conductor $A$ contains two spherical cavities as shown in Fig.2.127. The total charge on conductor itself is zero . However , there is a point charge $q_{1}$ at the center of one cavity and $q_{2}$ at the center of the other cavity . Another charge $q_{3}$ is placed at a large distance $r$ from the center of the spherical conductor.

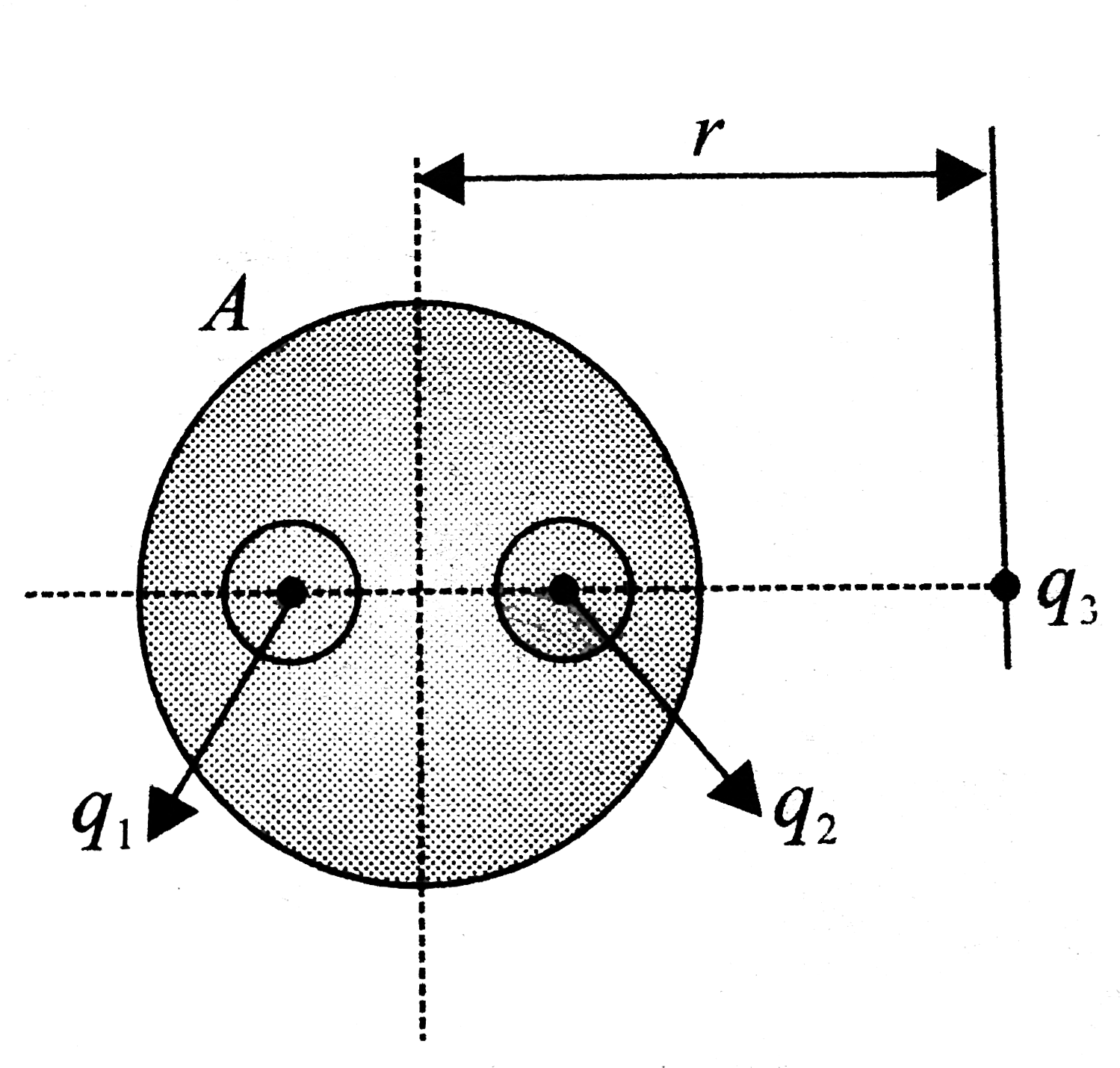
The force acting on conductor $A$ will be
Azero
B $\frac{q_{3} (q_{1} + q_{2})}{4 π ε_{0} r^{2}}$
C $\frac{- q_{3} (q_{1} + q_{2})}{4 π ε_{0} r^{2}}$
D $\frac{q_{3} q_{1} + q_{2} q_{3} + q_{1} q_{2}}{4 π ε_{0} r^{2}}$
Question 2 - Select One
A spherical conductor $A$ contains two spherical cavities as shown in Fig. The total charge on conductor itself is zero . However , there is a point charge $q_{1}$ at the center of one cavity and $q_{2}$ at the center of the other cavity . Another charge $q_{3}$ is placed at a large distance $r$ from the center of the spherical conductor.

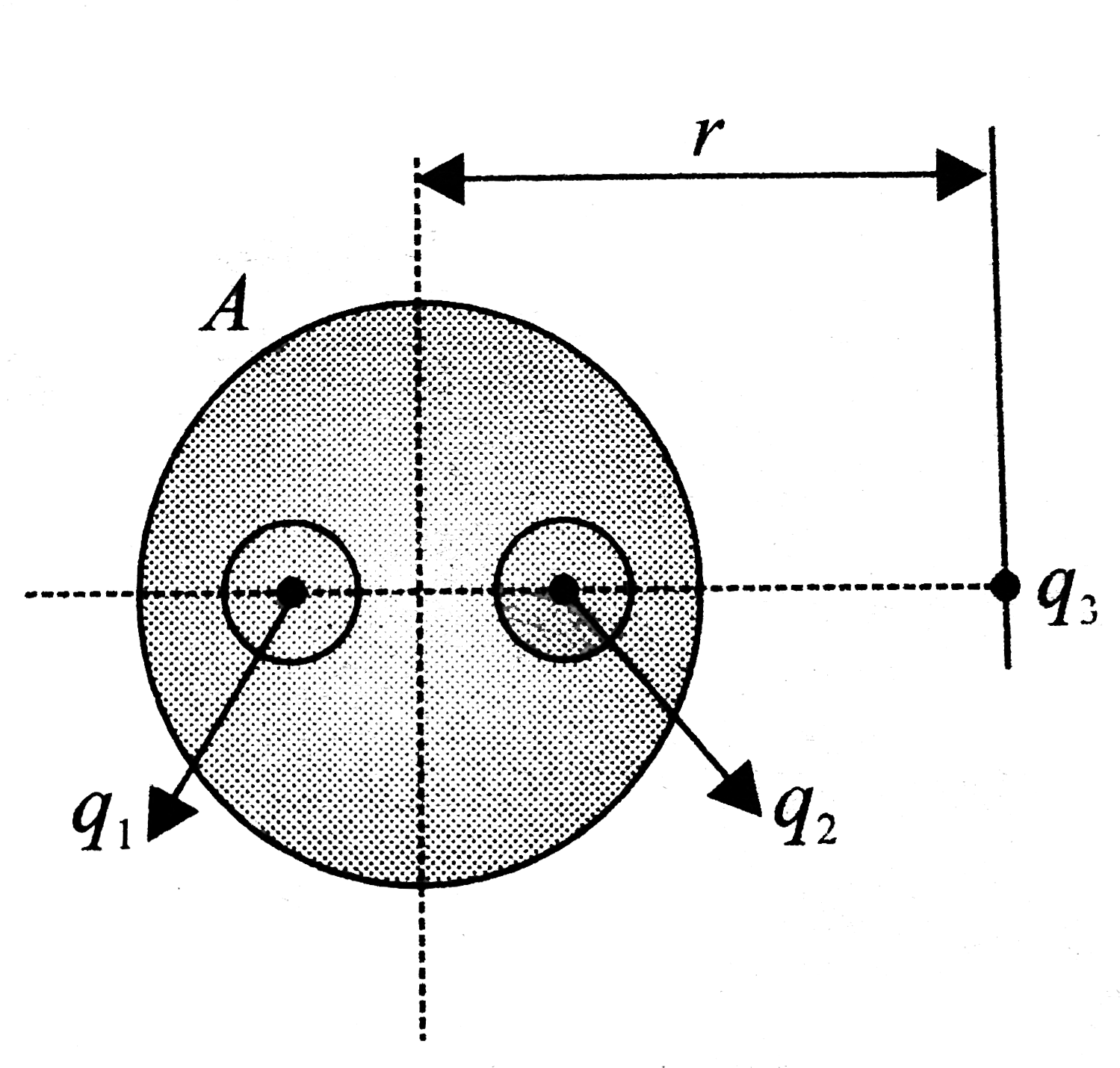
Which of the following statements are true ?
ACharge $q_{3}$ applies a larger force on the charge $q_{2}$ than on charge $q_{1}$ .
BCharge $q_{3}$ applies a smaller force on the charge $q_{2}$ than on charge $q_{1}$ .
CCharge $q_{3}$ applies equal force on both the charges.
DCharge $q_{3}$ applies no force on any of the charges.
Question 3 - Select One
A spherical conductor A contains two spherical cavities. The total charge on the conductor itself is zero. However, there is a point charge $q_{b}$ at the centre of one cavity and $q_{c}$ at the centre of the other. At considerable distance r away from the centre of the spherical conductor, there is another charge $q_{d}$ . Forces acting on $q_{b}, q_{c} and q_{d}$ are $F_{1}, F_{2}, and F_{3}$ , respectively. [Assume all charges are positive.]

A $F_{1} < F_{2} < F_{3}$
B $F_{1} = F_{2} < F_{3}$
C $F_{1} = F_{2} > F_{3}$
D $F_{1} > F_{2} > F_{3}$