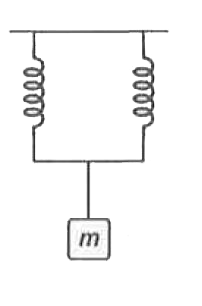
A mass m is suspended by means of two coiled springs which have the same length in unstretched condition as shown in figure. Their force constants are k1andk2 respectively. When set into vertical vibrations, the period will be

2π√(mk1k2)
2π√m(k1k2)
2π√(mk1−k2)
2π√(mk1+k2)
The correct Answer is:D
Since, keq=k1+k2
T=2π√mk1+k2.
Similar Questions
एक द्रव्यमान m समान लम्बाई की दो स्प्रिंगों से लटका हुआ है। स्प्रिंगों के बल नियतांक क्रमशः k1 व k2 हैं। जब पिण्ड को ऊर्ध्वाधर दिशा में दोलन कराया जाता है तो इसका आवर्तकाल होगा
View SolutionTwo springs are joined and attached to a mass of 16 kg. The system is then suspended vertically from a rigid support. The spring constant of the two springs are K_(1) and K_(2) respectively. The period of vertical oscillations of the system will be:
View SolutionTwo springs are joined and connected to mass m as shown. If the springs separately have force constants K1 and K2 , then frequency of oscillation of mass m is

View SolutionTwo springs are joined & attached to a mass of 16 kg. The system is then suspended vertically from a rigid support. The spring constants of the two springs are — K_(1) & K_(2) respectively. The period of vertical oscillations of the system will be -
View SolutionIn the arrangement shown in figure, pulleys are light and spring are ideal. K1, k2, k3and k4 are force constant of the spring. Calculate period of small vertical oscillations of block of mass m.

View SolutionAs shown in the figure, two light springs of force constant k_(1) and k_(2) oscillate a block of mass m. Its effective force constant will be
View SolutionThe spring constants of two springs of same length are k_(1) and k_(2) as shown in figure. If an object of mass m is suspended and set in vibration , the period will be
View SolutionA mass is suspended separately by two springs of spring constants k_(1) and k_(2) in successive order. The time periods of oscillations in the two cases are T_(1) and T_(2) respectively. If the same mass be suspended by connecting the two springs in parallel, (as shown in figure) then the time period of oscillations is T. The correct relations is
View Solution
