Where will be the centre of mass on combining two masses m and M(M>m)?
Towards m
Towards M
Between m and M
Anywhere
The correct Answer is:B
Since, position (r)∝1mass
Topper's Solved these Questions
- ROTATIONAL MOTIONBook:ERRORLESSChapter:ROTATIONAL MOTIONExercise:NCERT BASED QUESTIONS (Angular Displacement, Velocity and Acceleration)
Explore 34 Videos
- ROTATIONAL MOTIONBook:ERRORLESSChapter:ROTATIONAL MOTIONExercise:NCERT BASED QUESTIONS (Moment of Inertia)
Explore 139 Videos
- NEWTON'S LAWS OF MOTIONBook:ERRORLESSChapter:NEWTON'S LAWS OF MOTIONExercise:ASSERTION & REASON
Explore 18 Videos
- SIMPLE HARMONIC MOTIONBook:ERRORLESSChapter:SIMPLE HARMONIC MOTIONExercise:Assertion & Reason
Explore 15 Videos
Similar Questions
Two bodies of masses m1andm2(<m1) are connected to the ends of a massless cord and allowed to move as shown in figure. The pulley is both massless and frictionless. The acceleration of the centre of mass is (m1−m2)n(m1+m2)2g. Find the value of n.

View SolutionWe know that when two equal masses are placed on a line then, gravitational force on a third mass is zero due to these two masses when the third is placed at the centre of line joining the two masses. But if third mass is placed anywhere else on the x-axis, the gravitational force acts on that mass due to these two masses. Consider a system shown in figure.
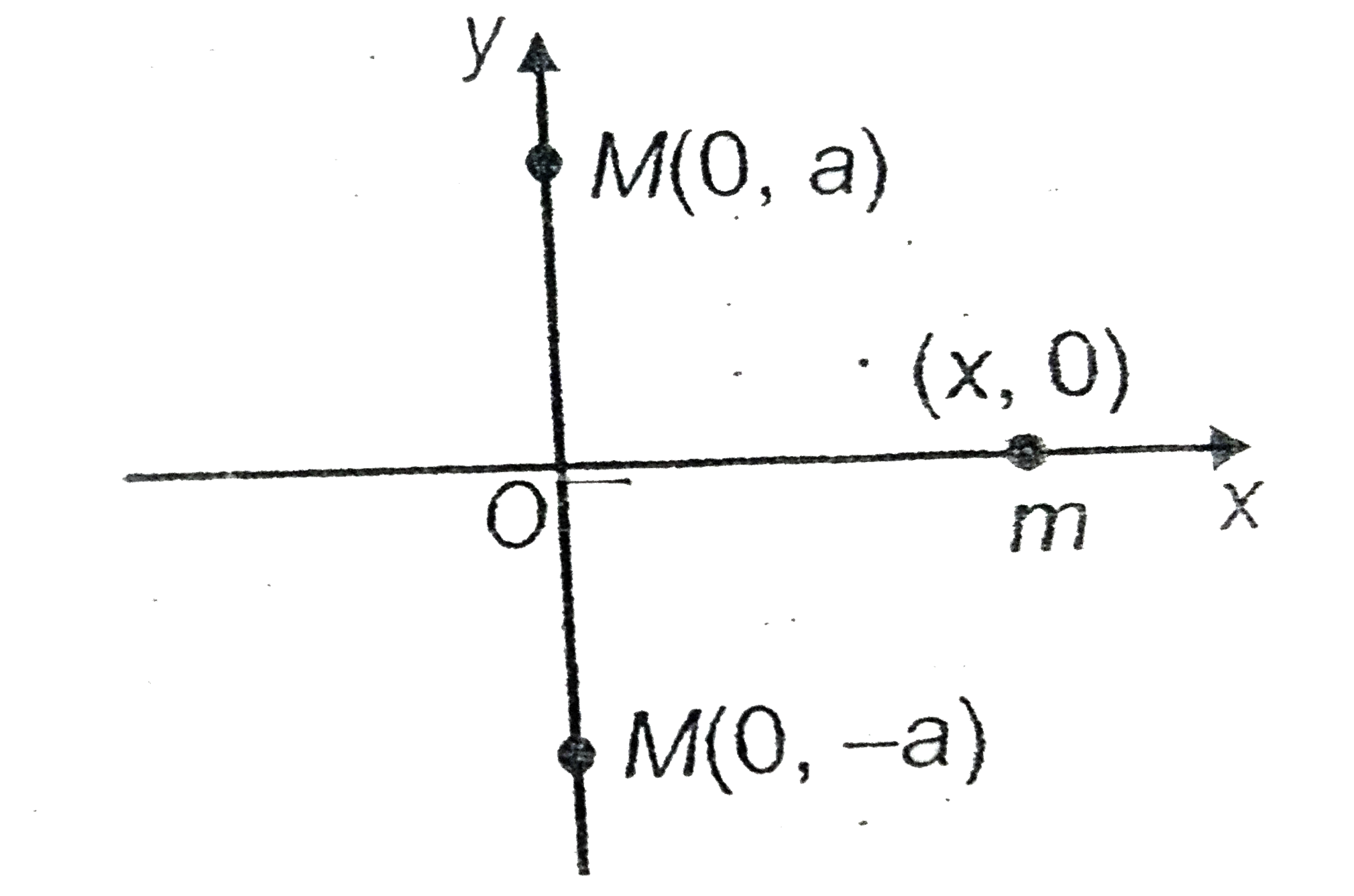
In this system two point masses of mass M each are placed at y-axis at a distance a from origin and these masses are fixed. If a third point mass of mass m is released at a distance x from origin on x-axis, then answer the following questions.
If a gt gt x, then which of the following statements is correct about the motion of mass m ?
View Solution
Knowledge Check
The reduce mass of two particles having masses m and 2 m is
A2 mB3 mC2 m/3Dm/2The centre of mass of a system of two particle of masses m1andm2 is at a distance d1 from m1 and at a distance d2 from mass m2 such that.
Ad1d2=m2m1Bd1d2=m1m2Cd1d2=m1m1+m2Dd1d2=m2m1+m2A pair of stars rotates about their centre of mass One of the stars has a mass M and the other has mass m such that M=2m The distance between the centres of the stars is d (d being large compared to the size of either star) .
The ratio of kinetic energies of the two stars (Km/KM) is .A1B2C4D9
Find the acceleration of centre of mass of the blocks of masses m1,m2(m1>m2) in Atwood's machine.
View SolutionThe centre of mass of a system of two particles of masses m1 and m2 is at a distance d1 from m1 and at a distance d2 from mass m2 such
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionIf →RCM is the position of the centre of mass of a system of two particles of masses m1 and m2 then →RCM is given by :
View SolutionThe centre of mass of two masses m & m' moves by distance x5 when mass m is moved by distance x and m' is kept fixed. The ratio m'm is
View Solution
