A sphere of mass M and radius r slips on a rough horizontal plane. At some instant it has translational velocity V0 and rotational velocity about the centre V02r. The translational velocity when the sphere starts pure rolling motion is Solution in Gujrati
Velociyt of the centre =v0 and the angular velocity about the centre =v02r. Thus v0>ω0r. The sphre slips forward and thsu the friction by the plane on the sphere will act backward. As the friction is kinetic its value is μN=μMg and the sphere will be decelerated by acm=fM. Hence
v(t)=v0−fMt............i
This friction will also have a torque Γ= about the centre. This torque is clockwise and in the directionof ω0. Hence the angular acceleration about the centre will be
alpa=fr(25)(Mr2)=5f2Mr
and the clockwise angular velocity at time t will be
ω(t)=5f2Mrt=v02r+5f2Mrt
pure rolling starts when v(t)=romega(t)<br>i.e.v(t)=v_0/2+(5f)/(2M)t....ii<br>elimiNAt∈tomiandii<br>5/2v(t)+v(t) =5/2 v_0+v_0/2<br>orv(t)=2/7xx3v_=6/7v_0<br>Thusthesphererollswithtranslationvelocity6v_0/7∈theforwarddirection.<br>A<erNAtive:≤tusconsrthe→rqueboutteh∈itilp∮ofcontactA.Theforceoffirctionipassesthroughthisp∮andhec≠its→rqueiszer⊙Thenorjalforceandtheweightbalanceeachother.The≠t→rqueaboutAiszer⊙Hencetheanga––rmomentumaboutAisconserved.<br>Initialanga––romentumis<br>L=L_(cm)+Mrv_0+I_(cm)omega+Mrv_)<br>=(2/5Mr^2)(v_0)/(2r)+Mrv_0=6/5Mrv_0<br>Thusanga––rvelocityisv/r.theanga––rmomentumabutAis<br>L=L_(cm)+Mrv<br>=(2/5Mr^2)(v/r)+mrv+7/5Mrv<br>Thsu6/5Mrv_0=7/5Mrv<br>or v=6/7v_0
Similar Questions
A sphere of mass M and radius r shown in figure slips on a rough horizontal plane. At some instant it has translational velocity V0 and rotational velocity about the centre v02r. Find the translational velocity after the sphere starts pure rolling.
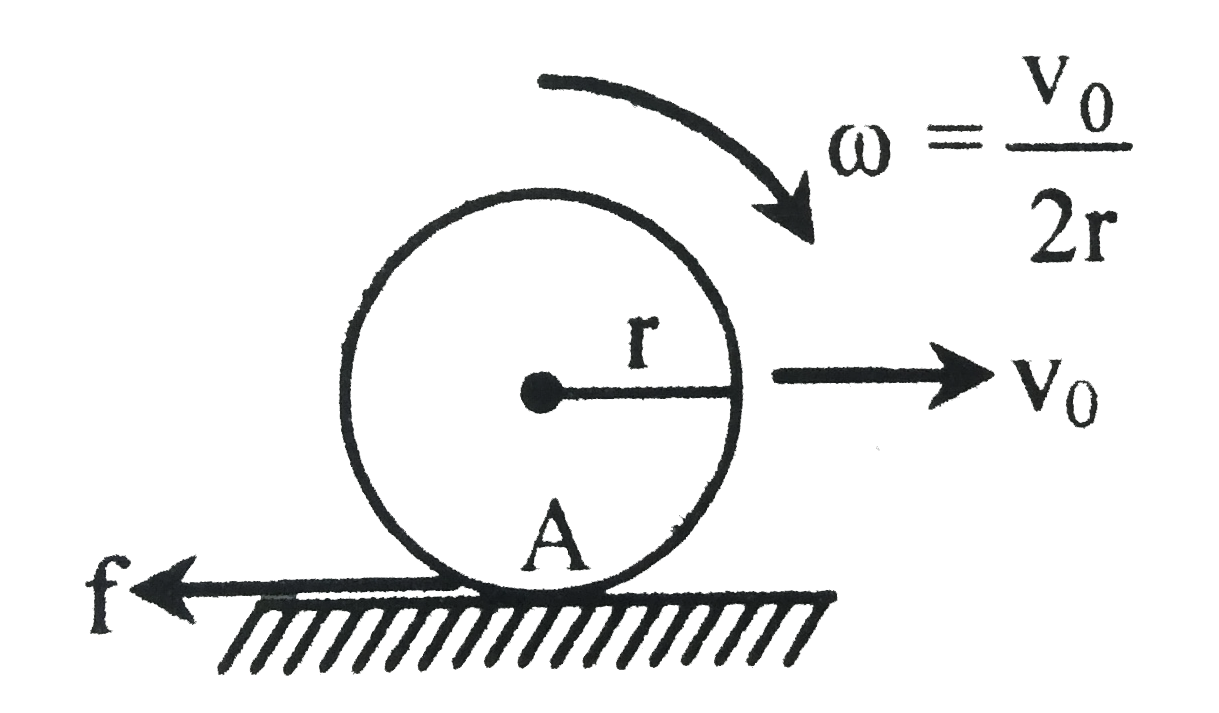 .
.
View SolutionA sphere of mass M and radius r shown in figure slips on a rough horizontal plane. At same instant it has translational velocity v0 and rotational velocity about the centre v0/2r. Find the translational velocity (in m/s) after the sphere start pure rolling (Take v0=7 m/s)
View SolutionA uniform ring of radius R is given a back spin of angular velocity V_(0)//2R and thrown on a horizontal sorgh surface with velocity of centre V_(0) . The velocity of the centre of the ring when it starts pure rolling will be
View SolutionA solid sphere has combined linear and rotation motion as shown in figure. The velocity of its centre of mass when it starts pure rolling is (R = radius of sphere)
View SolutionA solid sphere and a hollow sphere of equal mass and radius are placed over a rough horizontal surface after rotating it about its mass centre with same angular velocity omega_(0) . Once the pure rolling starts let v_(1) and v_(2) be the linear speeds of their centres of mass. Then
View SolutionA uniform ring of radius R is given a back spin of angular velocity V0/2R and thrown on a horizontal rough surface with velocity of centre to be V0. The velocity of the centre of the ring when it starts pure rolling will be
View SolutionA sphere of mass M and radius r shown in the figure on a rough horizontal plane. At some instant it has translational velocity v0, and rotational velocity about centre v0/2r. The percentage change of translational velocity after the sphere start pure rolling:
View SolutionA solid sphere of mass M and radius r slips on a rough horizontal plane. At some instant it has translational velocity v0androtationalvelocityaboutthecentrev_(0) / 2 r.Thetranslationalvelocityafterthesphere⋆tspureroll∈gis(x v_(0))/(y)∈forwarddirection.F∈d(x+y).
'(##CEN_KSR_PHY_JEE_C09_E01_027_Q14##)'
View Solution
