Question

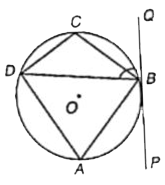
ABCD is a cyclic quadrilateral. PBQ is a tangent drawn from the point B of the circle, if $\angle DBP={65}^{\circ }$, then measures of $\angle BCD$ is

Options

A. `65^(@)`
B. `90^(@)`
C. `110^(@)`
D. `115^(@)`

Answer

D

Explanation

To solve the problem, we need to find the measure of angle BCD in the cyclic quadrilateral ABCD, given that angle DBP is 65 degrees.

1. Understand the Given Information:
- ABCD is a cyclic quadrilateral.
- PBQ is a tangent to the circle at point B.
- Angle DBP = 65 degrees.

2. Draw the Figure:
- Draw the cyclic quadrilateral ABCD.
- Mark the tangent PBQ at point B.
- Label the angles as given in the problem.

3. Identify Angles:
- Since PBQ is a tangent to the circle at point B, the radius OB is perpendicular to the tangent PB. Therefore, angle OBD + angle DBP = 90 degrees.
- Let angle OBD be denoted as \( \theta \). Then:
\( \theta + 65^\circ = 90^\circ \)
- Solving for \( \theta \):
\( \theta = 90^\circ - 65^\circ = 25^\circ \)

4. Use Isosceles Triangle Properties:
- In triangle OBD, OB = OD (both are radii of the circle).
- Therefore, triangle OBD is isosceles, which means angles OBD and ODB are equal.
- Let angle OBD = angle ODB = \( \theta = 25^\circ \).

5. Find Angle DOB:
- The sum of angles in triangle OBD is:
\( \text{Angle OBD} + \text{Angle ODB} + \text{Angle DOB} = 180^\circ \)
- Substituting the known angles:
\( 25^\circ + 25^\circ + \text{Angle DOB} = 180^\circ \)
- Therefore:
\( \text{Angle DOB} = 180^\circ - 50^\circ = 130^\circ \)

6. Relate Angle DAB to Angle DOB:
- Since angle DAB subtends the same arc as angle DOB at the circumference, we have:
\( \text{Angle DAB} = \frac{1}{2} \times \text{Angle DOB} = \frac{1}{2} \times 130^\circ = 65^\circ \)

7. Use the Cyclic Quadrilateral Property:
- In cyclic quadrilateral ABCD, the opposite angles are supplementary:
\( \text{Angle DAB} + \text{Angle BCD} = 180^\circ \)
- Substituting the known value:
\( 65^\circ + \text{Angle BCD} = 180^\circ \)
- Therefore:
\( \text{Angle BCD} = 180^\circ - 65^\circ = 115^\circ \)

Final Answer:
The measure of angle BCD is \( 115^\circ \).