Figure shows a liquid of densith 1200kgm−3 flowing steadily in a tube of varying cross section. The cross section at a point A is 1.0cm2 and that at B is 20mm2, the points A and B are in the same horizontal plane.The speed of the liquid at A is 10 cms−1. Calculate the difference in pressure at A and B.
 Solution in Tamil
Solution in Tamil
From equation of continuity the speed v2 at B is given by
A1v1=A2v2 ltbrrarr (1.0cm2)(10cms−1)=(20mm2)v2
orv2=1.0cm220mm2×10cms−1=50cms−1
By Bernoullil equation
P1+ρgh1+12ρv21=P2+ρgh2+12ρv22.
here h1=h2 Thus,
P1−P2=12ρv22−12ρv21
=12×91200kgm−3)(2500cm2s−2−100cm2^−2)
=600kgm−3×2400cm2s−2=144Pa
Topper's Solved these Questions
Similar Questions
Find the accelerationof the moon with respect to the eath from the following data, Distance between the earth and the moon =3.85×105 km and the time taken by the moon to complete one revolution around the earth =27.3days
View SolutionA body slipping on a rough horizontal plane moves wioth a deceleationof 4.0ms. What is cofficient of kineic friction between the block and the plane?
View SolutionFigure shows a liquid flowing through a tube at the rate of 0.1 m^(3)//s . The tube is branched into two semicircular tubes of cross-sectional area A//3 and 2A//3 . The velocity of liquid at Q is (the cross section of the main tube is A =10^(-2) m^(2) and v_(p) = 20 m//s )
View SolutionA horizontal tube has different cross sections at points A and B. The areas of cross section are a1 and a2 respectively, and pressures at these points are p1=ρgh1 and p2=ρgh2 where ρ is the density of liquid flowing in the tube and h1 and h2 are heights of liquid columns in vertical tubes connected at A and B. If h1−h2=h, then the flow rate of the liquid in the horizontal tube is
View SolutionIn the figure, an ideal liquid flows through the tube, which is of uniform cross section. The liquid has velocities v_(A) and v_(B) , and pressures P_(A) and P_(B) at the points A and B , respectively. Then
View SolutionA liquid of density 800 kg//m^3 flowing steadily in a tube of varying cross-section. If area of cross-section at A is 4 cm^2 and at B is 2 cm^2 . If speed of liquid at A is 10 cm//s , calculate (i) the rate of flow (ii) the difference in pressures at A and B .
View SolutionWater is flowing steadily through a horizontal tube a of non uniform cross-section. If the pressure of water is 4xx10^(4)N//m^(2) at a point where cross-section is 0.02m^(2) and velocity of flow is 2m//s , what is pressure at a point where cross-section reduces to 0.01m^(2)
View SolutionHow does the pressure of liquid depend on the area 'of cross section of tube of flow?
View SolutionIn this figure, an ideal liquid flows through the tube having uniform area of cross-section and is held in vertical plane. Find the ratio of speed of liquid at A and B and also find the pressure difference between- these points.
View SolutionAt two points on a horizontal tube of varying cross section carrying water, the radii are 1cm and 0.4cm. The pressure difference between these points is 4.9cm of water. How much liquid flows through the tube per second?
View SolutionIn the figure, an ideal liquid flows through the tube, which is of uniform cross section. The liquid has velocities v_(A) and v_(B) , and pressures P_(A) and P_(B) at the points A and B , respectively. Then
View SolutionThe given figure shows a streamline flow of a non-viscous liquid having density 1000kg/m3.The cross sectional area at point A is 2cm2 and at point B is 1mm2.The speed of liquid at the point A is 5cm/s. Both points A and B are at the same horizontal level. Calculate the difference in pressure at A and B.
View SolutionA liquid of density 800kg/m3 flowing steadily in a tube of varying cross-section. If area of cross-section at A is 4cm2 and at B is 2cm2, if speed of liquid at A is 10cm/s, calculate:- the rate of flow at B
View SolutionA liquid of density 800kg/m3 flowing steadily in a tube of varying cross-section. If area of cross-section at A is 4cm2 and at B is 2cm2, if speed of liquid at A is 10cm/s, calculate:- the difference in pressure at A and B.
View SolutionFigure shows a liquid flowing through a tube at the rate of 0.1m3/s. The tube is branched into two semicircular tubes of cross-sectional area A/3 and 2A/3. The velocity of liquid at Q is (the cross section of the main tube is A=10−2m2 and vp=20m/s)
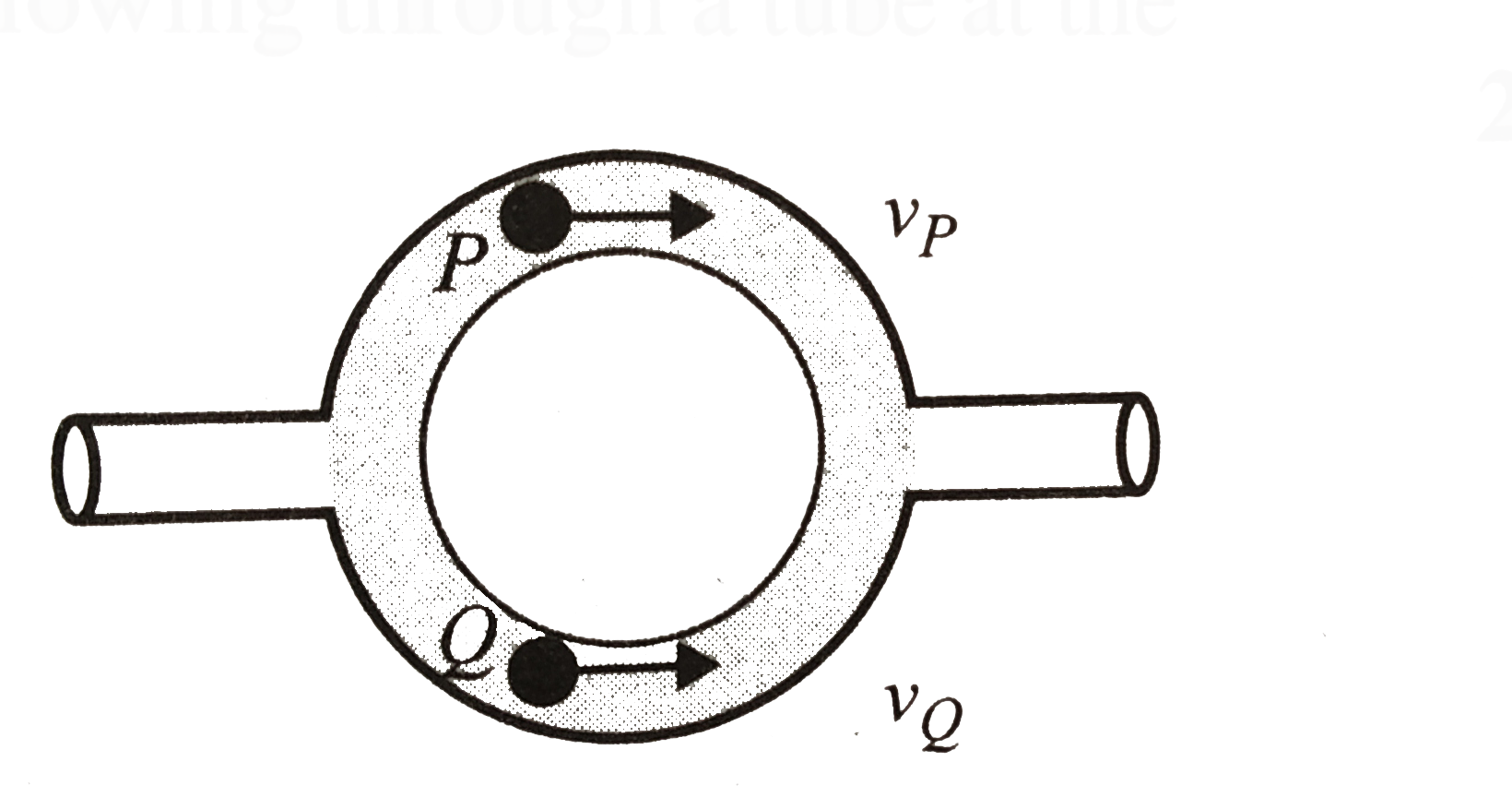
View SolutionA horizontal tube has different cross sections at points A and B . The areas of cross section are a_(1) and a_(2) respectively, and pressures at these points are p_(1)=rhogh_(1) and p_(2)=rhogh_(2) where rho is the density of liquid flowing in the tube and h_(1) and h_(2) are heights of liquid columns in vertical tubes connected at A and B . If h_(1)-h_(2)=h , then the flow rate of the liquid in the horizontal tube is
View Solution
