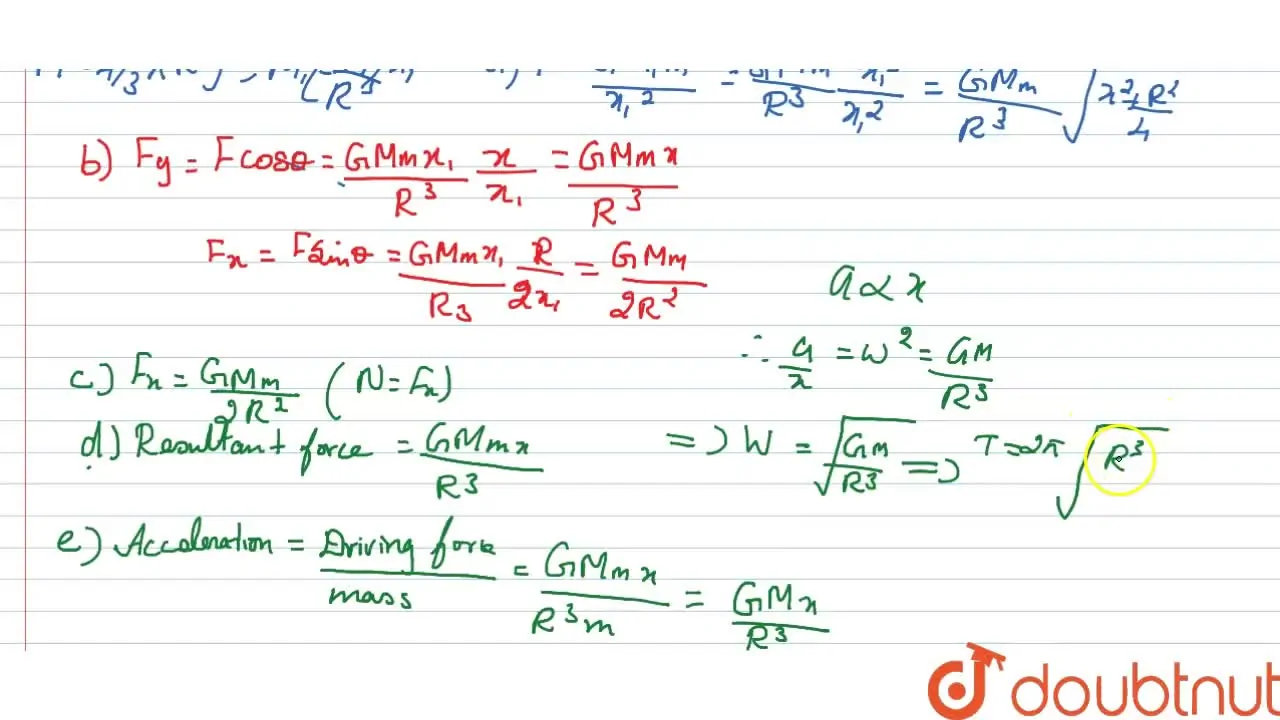
Assume that a tunnel ils dug along a chord of the earth, at a perpendicular distance R/2 from the earth's centre where R is the radius of the earth. The wall of the tunnel is frictionless. a. Find the gravitational force exerted by the earth on a particle of mass m placed in the tunnel at a distance x from the centre of the tunnel. b. Find the component of this fore along the tunnel and perpendicular to the tunnel. c. Find the normal force exerted by the wall on the particle. d. Find the resultant force on the particle. e.Show that the motion of the particle in the tunnel is simple harmonic and find the time period. Solution in Tamil

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C, D

$M = \frac{4}{3} π R^{3} ρ$
$M^{1} = \frac{4}{3} π x_{1}^{3} ρ$
$M^{1} = (\frac{M}{R^{3}}) π_{1}^{3}$
a. F=GravitatioN/A force
Exerted by the earth on the particle of mass m is
$F = \frac{G M_{1} m}{x_{1}^{2}} = \frac{G M m}{R^{3}} \frac{x_{1}^{3}}{x_{1}^{2}}$
$= \frac{G M m}{R^{3}} x_{1} = \frac{\frac{G M m}{R^{3}}}{[x^{2} + (\frac{R^{2}}{4})]}$
$F_{y} = F cos θ$
$= \frac{G M m x_{1}}{R^{3}} \frac{x}{x_{1}} = \frac{G M m x}{R^{2}}$
$F_{x} = F sin θ$
$= (G M m \frac{x_{10}}{R^{3}} \frac{R}{2 x_{1}} = \frac{G M m}{2 R^{2}}$
$F - x = \frac{G M m}{R^{2}}$
[since normal force exerted by the wall N=Fx]
Resultant force = $\frac{G M m x}{R^{3}}$
Acceleration=Driving force/mass
$= \frac{G M m x}{R^{3} m} = \frac{G M x}{R^{3}}$
So a alphax (the body makes SHM).
$∴ \frac{a}{x} = w^{2} = \frac{G M}{R^{3}}$
$\to v = \sqrt{\frac{G M}{R^{3}}}$
$\to T = 2 π (\frac{R^{3}}{G M})$

Updated on:21/07/2023

|

Class 11 PHYSICS OSCILLATIONS

Topper's Solved these Questions

Similar Questions

HC VERMA-SIMPLE HARMONIC MOTION-Exercises

Home
Ask Doubt
Profile