A particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?
 Solution in Tamil
Solution in Tamil
Take X,Y-axis as shown in ure Suppose that the particle strikes the plane at a point P with coordiN/Ates (x,y). Consider the motion between A and P.
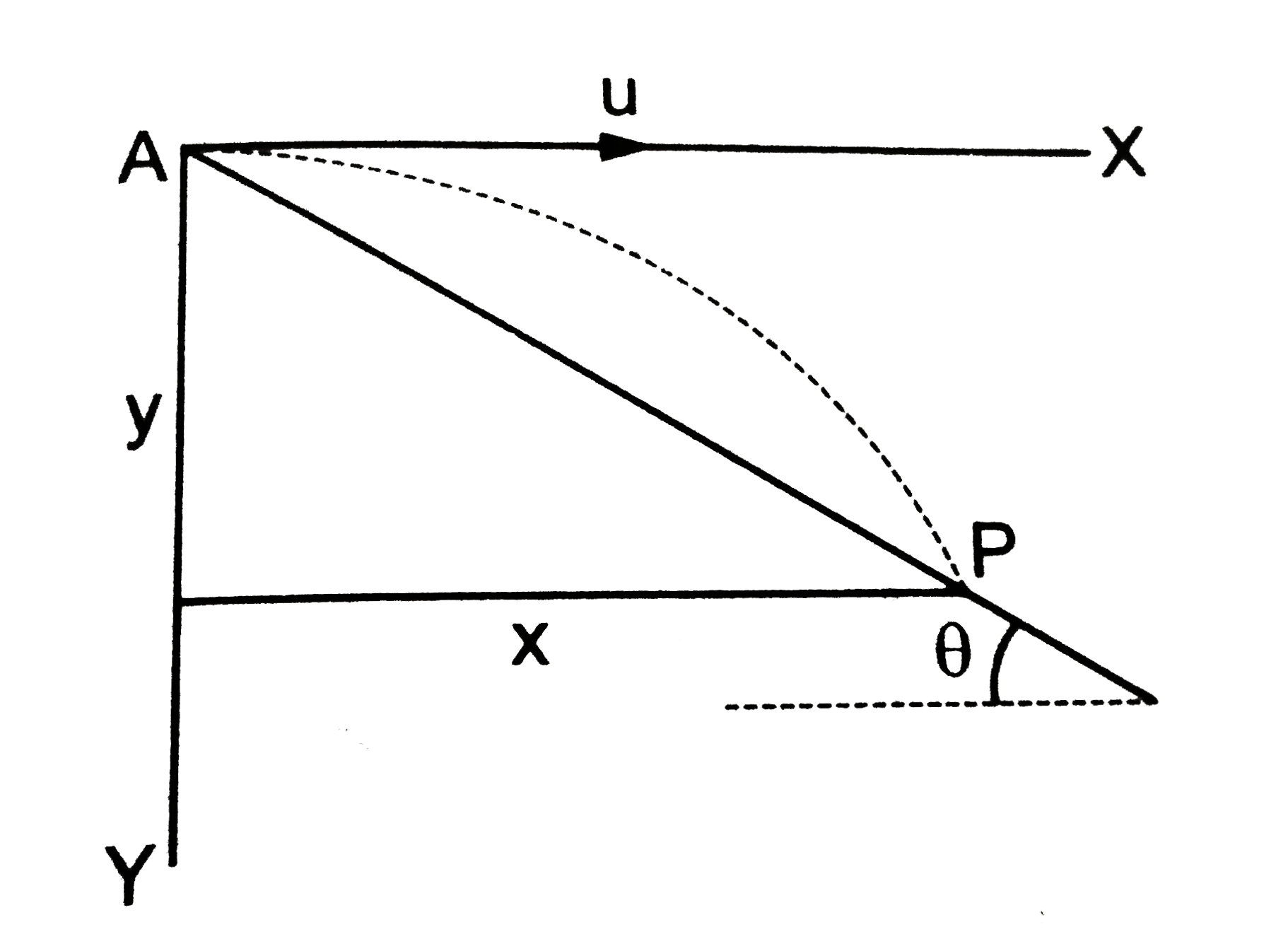
Motion in x- direction
Initialvelocity=u
Ae≤ration=g
y=12>2
ElimiN/Ating t from i. and ii
y=12gx2u2
Also y=xtanθ
Thus,(gx^2)/(2u^2)=x tan theta giv∈gx=0, or, 2u2tanθg,<br>theny= x tantheta= (2u^2 tan^2 theta)/g<br>ThedistanceAP=l=sqrt(x^2+y^2)<br>=(2u^2)/g tan theta sqrt (1+tan^2theta)<br>=(2u^2)/g tantheta sec theta.A<erNAtively:Taketheaxesasshown∈ure.consrthemotionbetweenAandP.<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/HCV_VOL1_C03_S01_024_S02.pngwh=80%mx-autosty≤=background:white;><br>MotionalongtheX−aξs:<br>Intialvelocity=u cos theta<br>Ae≤ration=g sin theta<br>Displacement =AP.<br>Thus,AP=(u cos theta)t+1/2(g sin theta)t^2<br>MotionalongtheY−aξs<br>Initial velocity =-u sin theta<br>acceleration =g cos theta<br>Displacement =0<br>Thus, 0=- uy sin theta +1/2 gt^2 cos theta<br>or, t=0,2usinθg(cosθ)
clearly the point P corresponds to t=2usinθgcosθ
Putting this value of t in i.
AP=(ucosθ)(2usinθgcosθ)+gsinθ2(2usinθgcosθ)2
2u2sinθg+2u2sinθtan2θg
=2u2gsinθsec2θ=2u2gtanθsecθ.
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer14videos
REST AND MOTION : KINEMATICS
- BOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Objective -113videos
REST AND MOTION : KINEMATICS
- BOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises51videos
REST AND MOTION : KINEMATICS
- BOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises34videos
PHYSICS AND MATHEMATICS
- BOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises86videos
ROTATIONAL MECHANICS
Similar Questions
Galileo was punished by the Church for teaching that the sum is stationary and the earth moves around it. His opponents held the view that the earth is stationary and the sun moves around it. If the absolute motion has no meaing,are the two view points not equally correct or equally wrong?
05:39View SolutionA motor car is going due north at a speed of 50 km/h. It makes a 900 left turn without changing the speed. The change in the velocity of the car is about
05:17View SolutionA man goes 10 m due east and then 24 m due north. Find the distance of his current position from the starting point ?
04:13View SolutionA vector →A makes an angle of 20∘and→B makes an angle of 110∘ with the X-axis. The magnitude of these vectors are 3 m and 4 m respectively.Find the resultant
04:47View SolutionA wheel is making revolutions about its axis with uniform angular acceleration. Starting from rest, till it reaches 100 rev/sec in 4 seconds. Find the angular acceleration.
02:45View SolutionA particle is projected with a certain velocity at an angle ∝ above the horizontal from the foot of an inclined plane of inclination 30∘. If the particle strikes the plane normally, then ∝ is equal to.
00:47View SolutionA ball is projected horizontally with a speed v from the top of the plane inclined at an angle 45∘ with the horizontal. How far from the point of projection will the ball strikes the plane?
01:19View SolutionA pariicle is projected horizontally with a speed (u) from top of a plance inclined at anangle θ with the horizontal direction. How far from the point of projection will the particle strike the plane ?
03:22View SolutionA ball is projected horizontally with a speed u from the top of inclined plane of inclination α with horizontal. At what distance along the plane, the ball will strike the plane ?
08:25View SolutionA particle is projected with a certain velocity at an angle alpha above the horizontal from the foot of an inclined plane of inclination 30^(@) . If the particle strikes the plane normally then alpha is equal to
04:14View SolutionA particle is projected from horizontal ground at angle 'θ' with speed 'u'. In same plane of motion a horizontal acceleration 'a' exists so that projected particle returns back to point of projection. Find time of flight.
View SolutionA particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle theta=37^(@) to the horizontal (g=10m//s^(2)) How far from the point of projection will the particle strike the plane?
View SolutionA particle is projected up an inclines plane. Plane is inclined at an angle theta with horizontal and particle is projected at an angle alpha with horizontal. If particle strikes the plane horizontally prove that tan alpha=2 tan theta
View SolutionA particles is projected horizontally with a speed v from the top of a plane inclined at an angle tehta to the horizontal as shown in the figure.
(a) Hwo far from the point of projection will the particle strike the plane ?
(b) Find the time taken by the particel to hit the plane.
(c) What is the velocity of particle just before it hits the plane ?05:16View SolutionA particle is thrown horizontally from the top of an inclined plane , with a speed of 5m/s . If the inclination of the plane is 30∘ with the horizontal , how far from the point of projection will the particle strike the plane ?
View SolutionA particle projected horizontally from the top of an inclined plane inclined at an angle theta with the horizontal with velocity u. What is the distance along the plane from the point of projection at which the projectile strikes the inclined plane ?
07:58View Solution
