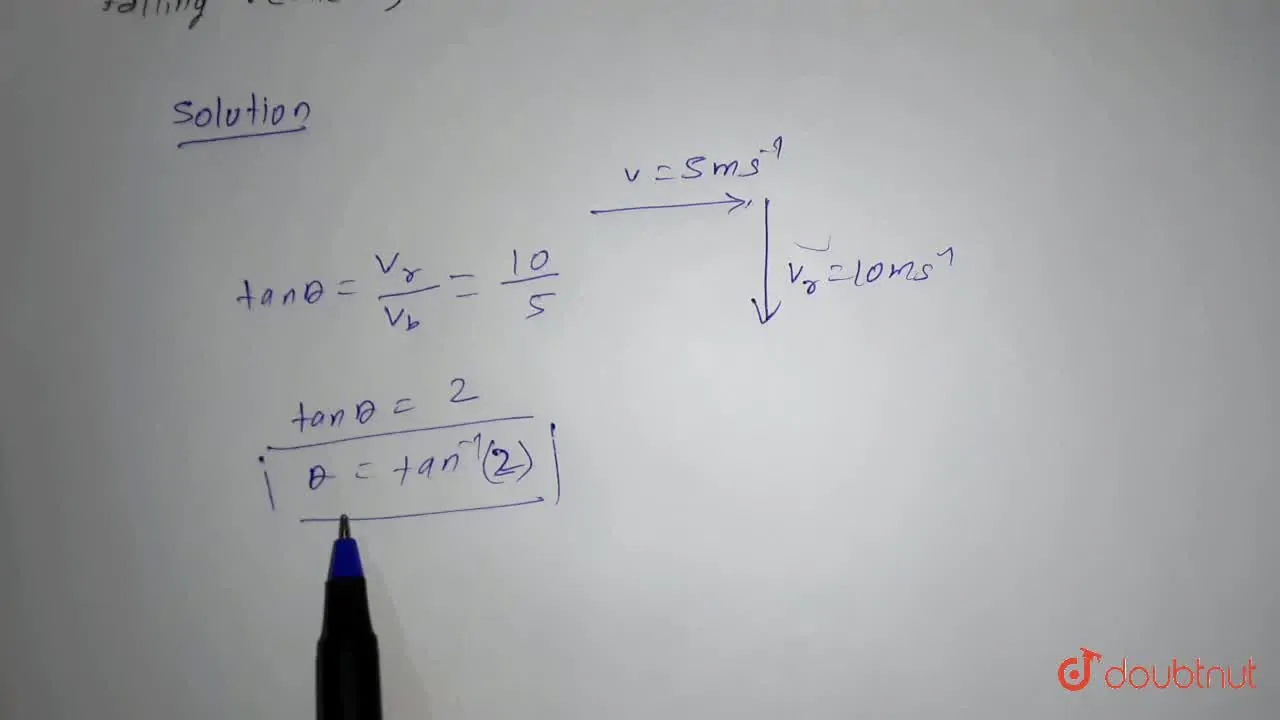A boy is running on a horizontal road with a velocity of 5 m/s. At what angle should he hold his umbrella in order to protect him self from the rain if the rain is falling vertically with a velocity of 10 m/s?
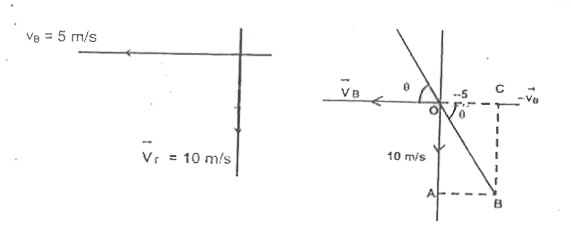
To obtain the relative velocity of rain w.r.t. boy a velocity triangle is foremed between ¯¯¯VB and ¯¯¯Vr as shown.
Let θ be the angle made by the resultant with the horizontal .
tanθ=105=2
θ=tan−1(2)
Similar Questions
A man runs along a horizontal road on a rainy day. His umbrella vertical in order to protect himself from the rain then rain actually
View SolutionA glass wind screen whose inclination with the vertical can be changed is mounted on a car. The moves horizontally with a speed of 2m/s. At what angle α with the vertical should the wind screen placed so that the rain drops falling vertically downwards with velcoity 6m/s strike the wind screen perpendicularly?
View SolutionBy the term velocity of rain, we mean velocity with which raindrops fall relative to the ground. In absence of wind, raindrops fall vertically and in presence of wind raindrops fall obliqucly. Moreover raindrops acquire a constant terminal velocity due air resistance very quickly as they fall toward the carth. A moving man relative to himself observes an altered velocity of raindrops. Which is known as velocity of rain relative to the man. It is given by the following equation.
→vrm=→vr−→vm
A standstill man relative to himself observes rain falling with velocity, which is equal to velocity of the raindrops relative to the ground. To protect himself a man should his umbrella against velocity of raindrops relative to himself as shown in the following figure.

Rain is falling vertically with velocity 80 cm/s.
(a) How should you hold your umbrella ?
(b) You start walking towards the east with velocity 60 cm/s. How should you hold umbrella ?
(c) You are walking towards the west with velocity 60 cm/s. How should you hold your umbrella ?
(d) You are walking towards the north with velocity 60 cm/s. How should you hold your umbrella?
(e) You are walking towards the south with velocity 80 cm/s. How should you hold your umbrella ?View SolutionA person is walking in rain feels the velocity of rain as twice to his velocity. At which angle he should hold the umbrella with vertical if he moves forward, if it is raining vertically downwards
View SolutionA car is moving on horizontal ground with a velocity vm s−1 with respect to earth. The driver of the car observes the rain to be falling vertically downwards with velocity 2vm s−1 . What is the velocity of the rain with respect to the ground ?
View SolutionA man is travelling at 10.8 kmph in a topless car on a rainy day. He holds an umbrella at an angle of 37∘ with the vertical to protect himself from rain which is falling vertically downwards. What is the velocity of the rain drop ?
View SolutionStatement -1 : A man standing on the ground has to hold his umbrella at an angle θ with the vertical to protect himself from rain. It is possible that the man now starts running on the ground with certain speed, but he still has to hold his umbrella at angle θ with the vertical to protect himself from rain.
Statement -2 : Vertical component of velocity of rain w.r.t man does not change. if he starts running on a horizontal ground.View SolutionRain is falling vertically. A man running on the road keeps his umbrella tilted but a man standing on the street keeps his umbrella vertical to protect himself from the rain. But both of them keep their umbrella vertical to avoid the vertical sun-rays. Explain.
View SolutionA body is projected horizontally from the top of a hill with a velocity of 9.8 m/s. What time elapses before the vertical velocity is twice the horizontal velocity?
View SolutionRain is falling vertically with a velocity of 4 km hr−1 . A cyclist is going along a horizontal road with a velocity of 3 km hr−1 towards cast. Calculate the relative velocity of the rain with respect to the cyclist. In what direction must the cyclist hold his umbrella to save himself from the falling rain ?
View Solution