A projectile is thrown to have thte maximum possible horizontal range of 400 m. Taking the point of projecting as the origin, the co-ordinate of the point where the velocity of the projectile is minimum are
-400100
-200100
-400200
-200200
The correct Answer is:B

for maximum range θ=45∘
∵tanθ=4HR
H=R4tan45∘=4004=100
∴ required coordinates are (R2,H)=(200,100)
Topper's Solved these Questions
- MOTION IN A PALNEBook:ALLENChapter:MOTION IN A PALNEExercise:EXERCISE-3
Explore 40 Videos
- MOTION IN A PALNEBook:ALLENChapter:MOTION IN A PALNEExercise:SOLVED EXAMPLE
Explore 28 Videos
- MOTION IN A PALNEBook:ALLENChapter:MOTION IN A PALNEExercise:EXERCISE-1
Explore 99 Videos
- KINEMATICS-2DBook:ALLENChapter:KINEMATICS-2DExercise:Exercise (O-2)
Explore 46 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBook:ALLENChapter:NEWTON'S LAWS OF MOTION & FRICTIONExercise:EXERCISE (JA)
Explore 4 Videos
Similar Questions
A projectile is thrwon into space so as to have the maximum possible horizontal range equal to 400m. Taking the point of projection as the origin, the coordinates of the point where the velocity of the projectile is minimum are:-
View SolutionA projectile is thrown into space so as to have maximum horizontal range R. Taking the point of projection as origin, find out the co-ordinates of the point where the speed of the particle is minimum.
View Solution
Knowledge Check
If R and H represent horizontal range and maximum height of the projectile, then the angle of projection with the horizontal is
Atan−1(HR)Btan−1(2HR)Ctan−1(4HR)Dtan−1(4HH)
A projectile is thrown with velocity v at an angle θ with the horizontal. When the projectile is at a height equal to half of the maximum height,.
The velocity of the projectile when it is at a height equal to half of the maximum height is.
View SolutionA projectile is fired to have maximum range 500 m. Maximum height attained by the projectitle in this case will be
View SolutionA javelin thrown into air at an angle with the horizontal has a range of 200 m. If the time of flight is 5 second, then the horizontal component of velocity of the projectile at the highest point of the trajectory is
View SolutionThe horizontal range and the maximum height of a projectile are equal. The angle of projection of the projectile is
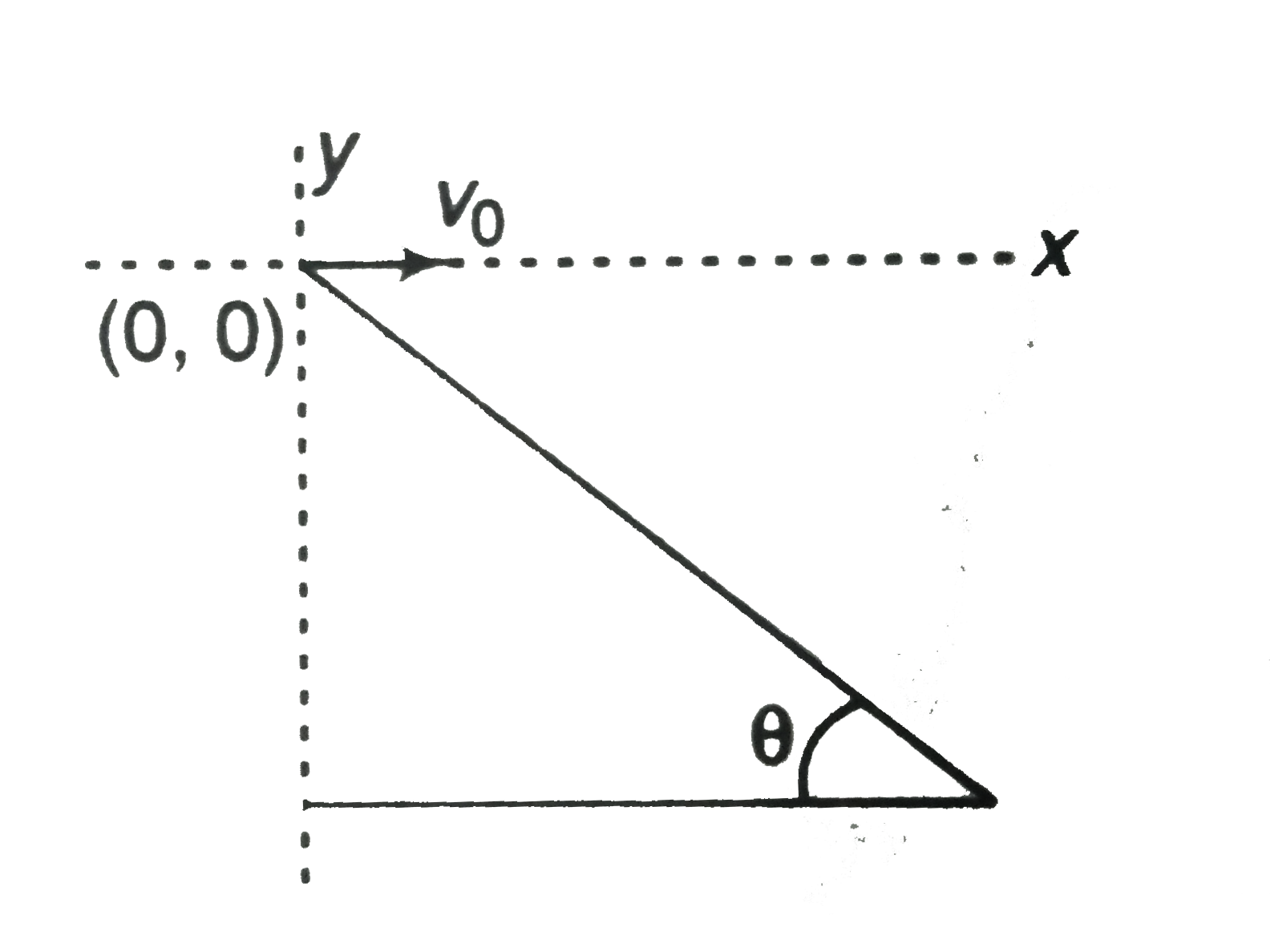
View SolutionA man standing on a hill top projects a stone horizontally with speed v0 as shown in figure. Taking the co-ordinate system as given in the figure. Find the co-ordinates of the point where the stone will hit the hill surface.
View SolutionA projectile is thrown with velocity v at an angle θ with the horizontal. When the projectile is at a height equal to half of the maximum height,.
The vertical component of the velocity of projectile is.
View SolutionIf the maximum vertical height and horizontal ranges of a projectile are same, the angle of projection will be
View Solution