The angle between vectors (A×B)and(B×A) is
In x-y plane
In y-z plane
In x-z plane
Along x-axis
The correct Answer is:B
→F1+→F2+→F3+→F4
=(4ˆi−5ˆj+5ˆk)+(−5ˆi+8ˆj+6ˆk)+(−3ˆi+4ˆj−7ˆk)+(12ˆi−3ˆj−2ˆk)=4ˆj+2ˆk
⇒ motion will be in y-z plane
Topper's Solved these Questions
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exercise-03
1 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exercise-04
1 Videos
- BASIC MATHSBOOK - ALLENCHAPTER - BASIC MATHSEXERCISE - Exercise-01
24 Videos
- AIIMS 2019BOOK - ALLENCHAPTER - AIIMS 2019EXERCISE - PHYSICS
40 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
Similar Questions
If A and B are two vectors such that |A+B|=2|A-B|. The angle between vectors A and B is
View SolutionThe angle between vectors (→A×→B)and(→B×→A) is :
View SolutionThe angle between Vectors (→A×→B) and (→B×→A) is
View SolutionIf A and B are Two non -zero vector having equal magnitude , the angle between the vector A and A-B is
View SolutionA = →i+→j . What is the angle between the vector and x-axis ?
View SolutionThe angle between two vector A and B is θ. Vector R is the resultant of the two vectors. If R makes an angle θ2 with A, then
View SolutionIf the angle between two vectors A and B is 120∘, then its resultant C will be
View SolutionIf the angle between the vectors A and B is θ, the value of the product(BxA)). A is equal to
View SolutionIf the angle between the vectors A and B is θ, the value of the product (BxA). A is equal to
View SolutionWhat is the angle between the vector →A and →B as shown in figure ?
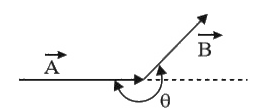
View Solution
