The magnitude of resultant of two equal forces is double the magnitude of either of forces, The angle between them is
30∘
60∘
120∘
150∘
The correct Answer is:B, C
∣∣→A.→B∣∣=AB|cosθ|=8,
∣∣→A×→B∣∣=AB|sinθ|=8√3
⇒|tanθ|=8√38=√3⇒θ=60∘,120∘
Topper's Solved these Questions
- BASIC MATHSBook:ALLENChapter:BASIC MATHSExercise:Exercise-03
Explore 1 Video
- BASIC MATHSBook:ALLENChapter:BASIC MATHSExercise:Exercise-04
Explore 1 Video
- BASIC MATHSBook:ALLENChapter:BASIC MATHSExercise:Exercise-01
Explore 24 Videos
- AIIMS 2019Book:ALLENChapter:AIIMS 2019Exercise:PHYSICS
Explore 40 Videos
- CIRCULAR MOTIONBook:ALLENChapter:CIRCULAR MOTIONExercise:EXERCISE (J-A)
Explore 6 Videos
Similar Questions
If the magnitude of sum of two vectors is equal to the magnitude of difference of the two vector, the angle between these Vector is
View SolutionIf the magnitude of sum of two vectors is equal to the magnitude of difference of the two vector, the angle between these Vector is
View SolutionTwo vectors of equal magnitude are acting through a point. The magnitude of resultant is equal to the magnitude of either vectoe. Find the angle between the vectors.
View SolutionFind the magnitude of the resultant of shown forces :-
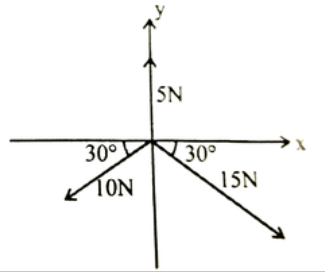
View SolutionIf magnitude of the resultant of two vectors equal of magnitude, is equal to the magnitude of either of the vectors, what is the angle between them ?
View SolutionTwo vectors of equal magnitude P are inclined at some angle such that the difference in magnitude of resultant and magnitude of either of the vectors is 0.732 times either of the magnitude of vectors. If the angle between them is increased by half of its initial value then find the magnitude of difference of the vectors
View SolutionMagnitude of resultant of two vectors →P and →Q is equal to magnitude of →P . Find the angle between →Q and resultant of →2P and →Q .
View SolutionThe square of resultant of two equal forces is three times their product. Angle between the force is
View SolutionAsserion: Magnitude of the resultant of two vectors may be less than the magnitude of either vector.
Reason: The resultant of two vectors is obtained by means of law of parallelogram of Vectors.
View SolutionTwo force in the ratio 1:2 act simultaneously on a particle. The resultant of these forces is three times the first force. The angle between them is
View Solution
