A body of mass 1.0 kg is suspended from a weightless spring having force constant 600Nm−1. Another body of mass 0.5 kg moving vertically upwards hits the suspended body with a velocity of 3.0ms−1 and gets embedded in it. Find the frequency of oscillations and amplitude of motion.
The correct Answer is:A, C
To solve the problem step by step, we will follow these steps:
Step 1: Understand the scenario
We have two bodies:
- Body 1 (mass m1=1.0kg) is suspended from a spring with a spring constant k=600N/m.
- Body 2 (mass m2=0.5kg) is moving upwards with a velocity v=3.0m/s and collides with Body 1, becoming embedded in it.
Step 2: Apply conservation of momentum
Before the collision, the momentum of the system is given by the momentum of Body 2 (since Body 1 is at rest):
Initial momentum=m2⋅v=0.5kg⋅3.0m/s=1.5kg m/s
After the collision, both bodies move together with a combined mass m1+m2=1.0kg+0.5kg=1.5kg and a new velocity v′:
Final momentum=(m1+m2)⋅v′
Setting the initial momentum equal to the final momentum:
1.5kg m/s=1.5kg⋅v′
Solving for v′:
v′=1.5kg m/s1.5kg=1.0m/s
Step 3: Calculate the angular frequency ω
The angular frequency ω of the oscillation can be calculated using the formula:
ω=√km
where m is the total mass after the collision:
ω=√600N/m1.5kg=√400=20rad/s
Step 4: Calculate the frequency f
The frequency f is related to the angular frequency ω by:
f=ω2π
Substituting the value of ω:
f=20rad/s2π=10πHz
Step 5: Calculate the amplitude A
The amplitude A can be calculated using the formula:
A=v′ω
Substituting the values of v′ and ω:
A=1.0m/s20rad/s=0.05m=5cm
Final Answers
- Frequency of oscillations: f=10πHz
- Amplitude of motion: A=5cm
We have two bodies:
- Body 1 (mass m1=1.0kg) is suspended from a spring with a spring constant k=600N/m.
- Body 2 (mass m2=0.5kg) is moving upwards with a velocity v=3.0m/s and collides with Body 1, becoming embedded in it.
Before the collision, the momentum of the system is given by the momentum of Body 2 (since Body 1 is at rest):
Initial momentum=m2⋅v=0.5kg⋅3.0m/s=1.5kg m/s
Final momentum=(m1+m2)⋅v′
1.5kg m/s=1.5kg⋅v′
v′=1.5kg m/s1.5kg=1.0m/s
The angular frequency ω of the oscillation can be calculated using the formula:
ω=√km
where m is the total mass after the collision:
ω=√600N/m1.5kg=√400=20rad/s
The frequency f is related to the angular frequency ω by:
f=ω2π
Substituting the value of ω:
f=20rad/s2π=10πHz
The amplitude A can be calculated using the formula:
A=v′ω
Substituting the values of v′ and ω:
A=1.0m/s20rad/s=0.05m=5cm
- Frequency of oscillations: f=10πHz
- Amplitude of motion: A=5cm
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-04 [B]
105 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-05 [A]
39 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Comprehension Base Questions (5)
3 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
A force acts for 0.1 s on a body of mass 2.0 kg initially at rest. The force is then withdrawn and the body moves with a velocity of 2m s−1. Find the magnitude of the force.
View SolutionA force acts for 0.1 s on a body of mass 2 kg initially at rest. The force is then withdrawn and the body moves with a velocity of 2 m/s. Find the magnitude of force.
View SolutionA body of mass 5 kg moving on a horizontal surface with a velocity of 10ms−1 comes to rest in 2s. The force required to make this body move with a velocity of 10ms−1 on the same surface is
View SolutionTwo rigid bodies A and B of masses 1 kg and 2 kg respectively are rigidly connected to a spring of force constant 400 Nm−1 . The body B rests on a horizontal table. From the rest position , the body A is compressed by 2 cm and then released . Deduce (i) teh frequency of oscillation, (ii) total oscillation energy, (iii) the amplitude of the harmonic vibration of the reaction of the table on body B.
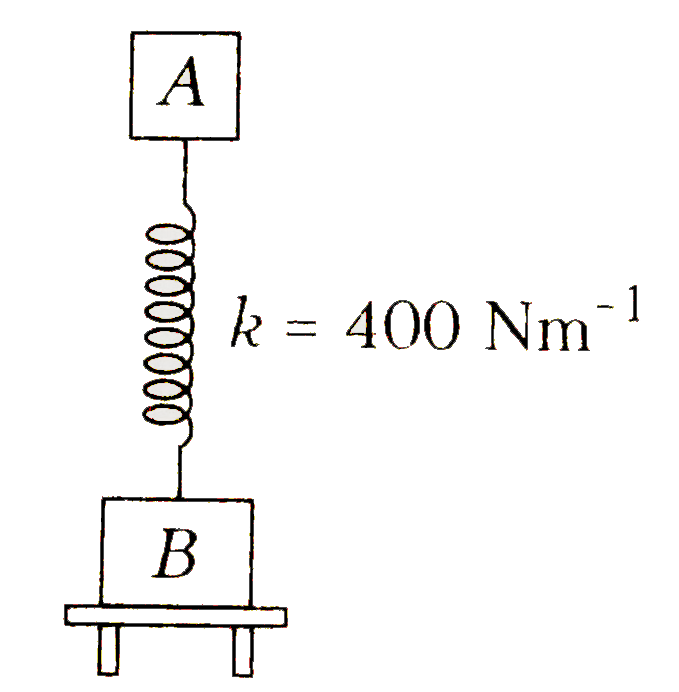
View SolutionA block of mass 0.01 kg is attached to a spring of force constant 10−1Nm−1 . If the energy of oscillation is 10−5 J, find the angular frequency and amplitude of oscillation.
View SolutionA body of mass 5 kg is raised vertically to a height of 10 m by a force 170 N. The velocity of the body at this height will be
View SolutionA weightless spring has a force constant k oscillates with frequency f when a mass m is suspended from it The spring is cut into three equal parts and a mass 3 m is suspended from it The frequency of oscillation of one part will now become
View SolutionA sliding fit cylindrical body of mass of 1kg drops vertically down at a constant velocity of 5cms−1. Find the viscosity of the oil.

View SolutionA body of mass 2kg collides with a horizontal weight spring of force constant 4Nm−1. The body compresses the spring by 1m from rest position. Find the speed of the block at the instant of collision ? Given that the coefficient of kinetic friction between the body and the surface is 0.1.
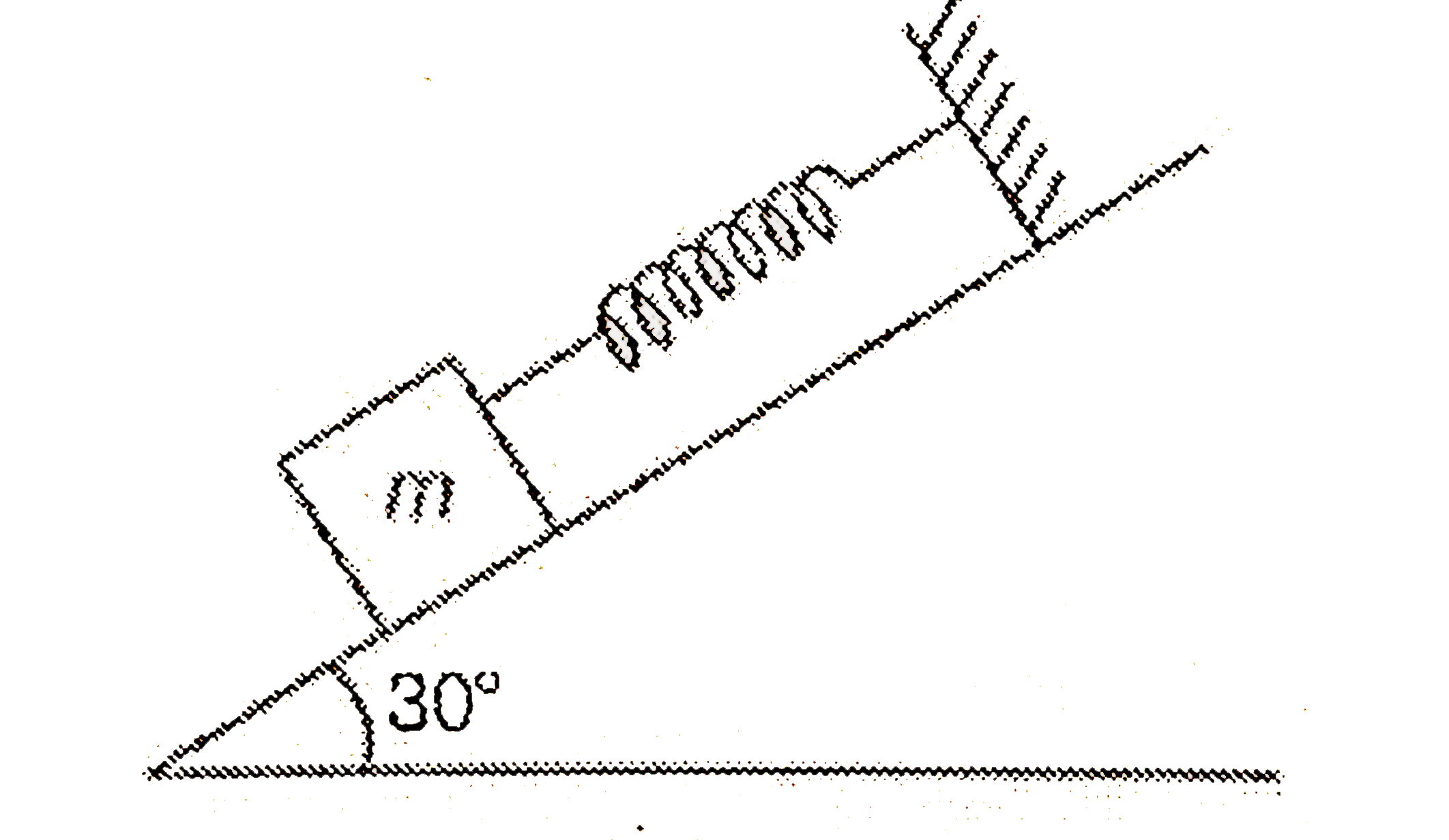
View SolutionA body of mass 5 kg is suspended by a spring balance on an inclined plane as shown in figure. The spring balance measure
View Solution
