A Rubber Ball Rebound
A rubber ball of mass 2.0kg falls to the floor. The ball hits with a speed of 8m/s and rebounds with approximately the same speed. High speed photographs show that the ball is in contact with the floor for 10^-3s. What can we say about the force exerted on the ball by the floor?

The momentum of the ball just before it hits the floor is Pa=1.6ˆkkg−m/s and its momentum 10−3 later is Pb=+1.6ˆkkg−m/s
Since tb∫taFdt=Pb−Pa
tb∫taFdt=1.6ˆk−(−1.6ˆk)=3.2ˆkkg−m/s
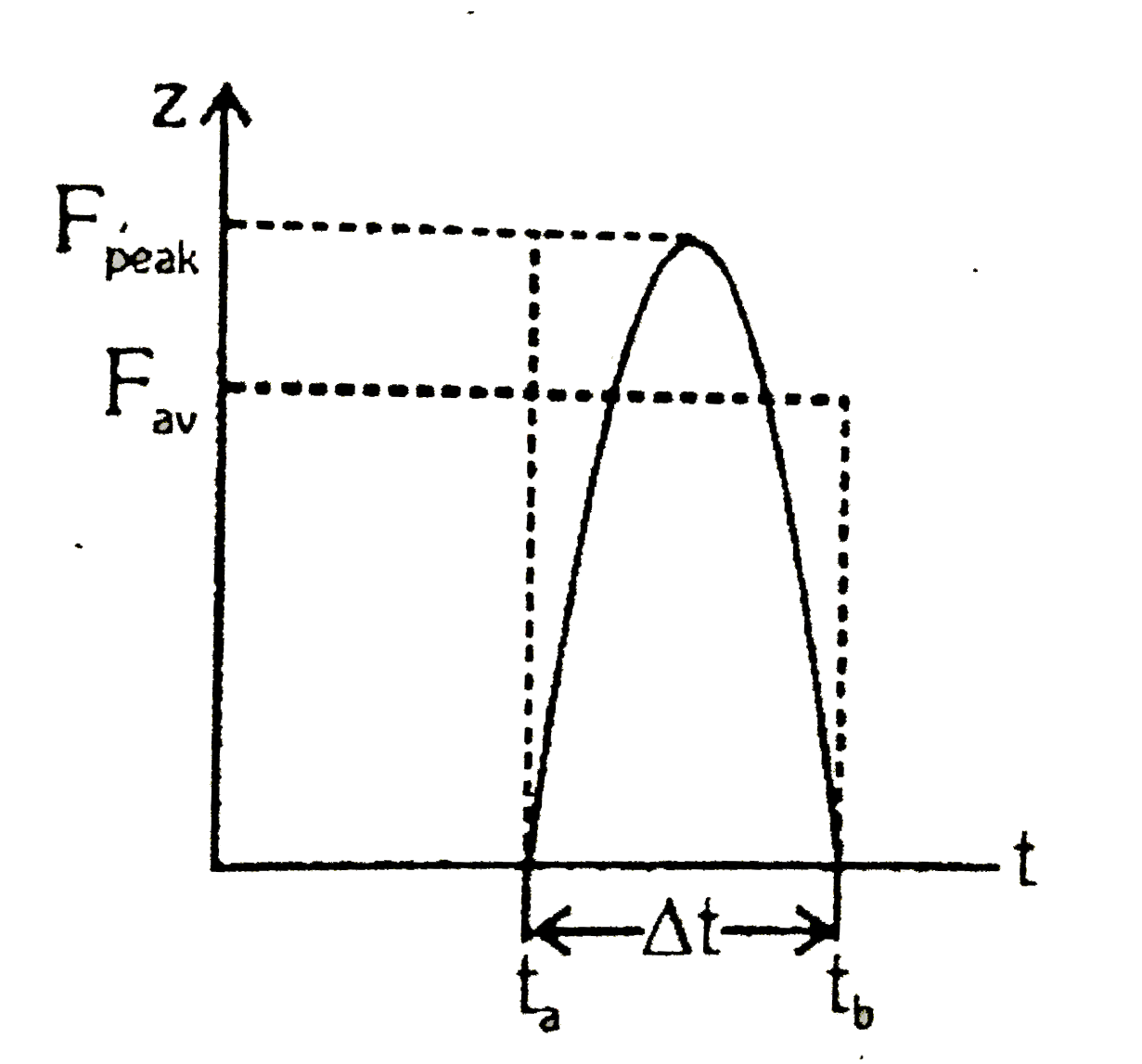
Although the exact variation of F with time is not known, it is easy to find the average force exerted by the floor on the ball. IF the collision time is Δt=tb−ta, the average force Fav acting during the collision is
FavΔt∫ta+ΔttaFdt
Since Δt=10−3s,
Fav=3.2ˆkkg−ms10−2s=3200ˆkN
The average force is directed upward, as we expect. In more familiar units, 3200N=720lb−−a sizable force. The instantanwous force on the ball is even larger at the peak, as the sketch shows. If the ball hits a resillient surface, the collision time is longer and the peak force is less.
Actually, there is a weakness in our treatment of the rubber ball rebound. In calculating the impulse ∫Fdt,F is the total force. this includes the gravitational force, which we have neglected. Proceeding more carefully, we write.
F=Ffloor+Fgrav=Ffloor−Mgˆk.
The impulse equation then becomes
∫10−30Ffloordt−∫10−30Mgˆkdt=3.2ˆkkg−m/s The impulse due to the gravitational force is −∫10−30Mgˆkdt=−Mgˆk∫10−30dt=(0.2)(9.8)(10−3)ˆk=−1.96×10−3ˆkkg−m/s
Topper's Solved these Questions
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-I
40 Videos
- CENTRE OF MASSBOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-II
43 Videos
- BASIC MATHEMATICS USED IN PHYSICS &VECTORS BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON
11 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)
3 Videos
Similar Questions
A ball weighing 10g hits a hard surface vertically with a speed of 5m/s and rebounds with the same speed The ball remains in contact with the surface speed The ball remains in contact with the surface for 0.01s The average force exerted by the surface on the ball is .
View SolutionA 3.00kg steel hall strikes the wall with a speed of 10.0m/s at an angle of 60∘ with the surface. It bounces off with the same speed and angle. If the ball is in contact with the wall for 0.200s, what is the average force exerted by the wall on the ball?
View Solution
Knowledge Check
A 3 kg ball strikes a heavy rigid wall with a speed of 10 m/s at an angle of 60∘. It gets reflected with the same speed and angle as shown in Fig. 15.3.11. It the ball is in contact with the wal for 0.20 s, what is the average force exerted on the ball by the wall ?
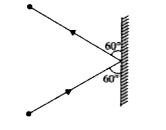 AzeroB150 NC150 √3 ND300 N
AzeroB150 NC150 √3 ND300 NA ball of mass m strikes a rigid walJ with speed u and rebounds with the same speed. The impulse imparted to the ball by the wall is
A2muBmuCzeroD−2muA ball of mass m strikes a rigid walJ with speed u and rebounds with the same speed. The impulse imparted to the ball by the wall is
 Amu sin30∘tB2mu sin30∘tCmu cos30∘tD2mu cos30∘t
Amu sin30∘tB2mu sin30∘tCmu cos30∘tD2mu cos30∘t
A ball of mass 1kg drops vertically on to the floor wit a speed of 25m/s. It rebounds with an initial velocity of 10m/s. What impulse acts on the ball during contact ?
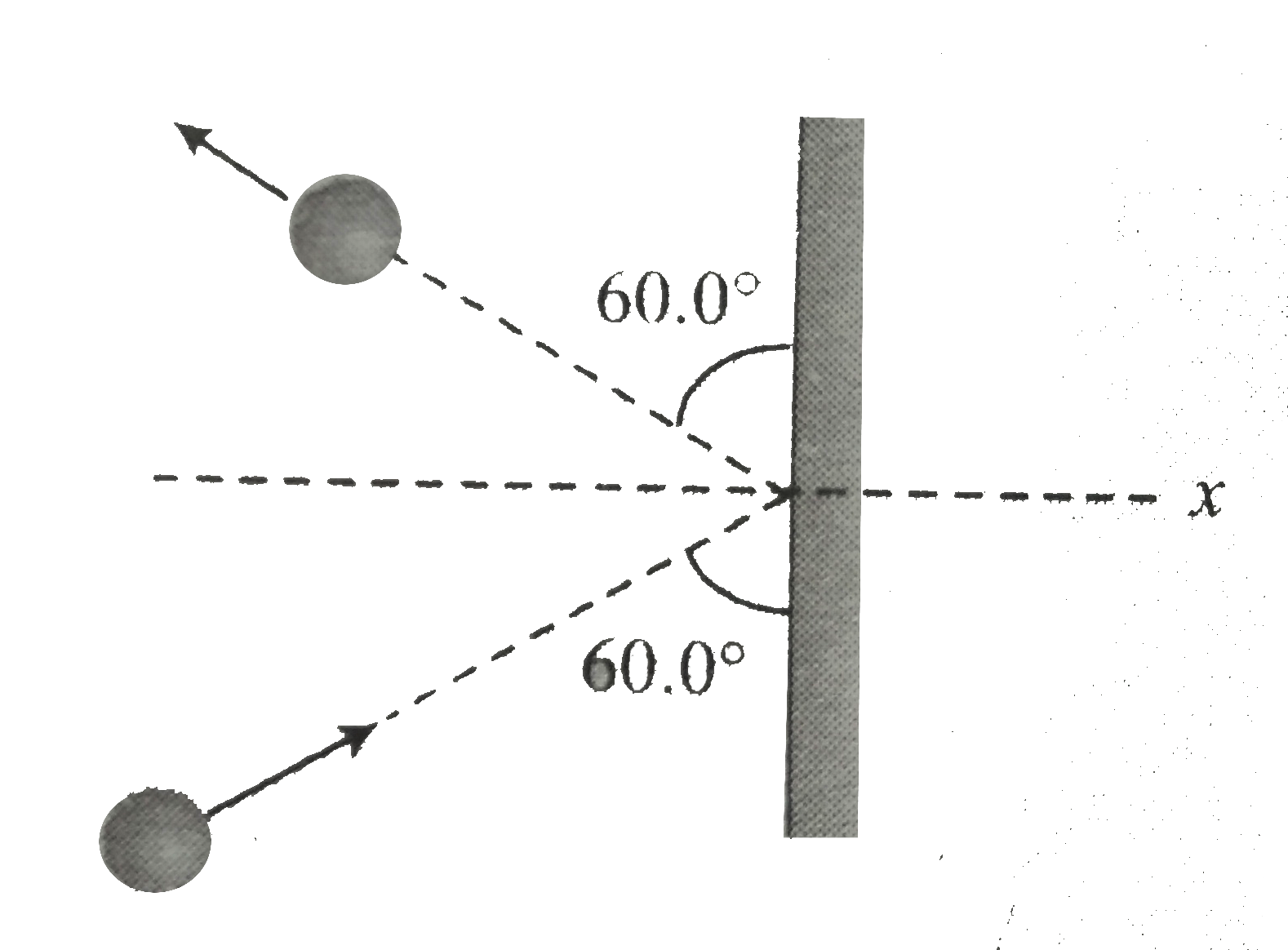
View SolutionA ball of mass 3kg moving with a speed of 100 m/s, strikes a wall at an angle 60∘ (as shown in figure). The ball rebounds at the same speed and remains in contact with the wall for 0.2 s, the force exerted by the ball on the wall is

View SolutionA 3-kg steel ball strikes a wall with a speed of 10.0ms−1 at an angle of 60.0∘ with the surfaces of the wall. The ball bounces off with the same speed and same angle. If the ball was in contact with the wall for 0.2s, find the average force exerted by the wall on the ball.
View SolutionTwo billiard balls each of mass 0.05 kg moving in opposite directions with speed 6ms−1 collide and rebound with the same speed. What is the impulse imparted to each ball due to the other ?
View SolutionA ball of mass m collides with a wall with speed v and rebounds on the same line with the same speed. If the mass of the wall is taken as infinite, then the work done by the ball on the wall is
View Solution
