Assume that a tunnel is dug across the earth (radius=R) passing through its centre. Find the time a particle takes to reach centre of earth if it is projected into the tunnel from surface of earth with speed needed for it to escape the gravitational field to earth.
The correct Answer is:t=sin−1(1√3)√Reg
To solve the problem of finding the time a particle takes to reach the center of the Earth when projected into a tunnel from the surface with the escape velocity, we will follow these steps:
Step 1: Understand the Escape Velocity
The escape velocity ve from the surface of the Earth is given by the formula:
ve=√2GMR
where G is the gravitational constant, M is the mass of the Earth, and R is the radius of the Earth.
Step 2: Gravitational Acceleration Inside the Earth
When the particle is at a distance r from the center of the Earth, the gravitational force acting on it can be described by:
g′=GM(r)r2
where M(r) is the mass of the Earth enclosed within radius r. According to the shell theorem, the effective gravitational force inside the Earth is:
g′=GMR3r
This shows that the gravitational acceleration inside the Earth varies linearly with distance from the center.
Step 3: Set Up the Equation of Motion
Using Newton's second law, we can relate the gravitational acceleration to the velocity v and position x:
dvdt=−GMR3x
where x is the distance from the center of the Earth.
Step 4: Express Velocity in Terms of Position
We can express the acceleration in terms of velocity and position:
vdvdx=−GMR3x
This can be rearranged to:
vdv=−GMR3xdx
Step 5: Integrate to Find Velocity as a Function of Position
Integrate both sides:
∫vdv=−GMR3∫xdx
This gives:
v22=−GMR3x22+C
Using the initial condition at the surface where x=R and v=ve:
v2e2=−GMR3R22+C
Solving for C gives:
C=v2e2+GM2R
Step 6: Find the Time to Reach the Center
Now, we can express the time t taken to reach the center:
dt=dxv
Substituting v from our previous integration:
t=∫0Rdx√v2e+GMR3x22
Step 7: Evaluate the Integral
This integral can be evaluated using appropriate substitutions or numerical methods. The final result will yield the time taken to reach the center of the Earth.
Final Result
After evaluating the integral, we find that the time t taken to reach the center of the Earth when projected with escape velocity is given by:
t=π2√Rg
where g is the acceleration due to gravity at the surface of the Earth.
The escape velocity ve from the surface of the Earth is given by the formula:
ve=√2GMR
where G is the gravitational constant, M is the mass of the Earth, and R is the radius of the Earth.
When the particle is at a distance r from the center of the Earth, the gravitational force acting on it can be described by:
g′=GM(r)r2
where M(r) is the mass of the Earth enclosed within radius r. According to the shell theorem, the effective gravitational force inside the Earth is:
g′=GMR3r
This shows that the gravitational acceleration inside the Earth varies linearly with distance from the center.
Using Newton's second law, we can relate the gravitational acceleration to the velocity v and position x:
dvdt=−GMR3x
where x is the distance from the center of the Earth.
We can express the acceleration in terms of velocity and position:
vdvdx=−GMR3x
This can be rearranged to:
vdv=−GMR3xdx
Integrate both sides:
∫vdv=−GMR3∫xdx
This gives:
v22=−GMR3x22+C
Using the initial condition at the surface where x=R and v=ve:
v2e2=−GMR3R22+C
Solving for C gives:
C=v2e2+GM2R
Now, we can express the time t taken to reach the center:
dt=dxv
Substituting v from our previous integration:
t=∫0Rdx√v2e+GMR3x22
This integral can be evaluated using appropriate substitutions or numerical methods. The final result will yield the time taken to reach the center of the Earth.
After evaluating the integral, we find that the time t taken to reach the center of the Earth when projected with escape velocity is given by:
t=π2√Rg
where g is the acceleration due to gravity at the surface of the Earth.
Topper's Solved these Questions
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 5 A (Previous Year Questions)
26 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 5 B (Previous Year Questions)
9 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 4 A (Conceptual Subjective Exercise)
14 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
46 Videos
Similar Questions
Assume that a tunnel is dug across the earth (radius=R) passing through its centre. Find the time a particle takes to cover the length of the tunnel if (a) it is projected into the tunnel with a speed of √(gR) (b) it is relased from a height R above the tunnel (c ) it is thrown vertically upward along the length of tunnel with a speed of √gR.
View SolutionThe value of escape speed from the surface of earth is
View Solution
Knowledge Check
The escape speed of a body on the earth's surface is 11.2kms−1. A body is projected with thrice of this speed. The speed of the body when it escape the gravitational pull of earth is
A11.2kms−1B22.4√2kms−1C22.4√2kms−1D22.4√3kms−1The escape velocity from the surface of the earth is (where RE is the radius of the earth )
A√2gREB√gREC2√gRED√3gRE
A tunnel is made across the earth passing through its center. A ball is dropped from a height h in the tunnel. The motion will be periodic with time period::
View SolutionAssume that a frictionless tunnel is made in the earth along its diameter, a particle in projected in the tunnel from the surface of the earth with an initial speed v0=√gR, where g is the acceleration due to gravity on the earth's surface & R is the radius of the earth, if time taken by the particle to reach the centre of the earth is πn√4Rg the value of n is?
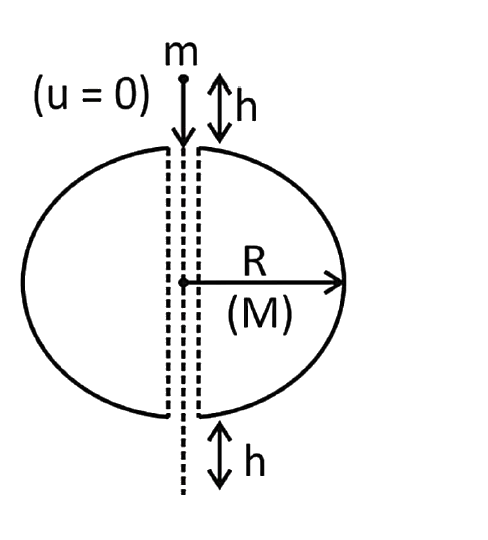
View SolutionA ball of mass m is dropped from a height h in a tunnel, made across the earth (mass = M, radius = R) passing through its center. If h<<R such that the motion of the particle through h can be considered uniformly accelerated at g, then the time period of the particle is
View SolutionA hole is drilled from the surface of earth to its centre. A particle is dropped from rest in the surface of earth in terms of its escape velocity on the surface of earth ve is :
View SolutionAssume that there is a smooth tunnel of depth R2 along a diameter of earth. A particle is projected from the bottom of tunnel with speed u. Find the minimum value of u so that the particle is able to escape the gravitational field of earth. M and R represents mass and radius of earth

View SolutionA tunnel has been dug through the centre of the earth and a ball is released in it. It will reach the other end of the tunnel after
View Solution
