State and prove Gauss's theorem in electrostatics.Solution in Punjabi
Gauss.s Theorem : It states that total electric flux through a closed surface is equal to 1ε0 times the magnitude of charge enclosed by it.
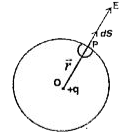
Proof : Consider a point charge + q is lying at O.
Draw a sphere of radius r with centre O which acts as a gussian surface.
Consider a point P on it where electric field intensity is given by
→E=14πε0qr2ˆr
where ˆr is a unit vector which gives the direction of electric field at point P.
Now consider a small of area dS around point P and −→dS is normal to the surface of area element.
Electric flux through the area element is given by
dϕ=→E.−→dS
dϕ=14πε0qr2ˆr−→dS
= 14πε0qr2.dSˆn
dϕ=14πε0qr2dS [∴ˆr.ˆn=1]
where ˆn is a unit vector normal to the surface of area element and gives the direction of −→dS Now flux over the whole closed surface is given by
∫dϕ=∫14πε0qr2dS
ϕ=14πε0qr2∫dS
ϕ=14πε0qr2(4πr2)
ϕ=qε0
which is the expression for Gauss.s theorem in electrostatic.
Topper's Solved these Questions
- GAUSS'S THEOREMBOOK - BETTER CHOICE PUBLICATIONCHAPTER - GAUSS'S THEOREMEXERCISE - LONG ANSWER TYPE QUESTIONS
9 Videos
- GAUSS'S THEOREMBOOK - BETTER CHOICE PUBLICATIONCHAPTER - GAUSS'S THEOREMEXERCISE - MOST EXPECTED NUMERICALS
4 Videos
- GAUSS'S THEOREMBOOK - BETTER CHOICE PUBLICATIONCHAPTER - GAUSS'S THEOREMEXERCISE - MOST EXPECTED QUESTIONS
10 Videos
- FORCE ON A CURRENTBOOK - BETTER CHOICE PUBLICATIONCHAPTER - FORCE ON A CURRENTEXERCISE - NUMERICAL PROBLEMS
10 Videos
- HUYGENS' PRINCIPLEBOOK - BETTER CHOICE PUBLICATIONCHAPTER - HUYGENS' PRINCIPLEEXERCISE - LONG ANSWER TYPE QUESTIONS
11 Videos
Similar Questions
State Gauss' law and using this law , derive an expression for the electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell.
View SolutionA box encloses an electric dipole of 3μCand−3C, if the dipole length is 6 cm, then find total electric flux associated with box.
View SolutionIs electric flux a scalar or a vector? Give the SI untis of electric flux.
View SolutionA currentof 5.0 A is flowing in eah of the two parallel conductors of infinite length separated by certain distance apart in vacuum in the same directions. Each conductor expereinces a force of 2×10−4Nm−1 due to the other. Find the separation between the two conductors.
View SolutionState Huygen's principle of propagation of light and prove the laws (Snell's law) of refraction on its basis.
View SolutionState and prove Gauss's law in electrostatics.
View SolutionState Gauss's Theorem in electrostatics and deduce coulomb's law from Gauss's theorem.
View SolutionState and prove Gauss's law in electrostatics.
View Solutionगॉस का प्रमेय लिखिए तथा ऐसे सिद्ध कीजिये।
View SolutionState and explain Gauss's theorem in Electrostatics.
View SolutionState and explain Gauss's law in electrostatics.
View SolutionDeduce coulomb's law in electrostatics from Gauss theorem. Or State and prove Gauss's law in electrostatics.
View SolutionState and prove Gauss's theorem in electrostatics.
View SolutionState Gauss's theorem in magnetism. How this differs from Gauss's electrostatics ? why is the difference in two cases ?
View SolutionState Gauss's theorem of electrostatics.
View SolutionState Gauss's Law of electrostatics.
View Solution
