[Punjabi] Consider a rectangular block of wood moving with a velocity

A gas enclosed in a container is heated up What is the effect on pressure?

What is the difference between gravity and gravitation?

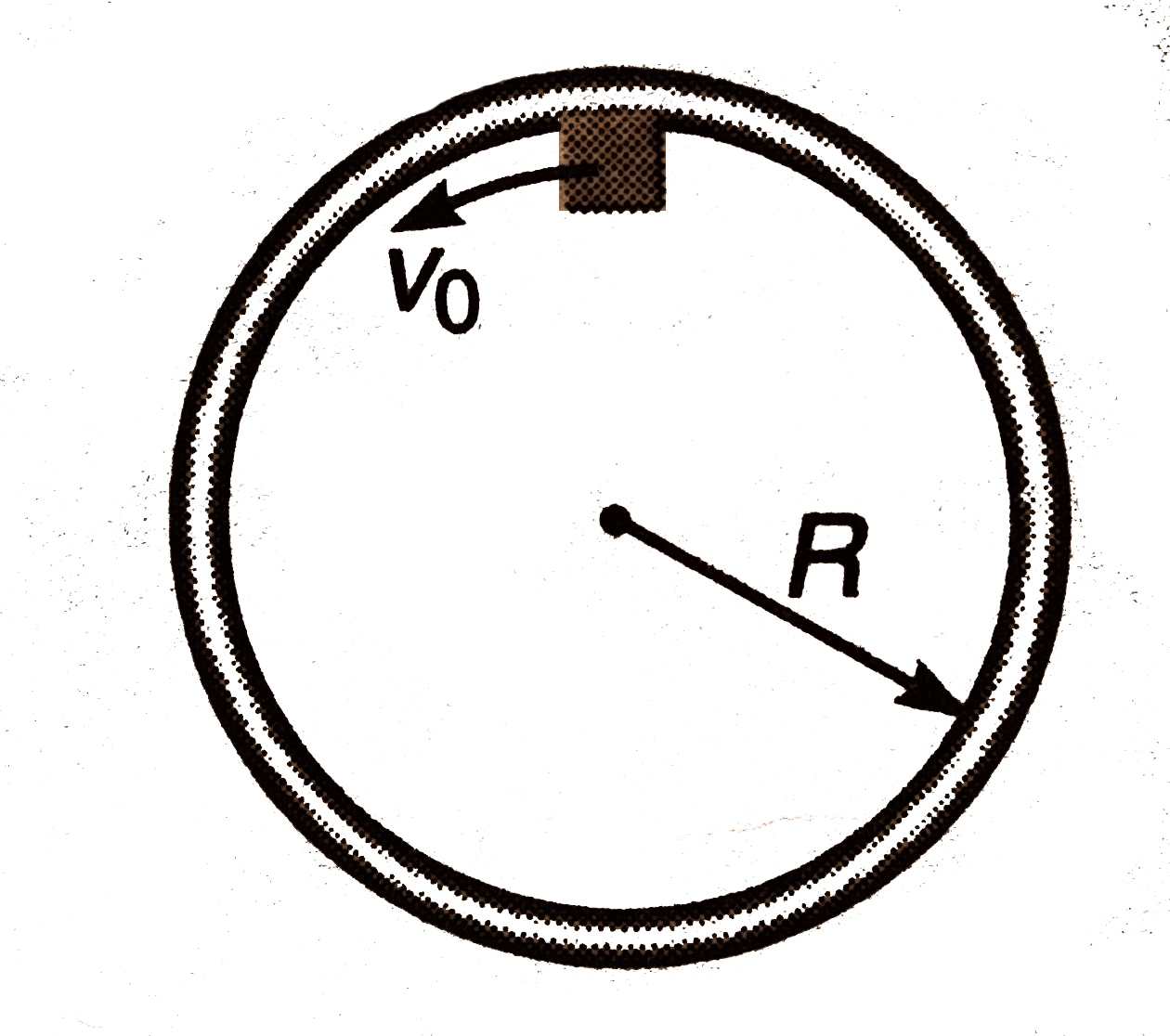
A block of mass $m$ slides 0n a frictionless table. It is constrained to move inside a ring of radius $R$ . At time $t = 0$ , block is moving along the inside of the ring (i.e. in the tangential direction) with velocity $v_{0}$ . The coefficient of friction between the block and the ring is $μ$ . Find the speed of the block at time $t$ .
ocr_image

04:03

View Solution

Consider a rectangular block of wood moving with a velocity $v_{0}$ in a gas at temperature T and mass density p. Assume the velocity is along x-axis and the are of cross-section of the block perpendicular to $v_{0}$ is A. show that the drag force on the block is $4 r A v_{0} \sqrt{\frac{k T}{m}}$ where,m is the mass of the gas molecule.

05:48

View Solution

A block of mass m is pushed towards the movable wedge of mass M and height h , with a velocity v_(0) . All surfaces are smooth . The minimum value of v_(0) for which the block will reach the top of the wedge is

02:48

View Solution

A block A of mass m connected with a spring of force constant k is executing SHM.The displacement time equation of the block is x= x_(0) = a sinomegat . An indentical block B moving towards negative x -axis with velocity v_(0) collides elastically with block A at time t=0. Then

View Solution

Consider a rectangular block of wood moving with a velocity v_(0) in a gas at temperature T and mass density p. Assume the velocity is along x-axis and the are of cross-section of the block perpendicular to v_(0) is A. show that the drag force on the block is 4rAv_(0)sqrt((kT)/(m)) where,m is the mass of the gas molecule.