A block A of mass m connected with a spring of force constant k is executing SHM.The displacement time equation of the block is x=x0=asinωt. An indentical block B moving towards negative x -axis with velocity v0 collides elastically with block A at time t=0. Then
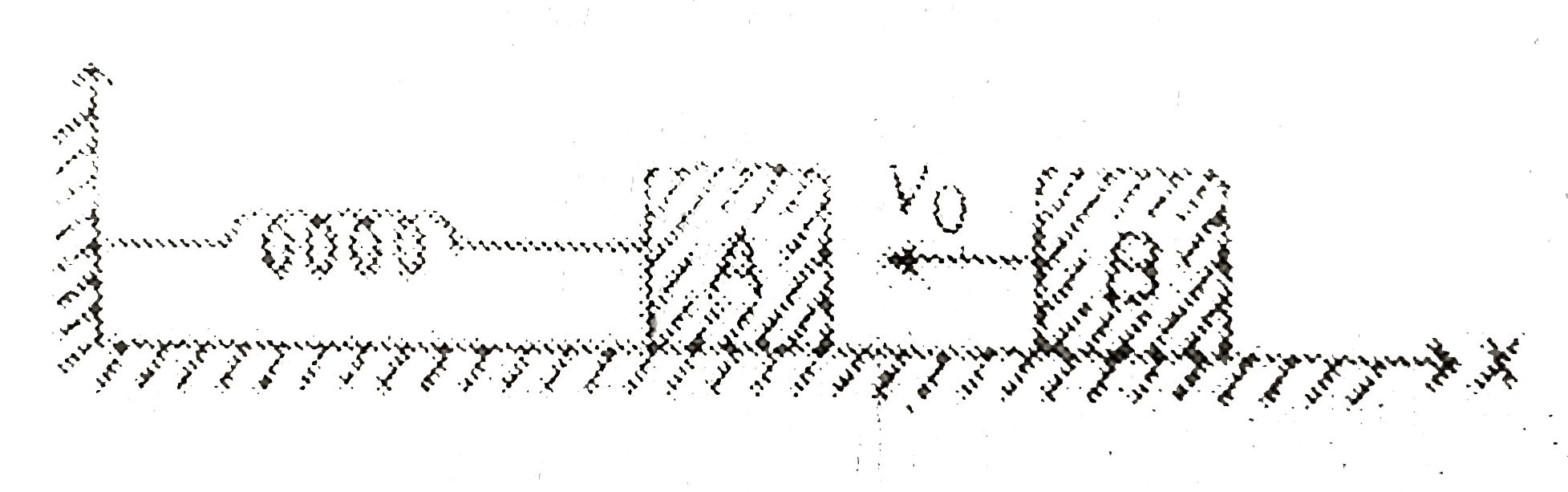
displacement time equation of A after collision will be x=x0−v0√mksinωt
displacement time equation of A after collision will be x=x0+v0√mksinωt
Velocity of b just after collision will be a ω towards positive x-direction.
velocity of B just after collision will be v0 towards positive x-direction.
The correct Answer is:A, C, D
Step by step video, text & image solution for A block A of mass m connected with a spring of force constant k is executing SHM.The displacement time equation of the block is x=x_(0) = a sinomegat. An indentical block B moving towards negative x -axis with velocity v_(0) collides elastically with block A at time t=0. Then by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBook:DC PANDEYChapter:SIMPLE HARMONIC MOTIONExercise:Comprehension types
Explore 18 Videos
- SIMPLE HARMONIC MOTIONBook:DC PANDEYChapter:SIMPLE HARMONIC MOTIONExercise:Matrix matching type questions
Explore 13 Videos
- SIMPLE HARMONIC MOTIONBook:DC PANDEYChapter:SIMPLE HARMONIC MOTIONExercise:JEE Advanced
Explore 34 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBook:DC PANDEYChapter:SEMICONDUCTORS AND ELECTRONIC DEVICESExercise:More than One Option is Correct
Explore 3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBook:DC PANDEYChapter:SOLVD PAPERS 2017 NEET, AIIMS & JIPMERExercise:Solved paper 2018(JIPMER)
Explore 38 Videos
Similar Questions
A block of mass M connected to an ideal spring of force constant k, is placed on a smooth surface. The block is pushed to the left so as to compress the spring by a length A and then it is released. The block hits an elastic wall at a distance 3A2 from its point of release. Assume the collision to be instantaneous.
(a) Calculate the time required by the block to complete one oscillation
(b) Draw the velocity - time graph for one oscillation of the block.
(c) Find the value of k for which average force experienced by the wall due to repeated hitting of the block is F0.
View SolutionA block of mass m is connected to another .block of mass M by a massless spring of spring constant k. A constant force f starts action as shown in figure, then:

View Solution
Knowledge Check
A block A of mass m connected with a spring of force constant k is executing SHM. The displacement time equation of the block is x=x0+asinωt. An identical block B moving towards negative x-axis with velocity vo collides elastically with block A at time t = 0. Then,
 Adisplacement time equation of A after collision will be x=x0−v0√mksinωtBdisplacement time equation of A after collision will be x=x0+v0√mksinωtCvelocity of B just after collision will be aw towards positive x-direction.Dvelocity of B just after collision will be vo towards positive x-direction.
Adisplacement time equation of A after collision will be x=x0−v0√mksinωtBdisplacement time equation of A after collision will be x=x0+v0√mksinωtCvelocity of B just after collision will be aw towards positive x-direction.Dvelocity of B just after collision will be vo towards positive x-direction.A block is suspended by an ideal spring of force constant force F and if maximum displacement of block from its initial mean position of rest is x0 then
Aincrease in energy stored in spring is kx20Bx0=3F2kCx0=2FkDwork done by applied force F is Fx0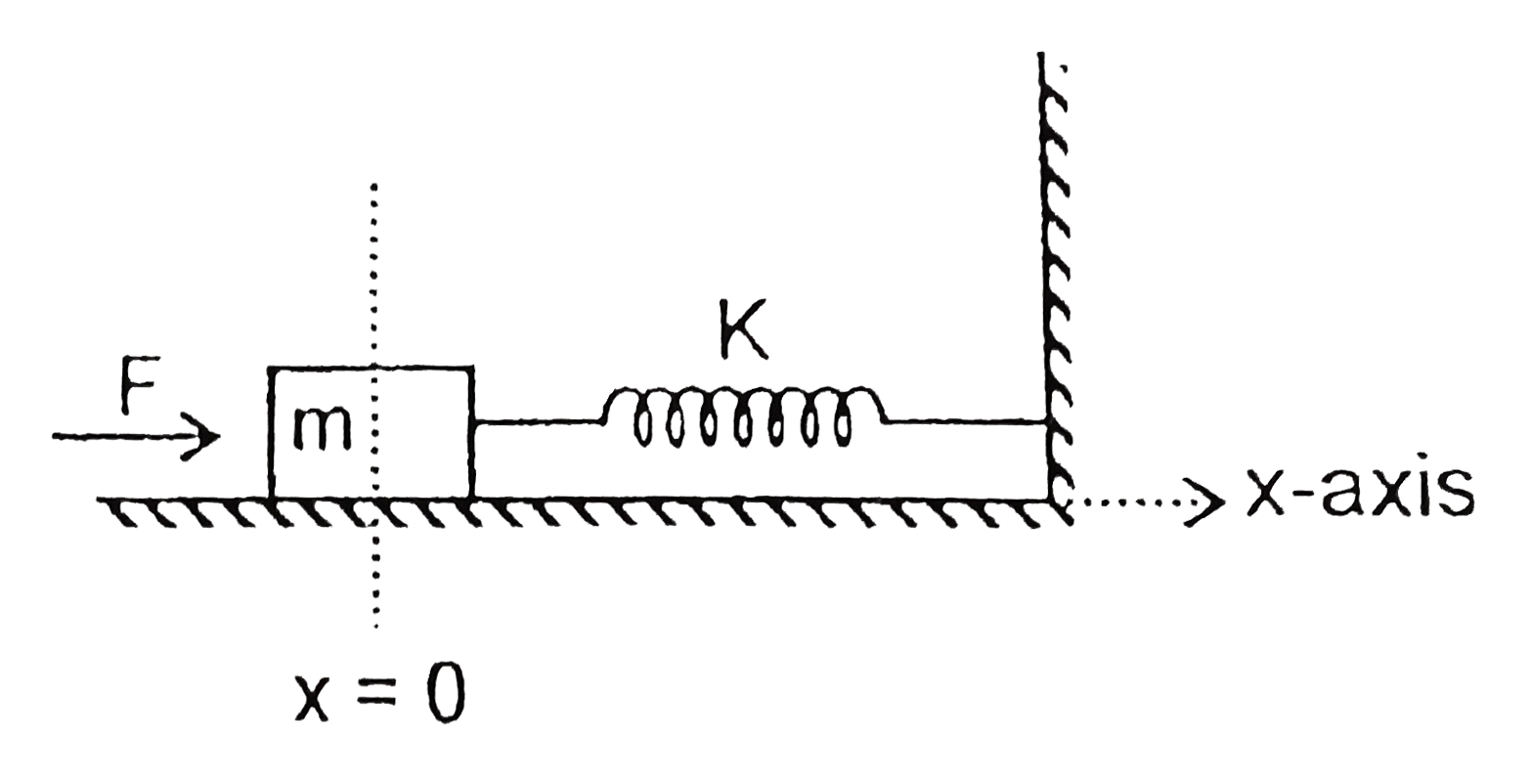
A block of mass m connected to a spring of stiffness k is placed on horizontal smooth surface. When block is at x=0, spring is in natural length. The block is also subjected to a force F=k|x|∘ thenAMotion of block is SHMBTime period of motion is π√mk[√2+√23]CTime period of motion is π√mk[1+√32]DSum of potential energy and kinetic energy of spring block system is maximum at right extreme.
A block of mass 0.18kg is attached to a spring of force constant 2N/m The coefficient of friction between the block and the force is 0.1 insitially its block is at rest and the block as spring is an streched , As impalse is given to the block as shown in the figure . The block sides a distance of 0.06 in and comes to the first time . The initial velocity of the for blocks is mis V=N10 then N is .
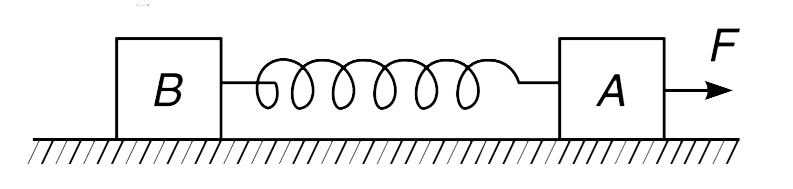
View SolutionTwo blocks A and B, each of mass m, are connected by a spring of force constant K. Initially, the spring is in its natural length. A horizontal constant force F starts acting on block A at time t=0 and at time t , the extension in the spring is seen to be l. What is the displacement of the block A in time t ?
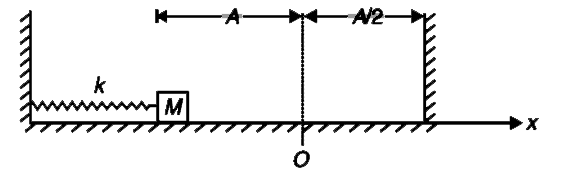
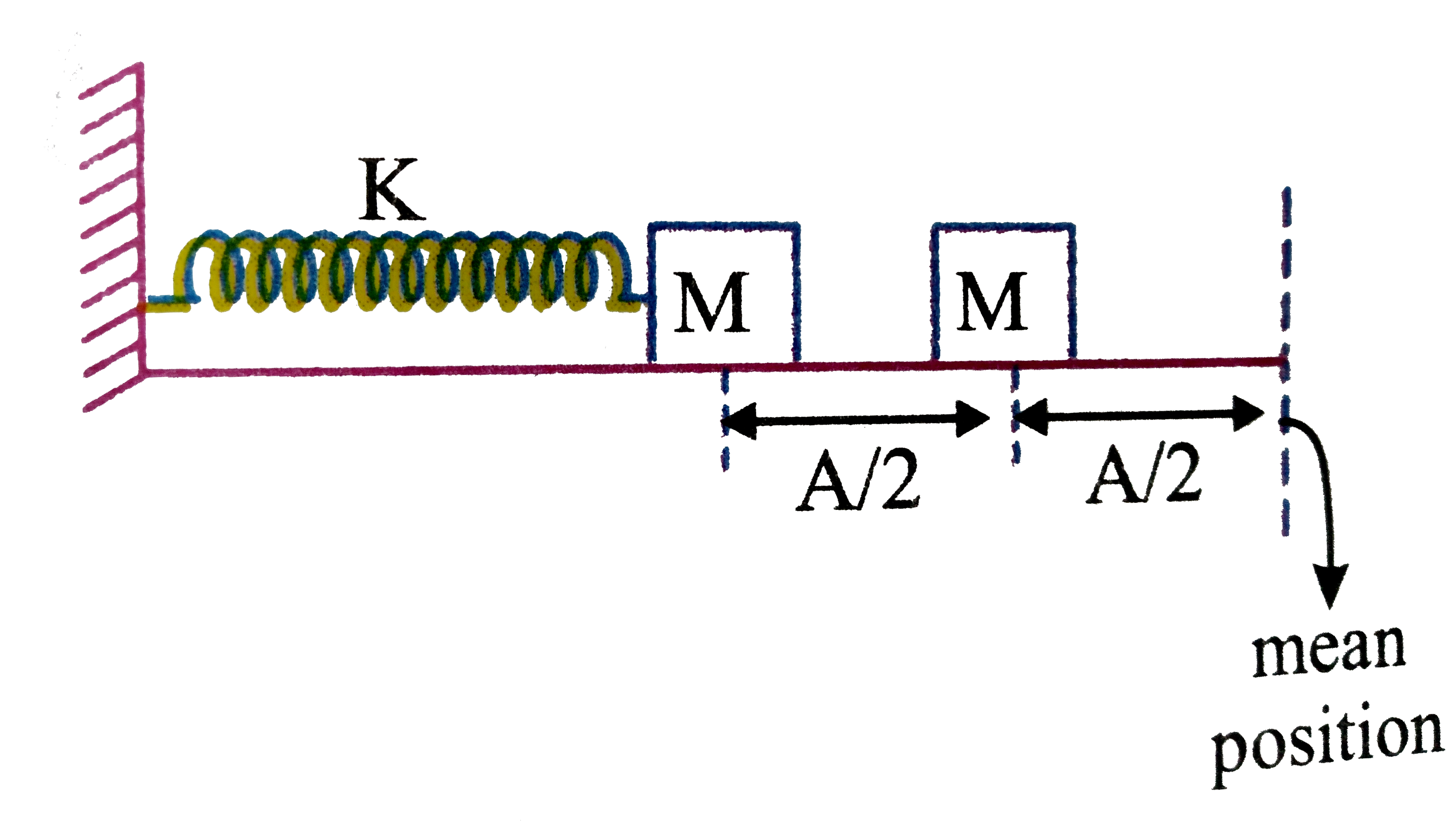
View SolutionA block of mass M is connected to a spring of force constant k and is placed on a smopth horizontal surface. The block is displaced and compressed the spring by ''A''. The block is left free to move form this position, when the block is at distance A/2 form mean position to collides elastically with the identical block. tme to be taken by block to move from exterme position to mean position is....
View SolutionA block of mass m is connected to two indentical springs of spring constant k which are in turn connected to fixed supports as shown in the figure. Find the time period for small oscillations of the block.

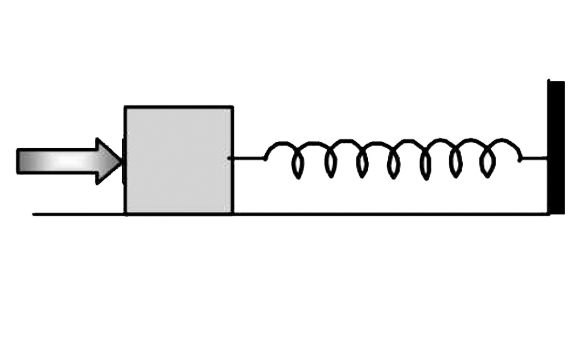
View SolutionA block of mass 1kg is connected with a massless spring of force constant 100N/m. At t=0, a constant force F=10N is applied on the block. The spring is in its netural length at t=0. The speed of particel at x=6cm form mean position is
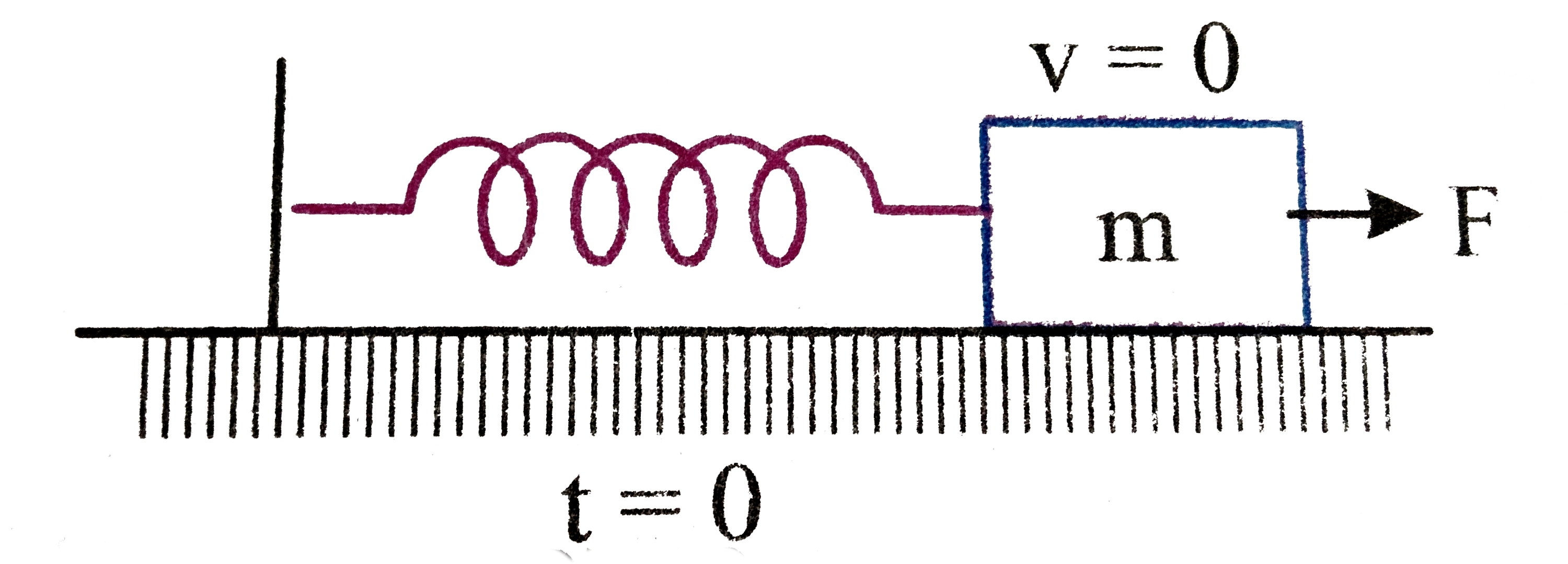
View Solution
