If ∣∣→A∣∣=2 and ∣∣→B∣∣=4∣∣, then match the relation in column I with the angle θ between →A and →B in column II .
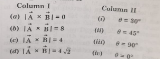 Solution in Punjabi
Solution in Punjabi
Step by step video & image solution for If |vecA|=2 and |vecB|=4|, then match the relation in column I with the angle theta between vecA and vecB in column II . by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Is it necessary to mention the direction of a vector having zero magnitude?
View SolutionName the device used for measuring the mass of atoms and molecules.
View SolutionIn which of the following examples of motion, can the body be considered approximately a point object:
View SolutionThe two vectors vecA and vecB are drawn from a common point and vecC = vecA + vecB . In column- I are given the conditions regarding the magnitudes of vecA, vecB and vecC as A, B, C respectively. Column- II gives the angle between the vectors vecA and vecB . Match them.
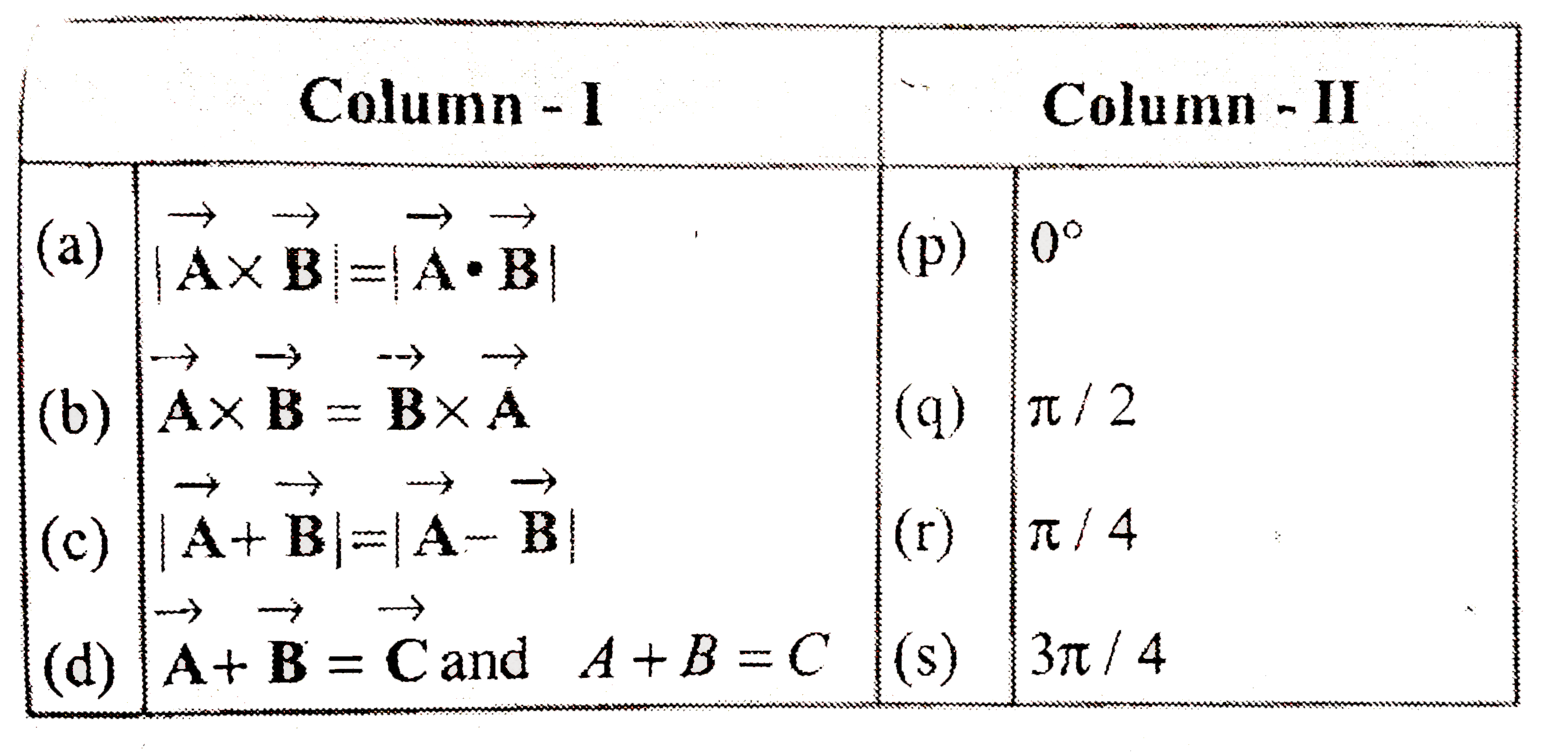
View SolutionColumn-I gives operation of vectors →Aand→B and column- II gives the angle (θ) between →A and →B (→A and →B are not zero vectors ).
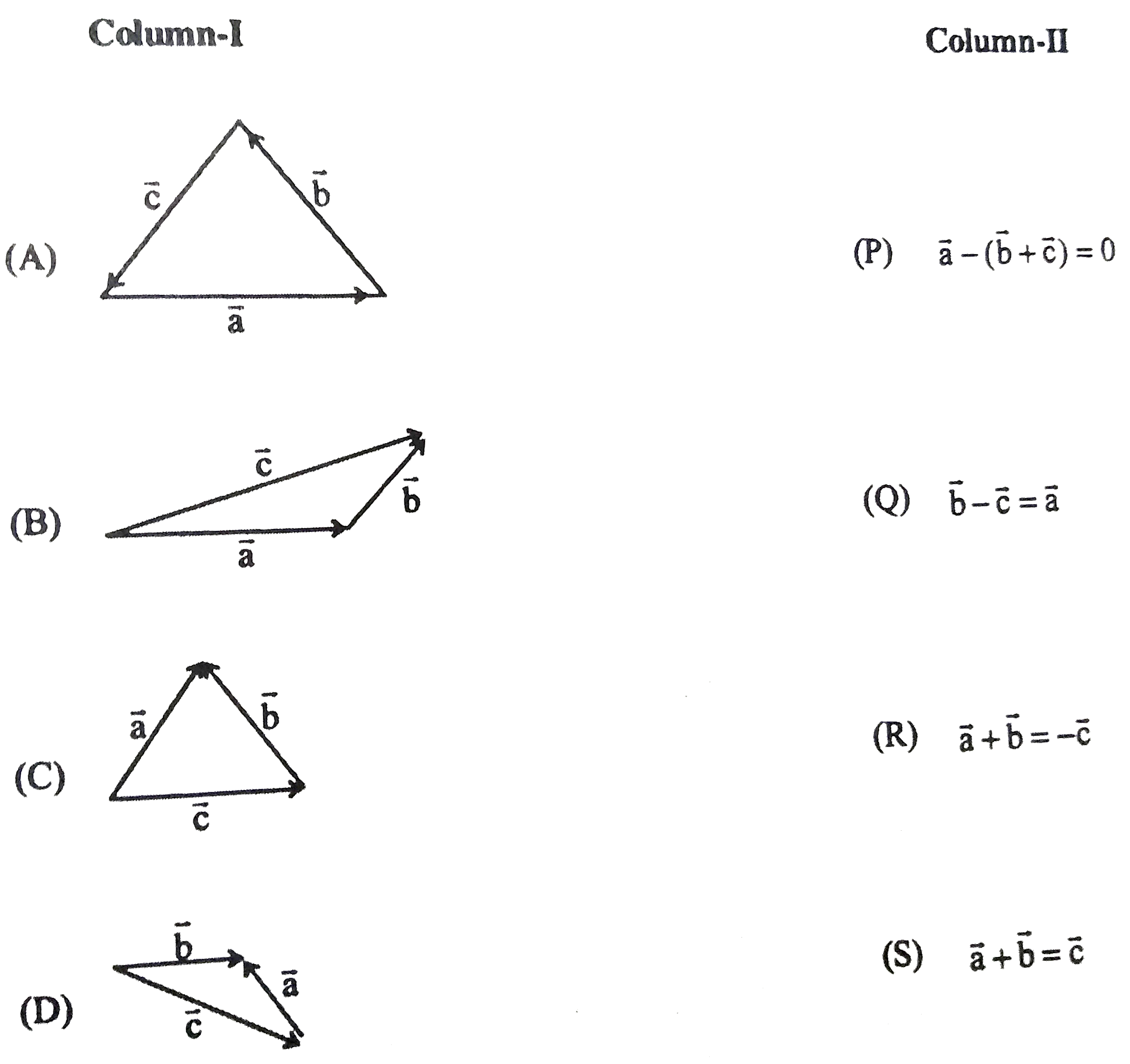
View SolutionColumn-I show vector diagram relating three vectors →a,→b and →c. Match the vector equation in column -II, with vector diagram in column-I:
View SolutionIf θ is the angle between the unit vectors →aand→b, then prove that
i. cos(θ2)=12∣∣→a+→b∣∣
ii.sin(θ2)=12∣∣→a−→b∣∣
View SolutionIf θ is the angle between the unit vectors →aand→b, then prove that
i. cos(θ2)=12∣∣→a+→b∣∣
ii.sin(θ2)=12∣∣→a−→b∣∣
View SolutionIf →a,→b are unit vectors and θ is the angle between them, show that
tanθ2=∣∣→a−→b∣∣∣∣→a+→b∣∣
View SolutionMatch the statements given in column-I with statements given in column-II
Column - IColumn - II(A) If∣∣→A∣∣=∣∣→B∣∣and∣∣→A∣∣ then(p)90∘ angle between →Aand→B is(B) Magnitude of resultant of two forces(q)120∘∣∣→F1∣∣=8Nand∣∣→F2∣∣=4N may be(C) Angle between→A=2ˆi+2ˆj&→B=3ˆk is(r)12N(D) Magnitude of resultant of vectors(s)√14→A=2ˆi+ˆj&→B=3ˆk is
View SolutionIf →a and →b are unit vector and θ is the angle between then, then ∣∣∣→a−→b2∣∣∣ is:
View SolutionIf θ is the angled between →aand→band∣∣→a×→b∣∣=∣∣→a.→b∣∣, then θ is equal to
View SolutionThe angle between two vectors →A=→B is θ. The resultant of →Aand→B making an angle θ2 with →A. Then:
View Solution→a,→b ஆகியவை அலகு வெக்டர்கள் மற்றும் θ என்பது இவற்றிற்கு இடைப்பட்ட கோணம் எனில் , tanθ2=∣∣→a−→b∣∣∣∣→a+→b∣∣ எனக் காட்டுக.
View SolutionIf |vecAxxvecB|=vecA*vecB , then angle between vecA and vecB" is "theta = …...
View Solution
