A heavy particle of weight w, attached to a fixed point by a light inextensible string describes a circle in a vertical plane. The tension in the string has the values nw and mw respectively when the particle is at the highest and lowest points in the path. Then :
m+n=6
mn=2
m−n=−6
n−m=−6
The correct Answer is:D
To solve the problem, we need to analyze the forces acting on the particle at the highest and lowest points of its circular motion and apply the principles of circular motion and energy conservation.
1. Identify the Forces at the Highest Point:
At the highest point of the circular path, the forces acting on the particle are:
- Weight (downward) = w
- Tension (upward) = T1=nw
The net centripetal force required to keep the particle moving in a circle is provided by the difference between the tension and the weight:
T1−w=mv21L
Substituting T1:
nw−w=mv21L
Simplifying gives:
(n−1)w=mv21L(1)
2. Identify the Forces at the Lowest Point:
At the lowest point of the circular path, the forces acting on the particle are:
- Weight (downward) = w
- Tension (upward) = T2=mw
The net centripetal force required is given by:
T2−w=mv22L
Substituting T2:
mw−w=mv22L
Simplifying gives:
(m−1)w=mv22L(2)
3. Apply the Work-Energy Theorem:
The particle travels a vertical distance of 2L (from the highest point to the lowest point). The work done by gravity is:
W=−w⋅2L=−2wL
The change in kinetic energy is:
ΔKE=12m(v22−v21)
According to the work-energy theorem:
−2wL=12m(v22−v21)
Rearranging gives:
v22−v21=−4wLm(3)
4. Relate the Velocities:
From equations (1) and (2), we can express v21 and v22:
- From (1): v21=(n−1)wLm
- From (2): v22=(m−1)wLm
5. Substituting into the Work-Energy Equation:
Substitute v21 and v22 into equation (3):
(m−1)wLm−(n−1)wLm=−4wLm
Simplifying gives:
(m−n)wLm=−4wLm
Canceling wL from both sides (assuming wL≠0):
m−n=−4(4)
6. Final Rearrangement:
Rearranging equation (4) gives:
m−n=6
Conclusion:
Thus, the correct relationship between m and n is:
m−n=6
At the highest point of the circular path, the forces acting on the particle are:
- Weight (downward) = w
- Tension (upward) = T1=nw
T1−w=mv21L
Substituting T1:
nw−w=mv21L
Simplifying gives:
(n−1)w=mv21L(1)
At the lowest point of the circular path, the forces acting on the particle are:
- Weight (downward) = w
- Tension (upward) = T2=mw
T2−w=mv22L
Substituting T2:
mw−w=mv22L
Simplifying gives:
(m−1)w=mv22L(2)
The particle travels a vertical distance of 2L (from the highest point to the lowest point). The work done by gravity is:
W=−w⋅2L=−2wL
The change in kinetic energy is:
ΔKE=12m(v22−v21)
According to the work-energy theorem:
−2wL=12m(v22−v21)
Rearranging gives:
v22−v21=−4wLm(3)
From equations (1) and (2), we can express v21 and v22:
- From (1): v21=(n−1)wLm
- From (2): v22=(m−1)wLm
Substitute v21 and v22 into equation (3):
(m−1)wLm−(n−1)wLm=−4wLm
Simplifying gives:
(m−n)wLm=−4wLm
Canceling wL from both sides (assuming wL≠0):
m−n=−4(4)
Rearranging equation (4) gives:
m−n=6
Thus, the correct relationship between m and n is:
m−n=6
Topper's Solved these Questions
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:Level - 1 PARAGRAPH QUESTIONS
Explore 3 Videos
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:LEVEL - 2
Explore 48 Videos
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:LEVEL - 0 - LONG ANSWER TYPE
Explore 5 Videos
- ELECTROSTATICSBook:VMC MODULES ENGLISHChapter:ELECTROSTATICSExercise:JEE Advanced (Archive)
Explore 89 Videos
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:JEE ADVANCED (ARCHIVE )
Explore 111 Videos
Similar Questions
A heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizontally with speed (gl) . Then the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string equal the weight of the particle-
View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizonally with speed √gl . Find the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string is equal to the weight of the particle.
View SolutionA particle is projected so as to just move along a vertical circle of radius r. The ratio of the tension in the string when the particle is at the lowest and highest point on the circle is infinite
View SolutionA particle tied to a string describes a vertical circular motion of radius r continually. If it has a velocity √3gr at the highest point, then the ratio of the respective tensions in the string holding it at the highest and lowest points is
View SolutionA 2 kg stone tied at the end of a string of l m len"gt"h, is whirled along a vertical circle at a constant speed of 4ms−1. The tension in the string has a value of 52 N when the stone is
View SolutionA particle of mass m attached to an inextensible light string is moving in a vertical circle of radius r. The critical velocity at the highest point is v0 to complete the vertical circle. The tension in the string when it becomes horizontal is
View SolutionA particle of mass m is attached to a string of length L and given velocity √10gL in the horizontal direction at the lowest point. Find tension in the string when the particle is at (i) lowest position (ii) highest position,
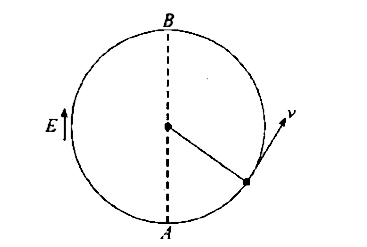
View SolutionA particle of mass m and charge Q is attached to a string of length l. It is whirled in a vertical circle in the region of an electric field E as shown in the figure-5.105.What is the speed given to the particle at the point B,so that tension in the string when the particle is at A is ten times the weight of the particle?
View SolutionA particle of mass m attached to a string of length l is descending circular motion on a smooth plane inclined at an angle α with the horizontal. For the particle to reach the highest point its velocity at the lowest point should exceed.
View SolutionA particle, held by a string whose other end is attached to a fixed point C, moves in a circle on a horizontal frictionless surface. If the string is cut, the angular momentum of the particle about the point C
View Solution