Two springs A and B(kA=2kB) are stretched by applying forces of equal magnitudes at the force ends. If the energy stored in A is E, that in B is
(a)E/2
(b)2E
(c)E
(d)E/4
The correct Answer is:B
Let kA=2kB=K⇒F=kAxA=kBxB⇒xA=FK&xB=2FK
UA−12kA×x2A=12K(FK)2=F2K=E , UB=12kBx2B=12kBxB^2=12K2(2FK)2=F2K=2E.
Topper's Solved these Questions
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - Level - 1 PARAGRAPH QUESTIONS
3 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - LEVEL - 2
48 Videos
- ENERGY & MOMENTUMBOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - LEVEL - 0 - LONG ANSWER TYPE
5 Videos
- ELECTROSTATICSBOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)
89 Videos
- GASEOUS STATE & THERMODYNAMICSBOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )
111 Videos
Similar Questions
Two springs A and B (KA=2KB) are stretched by same suspended weights then ratio of workdone in stretching is -
View SolutionTwo springs have force constants kA such that kB=2kA. The four ends of the springs are stretched by the same force. If energy stored in spring A is E, then energy stored in spring B is
View Solution
Knowledge Check
Two springs A and B are identical except that A is stiffer than B i.e., kA>kB. If the two springs are stretched by the same force, then
Amore work is done on B i.e., WB>WABmore work is done on A i.e., WA>WBCwork done on A and B are equalDwork done depends upon the way in which they are stretchedA long spring is stretched by 2 cm, its potential energy is U. IF the spring is stretched by 10 cm, the potential energy stored in it will be
AU/25BU/5C5 UD25 U
Two springs have their force constants in the ratio of 3:4. Both the springs are stretched by applying equal force F. If elongation in first spring is x then elogation is second spring is
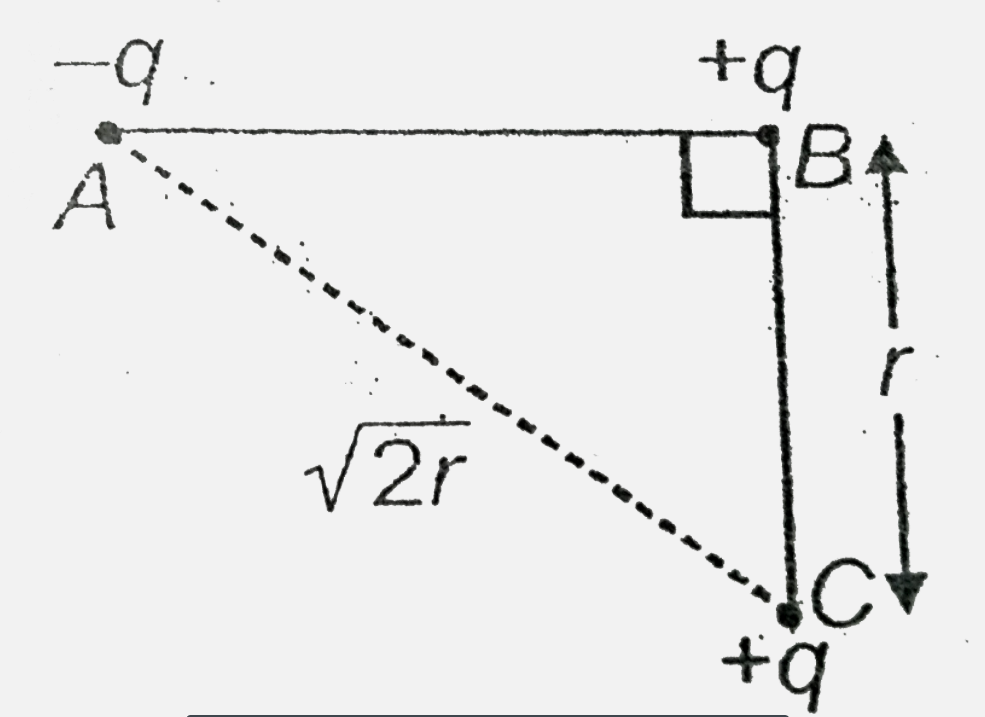
View SolutionIn the following figure, if magnitude of forces between B and C is F, then magnitude of force between A and B is
View SolutionA spring of force constant k extends by a length X on loading . If T is the tension in the spring then the energy stored in the spring is
View SolutionForce constants K1andK2 of two springs are in the ratio 5:4. They are stretched by same length. If potential energy stored in one spring is 25 J then potential energy stored in second spring is
View SolutionTwo springs with spring constants K1=1500N/mand m K2=3000N/m are stretched by the same force. The ratio of potential energy stored in spring will be
View SolutionThe potential energy of a long spring when stretched by 2 cm is U. If the spring is stretched by 8 cm the potential energy stored in it is:-
View Solution
