A particle of mass (m) is attached to a spring (of spring constant k) and has a natural angular frequency ω0. An external force F(t) proportional to cosωt (ω≠ω0) is applied to the oscillator. The time displacement of the oscillator will be proportional to.
mω20−ω2
1m(ω20−ω2)
1m(ω20+ω2)
mω20+ω2
The correct Answer is:B
To solve the problem step by step, we will analyze the system of a mass attached to a spring under the influence of an external force.
Step 1: Understand the System
We have a mass m attached to a spring with a spring constant k. The natural angular frequency of the system is given by:
ω0=√km
An external force F(t) is applied, which is proportional to cos(ωt), where ω≠ω0.
Step 2: Write the Net Force Equation
The net force acting on the mass when displaced by x from the mean position can be expressed as:
Fnet=Fspring−Fexternal
The force exerted by the spring is given by Hooke's law:
Fspring=−kx
The external force can be represented as:
Fexternal=F0cos(ωt)
where F0 is a constant of proportionality.
Thus, the net force can be written as:
Fnet=−kx−F0cos(ωt)
Step 3: Set Up the Equation of Motion
Using Newton's second law, we can write:
md2xdt2=−kx−F0cos(ωt)
Rearranging gives:
md2xdt2+kx=−F0cos(ωt)
Step 4: Substitute Natural Frequency
Substituting k=mω20 into the equation, we have:
d2xdt2+ω20x=−F0mcos(ωt)
Step 5: Identify the Type of Motion
This is a second-order linear differential equation with a non-homogeneous term. The solution to this equation will consist of a complementary (homogeneous) solution and a particular solution.
Step 6: Find the Particular Solution
Since the external force is of the form cos(ωt), we can assume a particular solution of the form:
xp=Acos(ωt)+Bsin(ωt)
Substituting xp into the differential equation will allow us to solve for the coefficients A and B.
Step 7: Solve for Coefficients
After substituting and simplifying, we find that the amplitude of the oscillation due to the external force is proportional to:
xp∝1ω20−ω2
This indicates that the displacement x is inversely proportional to ω20−ω2.
Final Answer
Thus, the time displacement of the oscillator will be proportional to:
x∝1ω20−ω2
We have a mass m attached to a spring with a spring constant k. The natural angular frequency of the system is given by:
ω0=√km
An external force F(t) is applied, which is proportional to cos(ωt), where ω≠ω0.
The net force acting on the mass when displaced by x from the mean position can be expressed as:
Fnet=Fspring−Fexternal
The force exerted by the spring is given by Hooke's law:
Fspring=−kx
The external force can be represented as:
Fexternal=F0cos(ωt)
where F0 is a constant of proportionality.
Fnet=−kx−F0cos(ωt)
Using Newton's second law, we can write:
md2xdt2=−kx−F0cos(ωt)
Rearranging gives:
md2xdt2+kx=−F0cos(ωt)
Substituting k=mω20 into the equation, we have:
d2xdt2+ω20x=−F0mcos(ωt)
This is a second-order linear differential equation with a non-homogeneous term. The solution to this equation will consist of a complementary (homogeneous) solution and a particular solution.
Since the external force is of the form cos(ωt), we can assume a particular solution of the form:
xp=Acos(ωt)+Bsin(ωt)
Substituting xp into the differential equation will allow us to solve for the coefficients A and B.
After substituting and simplifying, we find that the amplitude of the oscillation due to the external force is proportional to:
xp∝1ω20−ω2
This indicates that the displacement x is inversely proportional to ω20−ω2.
Thus, the time displacement of the oscillator will be proportional to:
x∝1ω20−ω2
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION Book:VMC MODULES ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:7-previous year question
Explore 46 Videos
- SIMPLE HARMONIC MOTION Book:VMC MODULES ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:LEVEL (2)
Explore 40 Videos
- ROTATIONAL MOTION Book:VMC MODULES ENGLISHChapter:ROTATIONAL MOTION Exercise:JEE Advanced (Archive) (True/False Type)
Explore 3 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBook:VMC MODULES ENGLISHChapter:SYSTEM OF A PARTICLES & ROTATIONAL MOTIONExercise:IN-CHAPTER EXERCISE F
Explore 10 Videos
Similar Questions
A particle of mass m is fixed to one end of a massless spring of spring constant k and natural length l0. The system is rotated about the other end of the spring with an angular velocity ω ub gravity-free space. The final length of spring is
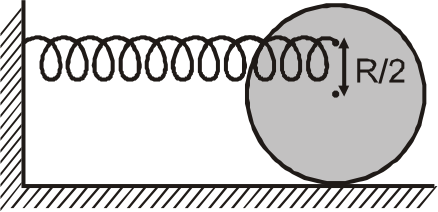
View SolutionA uniform disc of mass m is attached to a spring of spring constant k as shown in figure and there is sufficient friction to prevent slipping of disc. Time period of small oscillations of disc is:
View SolutionA body of mass 4 kg is made to oscillate on a spring of force constant 10 N/m. Calculate (i) angular frequency (2) frequency of vibration and (3) time period of vibration.
View SolutionWhen a particle of mass m is attached to a vertical spring of spring constant k and released, its motion is described by y(t)=y0sin2ωt, where 'y' is measured from the lower end of unstretched spring. Then ω is :
View SolutionA constant force(F) is applied on a stationary particle of mass 'm'. The velocity attained by the particle in a certain displacement will be proportional to
View SolutionA constant force (F) is applied on a stationary particle of mass m, the velocity attained by the particle in a certain interval of time will be proportional to
View SolutionA particles of mass m is fixed to one end of a light spring of force constant k and unstreatched length l. the system is rotated about the other end of the spring with an angular velocity ω in gravity free space. The increase in length of the spring is
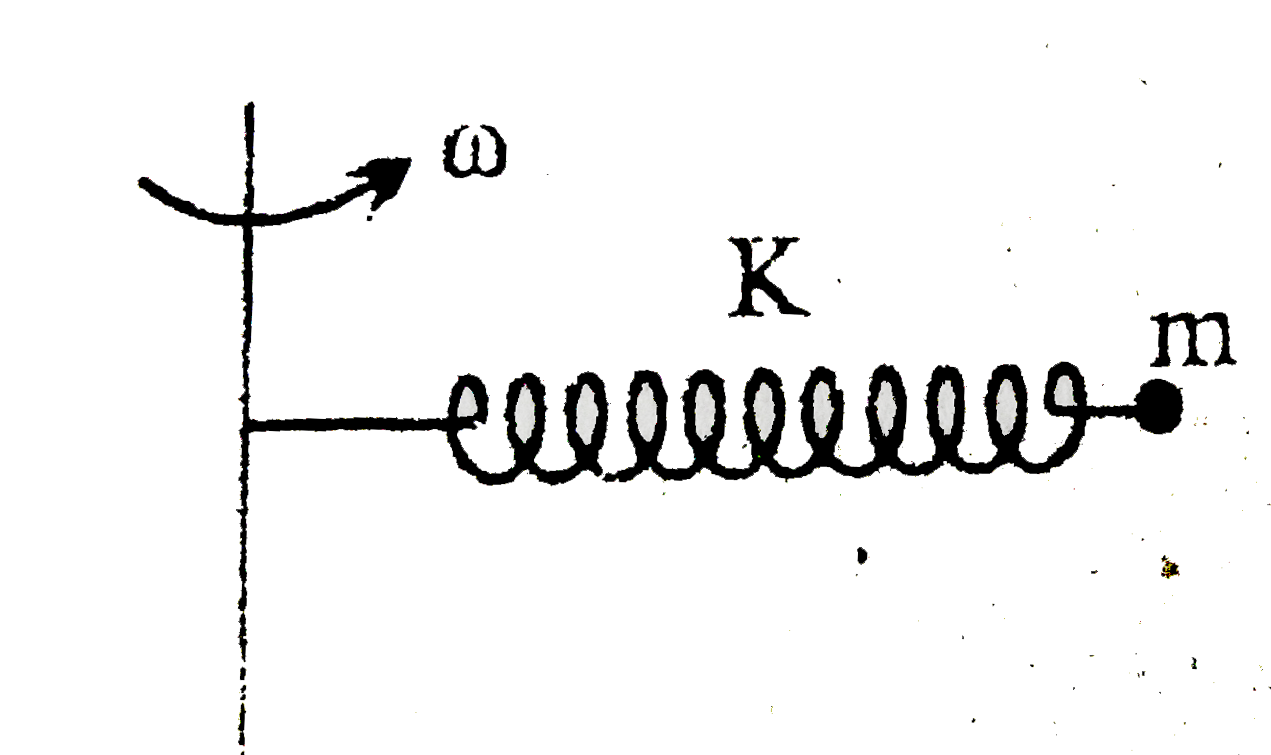
View SolutionA block of mass 0.01 kg is attached to a spring of force constant 10−1Nm−1 . If the energy of oscillation is 10−5 J, find the angular frequency and amplitude of oscillation.
View SolutionA constant force(F) is applied on a stationary particle of mass 'm'. the velocity attained by the particle in a certain interval of time will be proportional to
View SolutionA force F is exerted on a body of mass M, which is connected to a spring (spring constant k). The body has a constant acceleration a. Calculate the work done by the external force in moving the body by a distance D.
View Solution