In the figure shown, the string and pulley are massless. The inclined plane is fixed and has coefficients of friction μs=0.25 and μk=0.2 . Consider two cases:
Case I : m1=2kgm2=1.5kgθ=37∘,Case II:m1=3kgm2=2kgθ=53∘
Take g=10m/s2,sin37∘=35,sin53∘=45
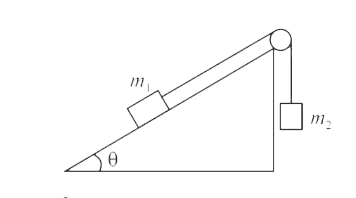
In case I, both blocks are in equilibrium
In case I, friction on is 3 N, acting down the plane
In case II, both blocks are in equilibrium
In case II, friction on m1 is 3.6 N, acting up the plane
The correct Answer is:abc
Assuming equilibrium T=m2g
Taking friction up the plane , T+f=m1gsinθ and N=mgcosθ
put f=μsN to get minimum allowed value of m2m2≥m1sinθ−μm1cosθ
reverser direction of friction to get maximum allowed value of m2m2≤m1sinθμm1cosθ
→ again m2 is in the right range → static friction acts as m2<m1sinθ friction acts up the plane
→T+F=m1gsinθ→T+F=m1gsinθ−T=m1gsinθ−m2g=24−20=4N
Topper's Solved these Questions
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Main ( ARCHIVE ) ( LEVEL-1)
12 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Advanced ( ARCHIVE LEVEL-2)
12 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - level 1
75 Videos
- GRAVITATIONBOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE
1 Videos
- JEE MAIN - 5BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN - 5EXERCISE - PART I : PHYSICS (SECTION - 2)
5 Videos
Similar Questions
In fig. both pulleys and the string are massless and all the surfaces are frictionless.
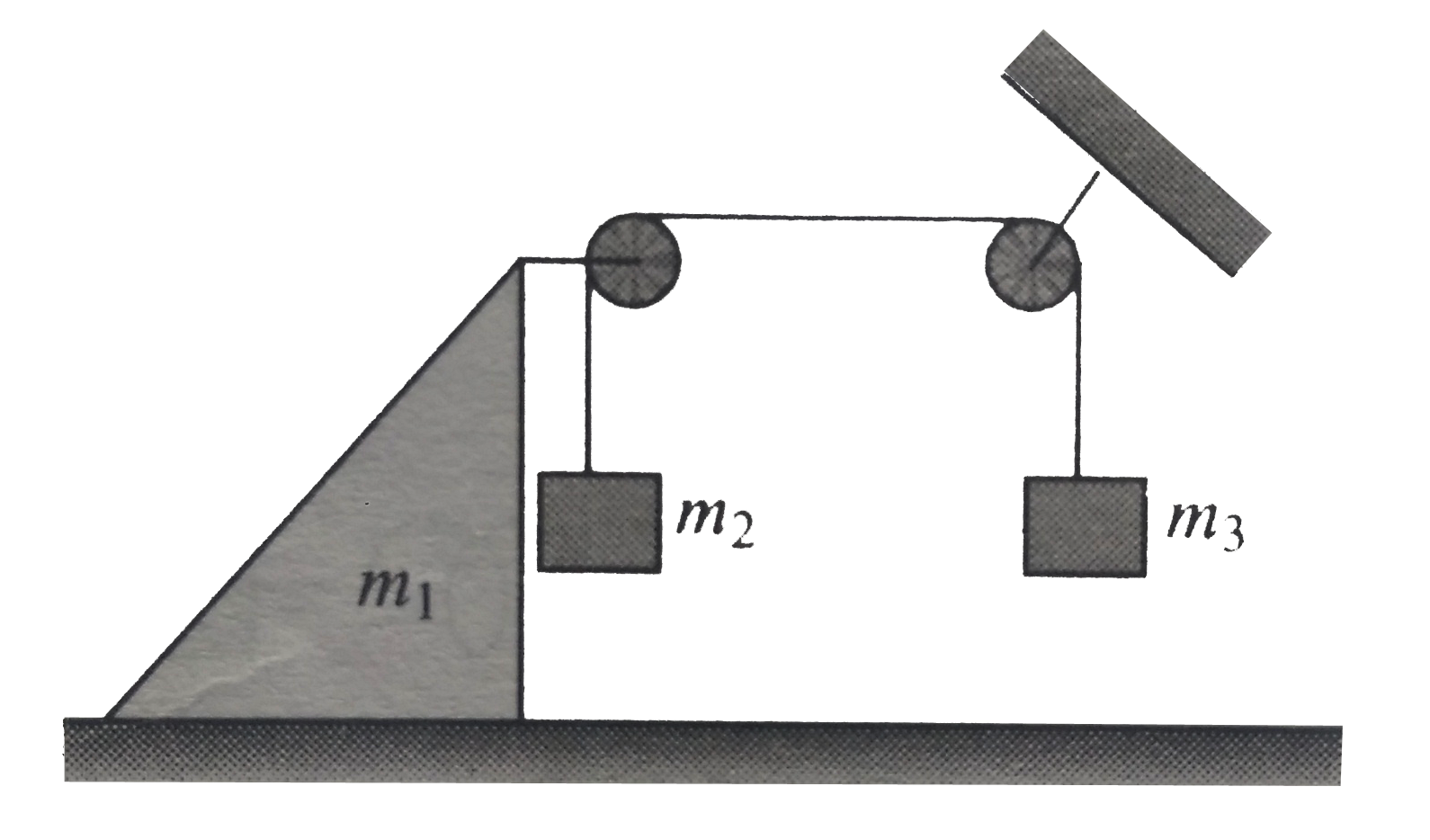
Given m1=1kg,m2=2kg,m3=3kg.
Find the tension in the string
View SolutionIn the figure shown, if friction coefficient of block 1 kg and 2kg with inclined plane is μ1=0.5 and μ2=0.4 respectively, then
View SolutionIn fig. both pulleys and the string are massless and all the surfaces are frictionless.
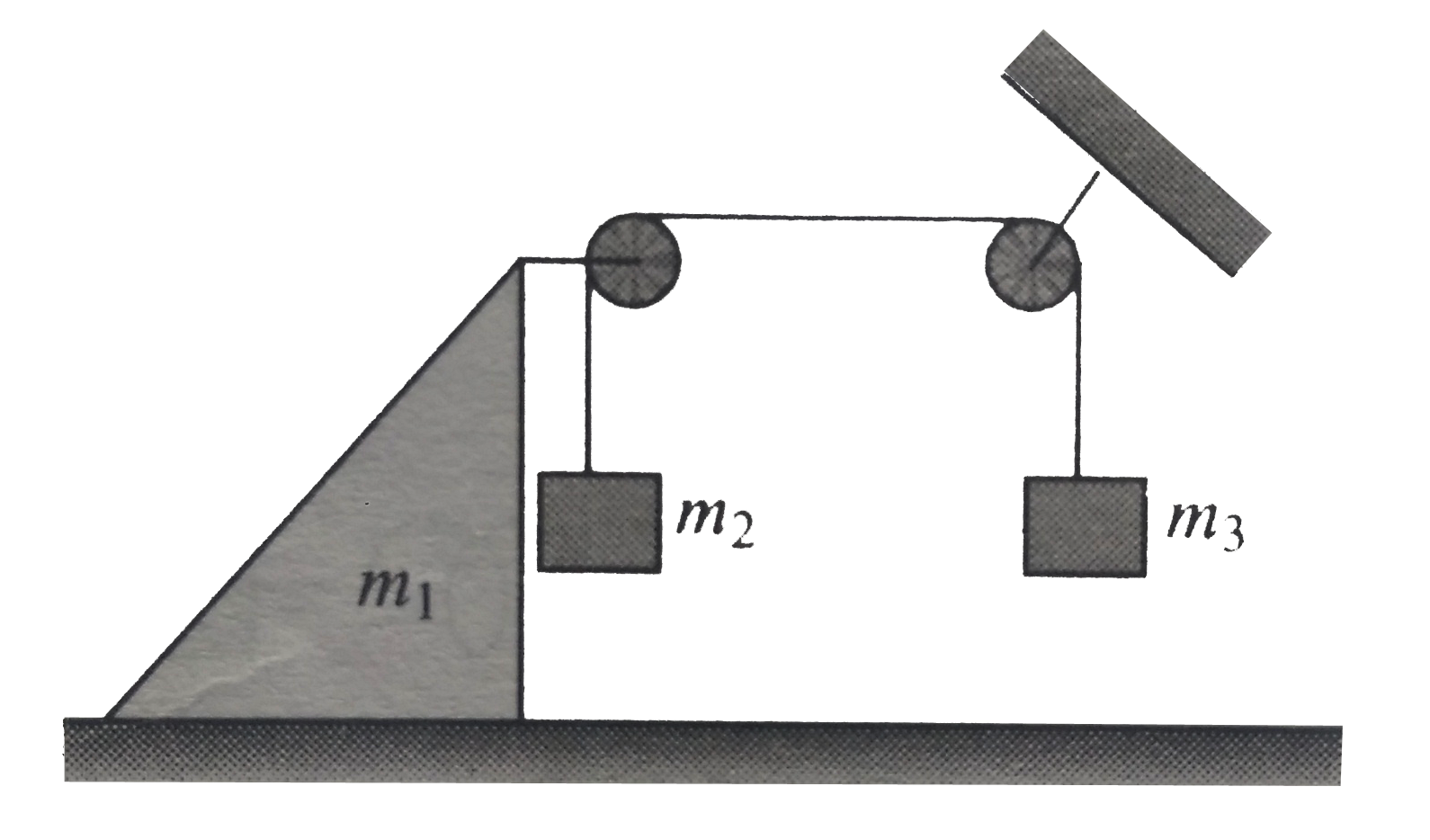
Given m1=1kg,m2=2kg,m3=3kg.
The acceleration of m1 is
View SolutionIn fig. both pulleys and the string are massless and all the surfaces are frictionless.
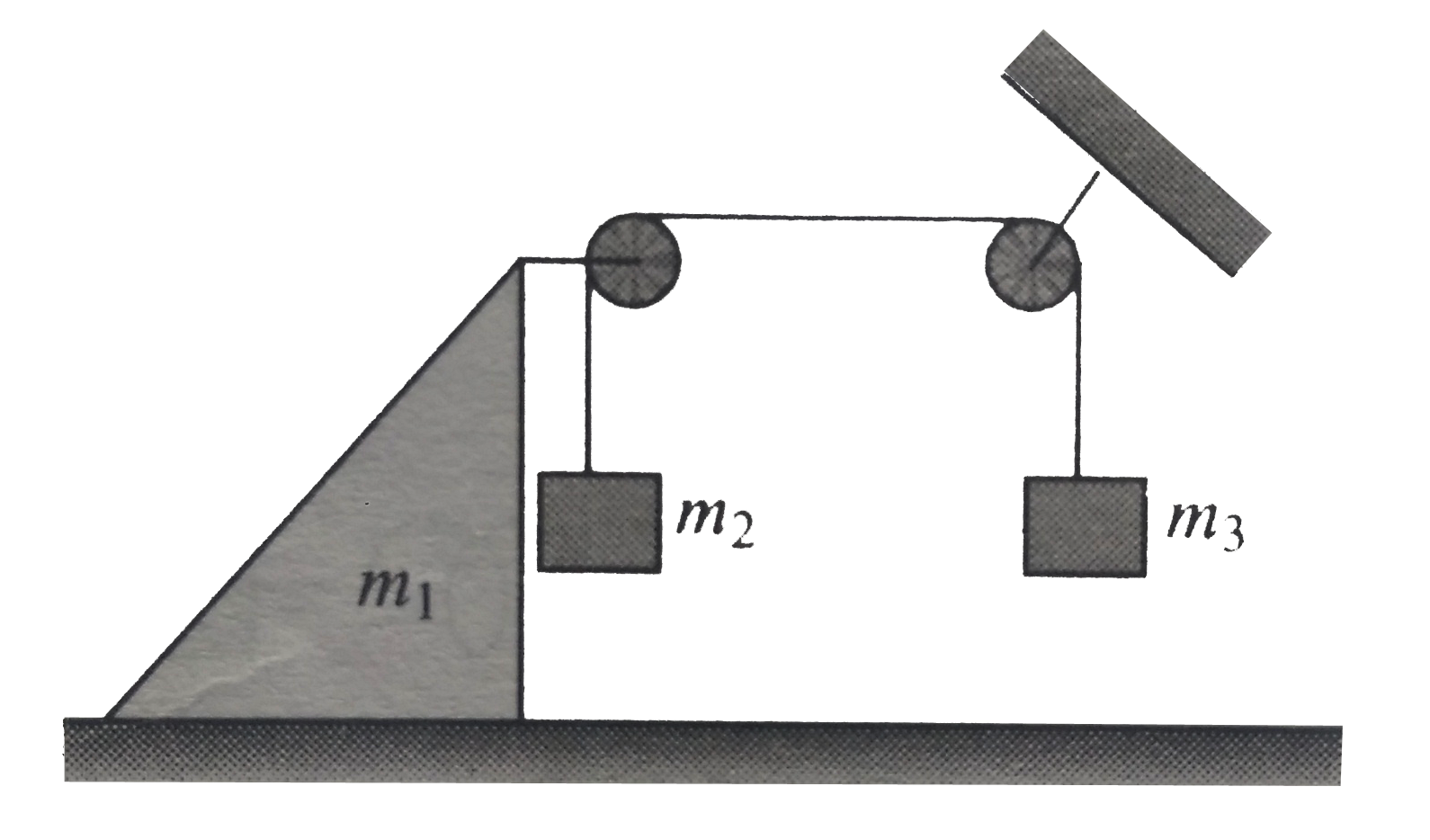
Given m1=1kg,m2=2kg,m3=3kg.
The acceleration of m3 is
View SolutionThe block of mass m1 is placed on a wedge of an angle θ, as shown. The block is moving over the inclined surface of the wedge. Friction coefficient between the block and the wedge is μ1, whereas it is μ2 between the wedge and the horizontal surface. if μ1=12,θ=45∘,m1=4kg,m2=5kg and g=10m/s2, find minimum value of μ2 so that the wedge remains stationary on the surface. express your answer in multiple of 10−3
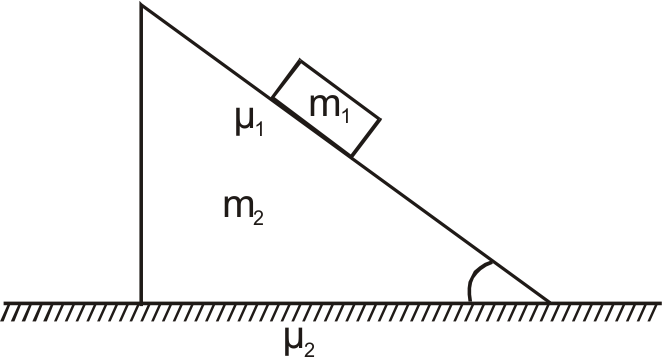
View SolutionThe block of mass m1 is placed on a wedge of an angle θ, as shown. The block is moving over the inclined surface of the wedge. Friction coefficient between the block and the wedge is μ1, whereas it is μ2 between the wedge and the horizontal surface. if μ1=12,θ=45∘,m1=4kg,m2=5kg and g=10m/s2, find minimum value of μ2 so that the wedge remains stationary on the surface. express your answer in multiple of 10−3

View SolutionTwo masses m1 and m2 which are connected with a light string, are placed over a frictionless pulley. This set up is placed over a weighing machine, as shown. Three combination of mass m1 and m2 are used, in first case m1=6kg and m2=2kg, in seconds case m1=5kg and m2=3kg and in third case m1=4kg and m2=4kg. masses are held stationary initially and then released. if the reading of the weighing machine after the release in three cases are W1,W2 and W3 respectively then:
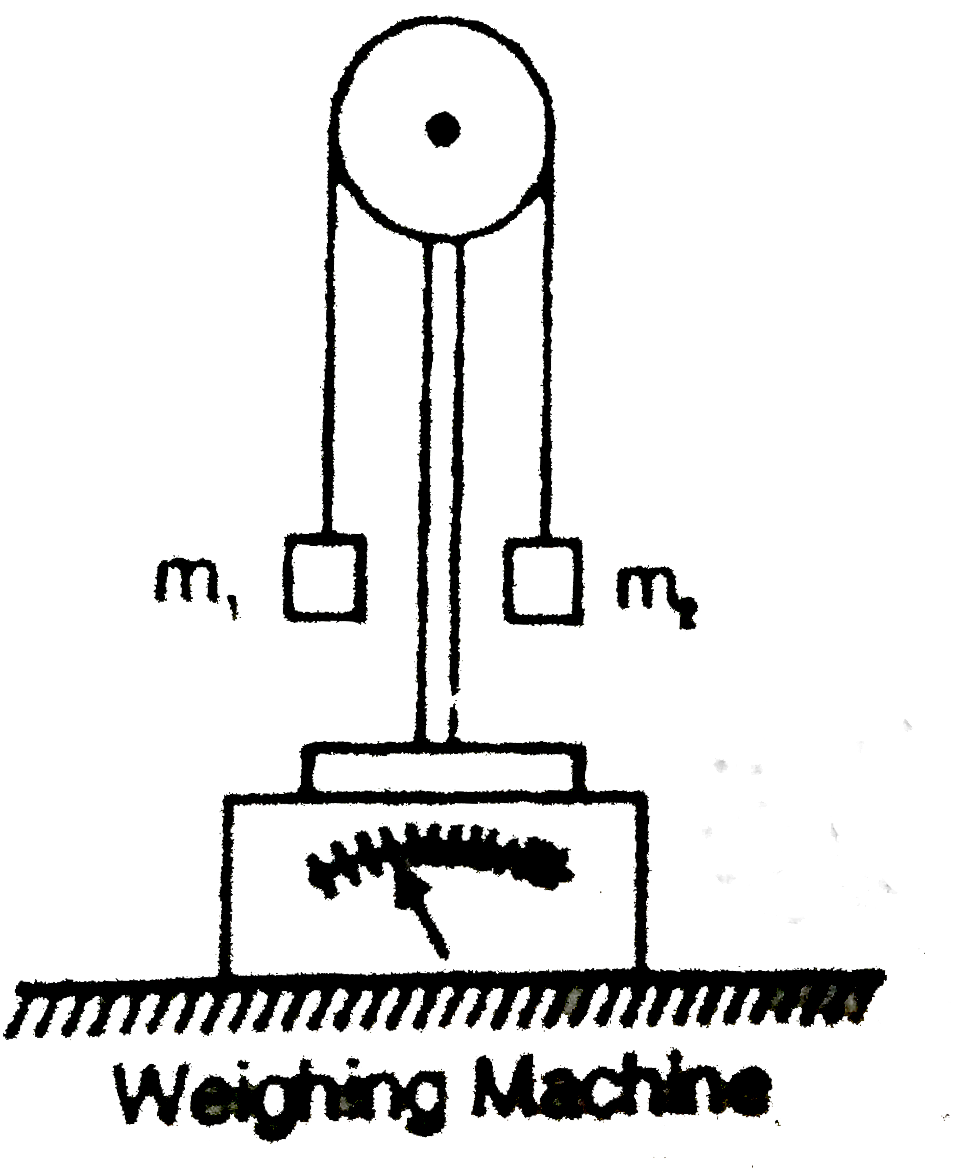
View SolutionIn the figure shown, m1 = 10kg, m2 = 6kg, m3 = 4kg. If T3 = 40 N, T2 = ?
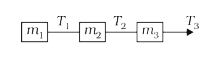
View SolutionFor the given figure the acceleration of 1 kg block if string is massless and mass of pulley is 2 kg and diameter of pulley is 0.2 m :-
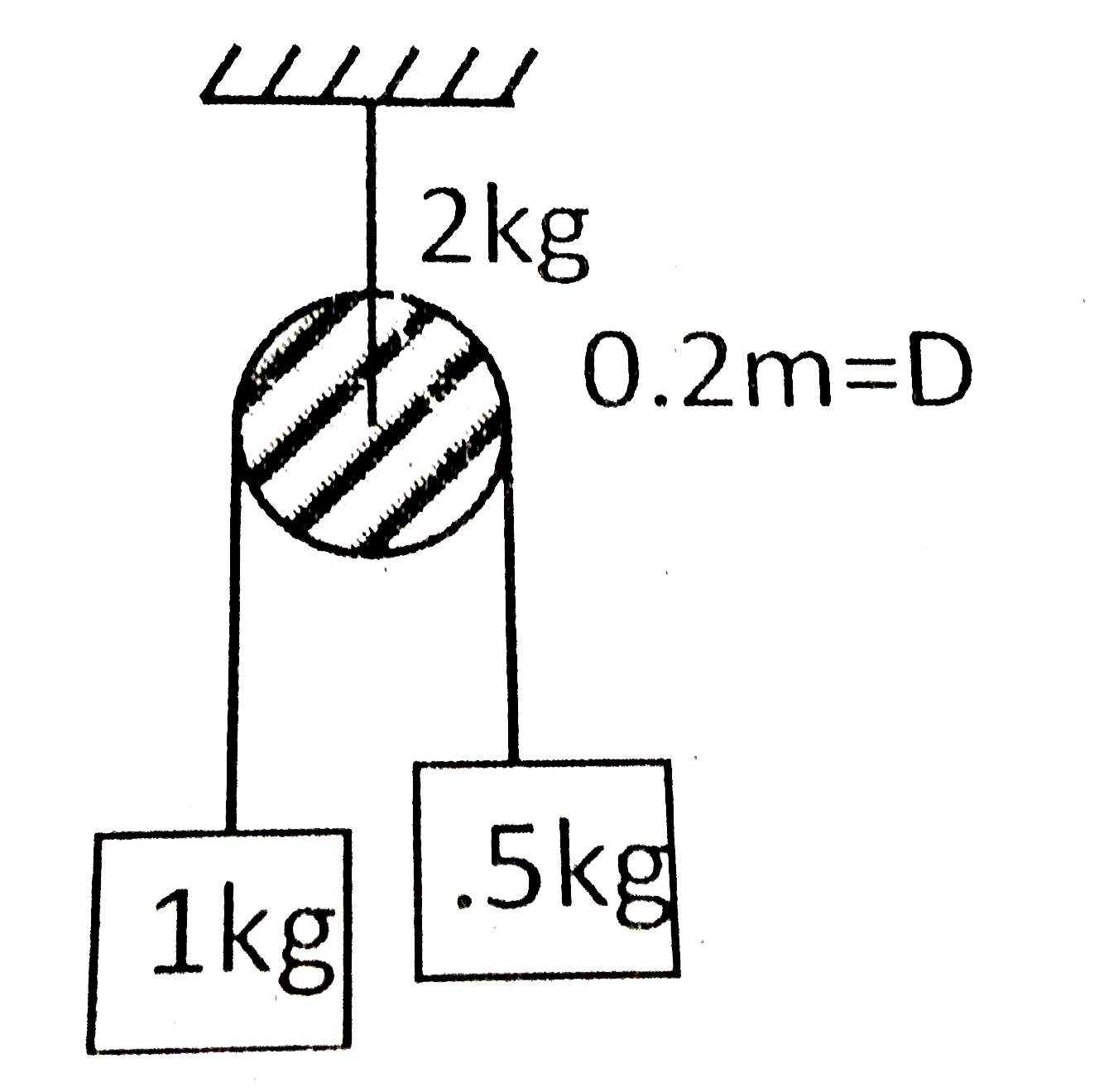
View SolutionTwo blocks of masses m1 = 4 kg and m2 = 2kg connected by a massless rod slide down on an inclined plane of inclination 37o as shown in figure. If coefficient of friction of m1 and m2 with inclined plane are μ1 = 0.75 and μ2 = 0.25 respectively, then tension (T) in the rod is (g = 10 ms−2)
View Solution
